0
$\begingroup$

The work done is the product of force acting on a body and the displacement of the body in the direction of the force. The gravitational potential $\text{energy}=Mgh$ is the negative of the work done by gravitational force. But actually, it is the person who applies force against gravity and does work, right? I am confused with the concept of work done, and it's relation with energy.

Improve this question
$\endgroup$
1

4 Answers 4

Reset to default
0
$\begingroup$

If you move an object from a height $h=h_1$ to a height $h=h_2$ then the difference in gravitational potential energy is

$\Delta \text {PE} = \text{PE}_{end} - \text{PE}_{start} = mgh_2 - mgh_1 = mg \Delta h$

Note that $\Delta h$ is in the *opposite * direction to the force of graivity on the object. $\Delta \text{PE}$ is positive when $\Delta h$ is positive i.e. when $h_2>h_1$. So $\Delta \text{PE}$ represents the work done by the object (or by whatever is moving the object) against the force of gravity $mg$. If the change in PE is negative (i.e. $h_2 < h_1$) then its absolute value $|\Delta \text{PE}|$ is the work done by gravity on the object.

Improve this answer
$\endgroup$
0
$\begingroup$

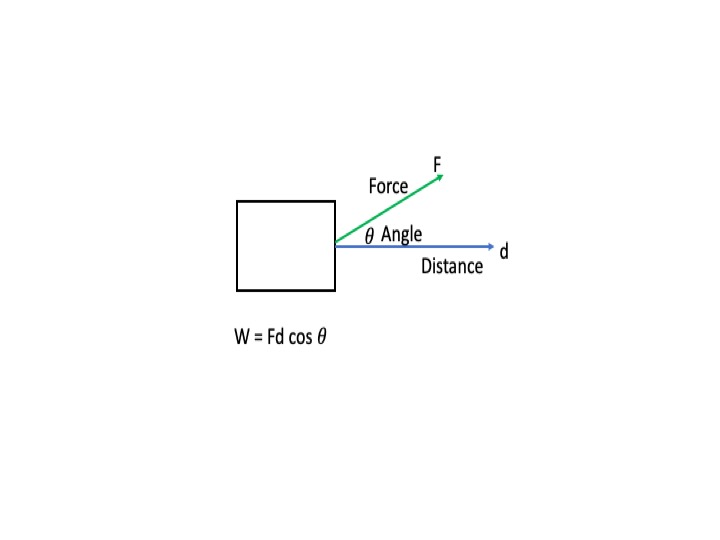
For a constant force, the work done on an object is the product of the force, distance moved, and the cosine of the angle between them. See the figure below.

If the angle is zero, the cosine is 1 and the work done is positive. If the force is in the opposite direction of the displacement, the angle is 180$^o$ and the cosine -1, making the work done on the object negative. If the work done on an object is positive, then the agent performing the work transfers energy to the object. If the work done is negative, then the agent performing the negative work takes energy away from the object.

If you, an external agent, lift an object of mass $m$ a height $h$ you do positive work of $mgh$ if the object begins and ends at rest. It is positive because the force you apply is in the same direction as the distance $h$ moved. It is $mgh$ because there is no change in kinetic energy of the object.

At the same time, however, gravity performs an equal amount of negative work of $-mgh$, because the force of gravity is opposite to the distance moved. Gravity takes away the energy you transferred to the object. The net work done on the object is zero. According to the work energy theorem, the net work done on an object equals its change in kinetic energy. Since the object began and ended at rest, the change in kinetic energy of the object is zero.

So where does that energy you gave the object go?

Gravity has stored it as gravitational potential energy of the earth/object system. It is important to realize than an object alone does not possess gravitational potential energy, though it is commonly stated that way. Every form of potential energy, including gravity, is a system property. That's because potential energy is the energy of the position. In this case it is the position of the object relative to the surface of the earth. That makes gravitational potential energy a property of the combination of the object and the earth.

Hope this helps.

enter image description here

Improve this answer
$\endgroup$
0
$\begingroup$

The total work done by all the forces is equal to the change in the kinetic energy (KE). The work done by a particular force contributes part of the total work, but total work considers all the forces.

The total work is $\int_{a}^{b}\vec F_{net}\cdot d \vec r$ where $\vec F_{net}$ is the total force. We can consider the contribution to work done by a single force $\vec F$ as $\int_{a}^{b}\vec F\cdot d \vec r$. Since gravity is a conservative force, we can express the portion of total work done by gravity as $\int_{a}^{b}\vec F_{grav}\cdot d \vec r = - mg(h_{final} - h_{initial})$, the negative of the change in potential energy (PE). Regardless of the complexity of the path taken, the work done by gravity is easily evaluated as the negative of the change in PE. If other forces besides gravity act, they must also be considered in evaluating the total work.

Consider two simple cases.

Consider a ball of mass $m$ thrown upwards with an initial velocity $v$. After the ball is thrown, the only force acting on the ball is gravity (neglecting air resistance). At maximum height $h$ the force of gravity has does work on the mass equal to $-Fh = -mgh$; negative since the force is down and the displacement $h$ is up. The change in KE, final minus initial, is $ 0 - {1 \over 2} m v^2$. Equating the total work done to the change in KE, $-mgh =-{1 \over 2} m v^2$.

Now consider a ball lifted very slowly upwards to a final height $h$ by a person applying a constant force $F_a$ upwards, just very slightly greater in magnitude than the force of gravity. The net force upwards is $F_a -mg$, the total work done by all the forces is $(F_a -mg)h$, and the change in kinetic energy is zero. So $(F_a -mg)h = 0$. The work done by $F_a$ is positive, $F_ah$, and the work done by gravity is negative, $-mgh$, but the total work is zero.

Improve this answer
$\endgroup$
0
$\begingroup$

Both the person and gravity apply a force to the lifted box. So both do work on it.

  • Gravity does negative work on it while it is being lifted, because its force is downwards (against the motion)
  • The lifting person does positive work on it while it is being lifted, because his force is up along with the motion.

These two works are equal in magnitude but opposite in sign.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.