Yes the phase argument is a bit too simple.
For starters, it would not explain anyons n 2D. Secondly, why should $\Psi(1,2)$ be exactly equal to $e^{2i\delta}\Psi(1,2)$ and not just proportional to it? Quantum mechanics is defined over a projective Hilbert space, so any phase factor would not affect the physical observable anyway.
The first thing you need to realise is that the permutation of the particles' labels is a continuous operation over a parameter space, which cannot reliably be captured by an instantaneous operator that just slaps on a phase factor:
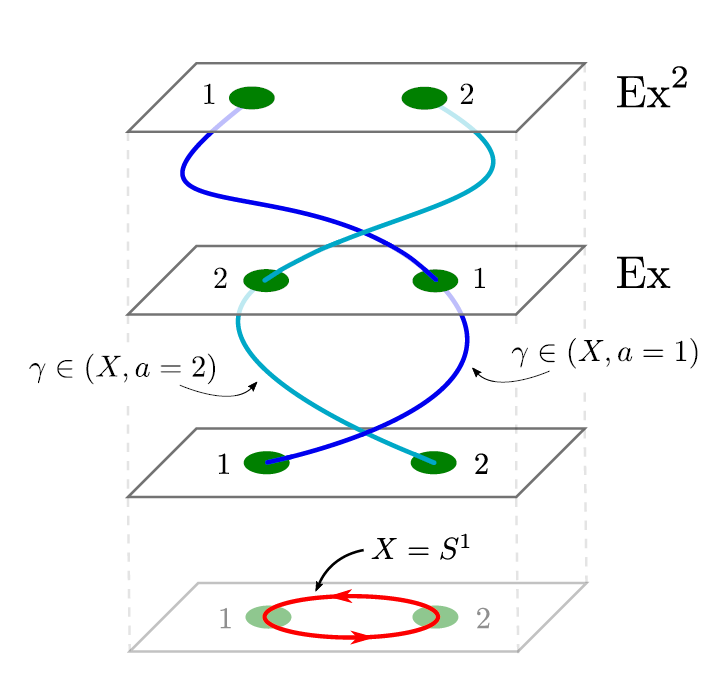
If the particles return to the same original position, they trace out a closed loop $\gamma$ (about a point $a$) in this topological space $X$. The description of loops requires basic topology -- in particular, we can use the fundamental homotopy group $\pi_1$ which comprises all topologically distinct loops, that is the different ways one can permute the particles labels.
The smooth and continuous label exchange operation being a proper rotation in $d$ dimensions, it is represented by $SO(d)$.
So you just need to know the fundamental homotopy group of $SO(d)$:
- $\pi_1$ of $SO(2) = \mathbb{Z}$,
- $\pi_1$ of $SO(3) = \mathbb{Z}_2.$
So, exchanging the particles means tracing out a trajectory in parameter space. A phase is picked up:
$$ \Psi(1,2) \rightarrow \Psi(1,2) = e^{i\theta} \Psi(1,2),$$
which can be acquired in $\pi_1$ different ways:
- $2\pi / \mathbb{Z}_2$ in $d = 3$ (and $d>3$ for that matter), which means that the phase can be $2\pi / {1,2}$ that is $2\pi$ or $\pi$. Bosons and fermions;
- $2\pi / \mathbb{Z}$ in $d = 2$, hence anyons.
Addition after comment.
2D
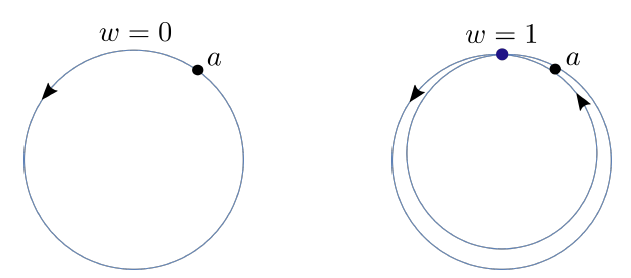
An easy way to visualise why, in 2D, you have a countably infinite way of permuting the particles. Say you want to go around $a$ (e.g. one particles is at $a$, and the other one is being rotated). You are in 2D so confined to a plane. You cannot avoid $a$. So the only way to go "round" $a$ is to cross it. The number of times you cross $a$, the winding number, is what distinguishes that path:
3D
For 3D it's similar albeit slightly more complicated.
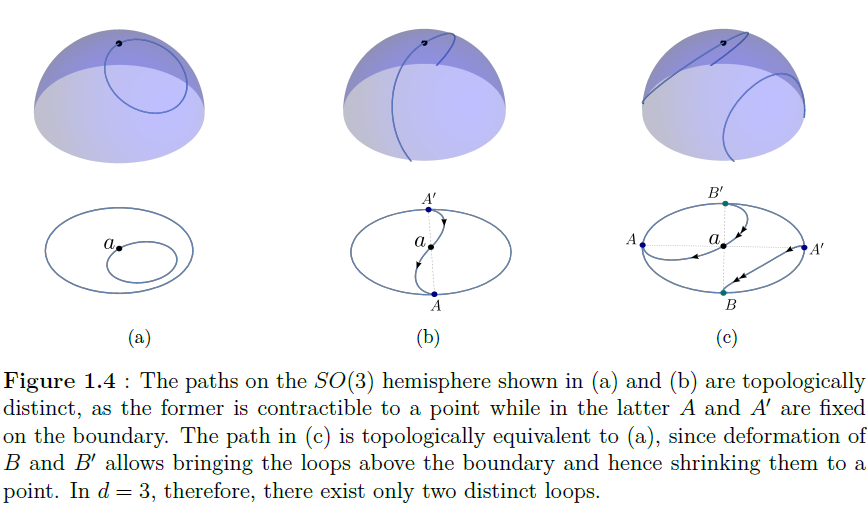
Let's say that a rotation of $\theta$ around $\mathbf{n}$ is identical to one of $\pi-\theta$ about $-\mathbf{n}$ so we can consider a hemisphere (with opposite ends identified). What distinguishes the path now is whether or not they can be shrunk down to a point: