This question (the 1st part), is fraught with difficulties.
It's true the electric field of a moving proton is not a $1/r^2$ Coulomb field. For a velocity $\vec v = v\hat x$, one finds (https://www.feynmanlectures.caltech.edu/II_26.html):
$$ E_x = \frac{+e}{4\pi\epsilon_0\sqrt{1-v^2}}
\frac{-v^2(x-vt)/(1-v^2)}{\big[\frac{(x-vt)^2}{1-v^2}-y^2+z^2 \big]^{\frac 3 2}}$$
while the field in any transverse direction follows:
$$E_y = \frac{+e}{4\pi\epsilon_0\sqrt{1-v^2}}
\frac{y}{\big[\frac{(x-vt)^2}{1-v^2}-y^2+z^2 \big]^{\frac 3 2}} $$
Moreover, there is a magnetic field associated with the current, given by:
$$ \vec B = \vec v \times \vec E$$
The electric field looks like this:
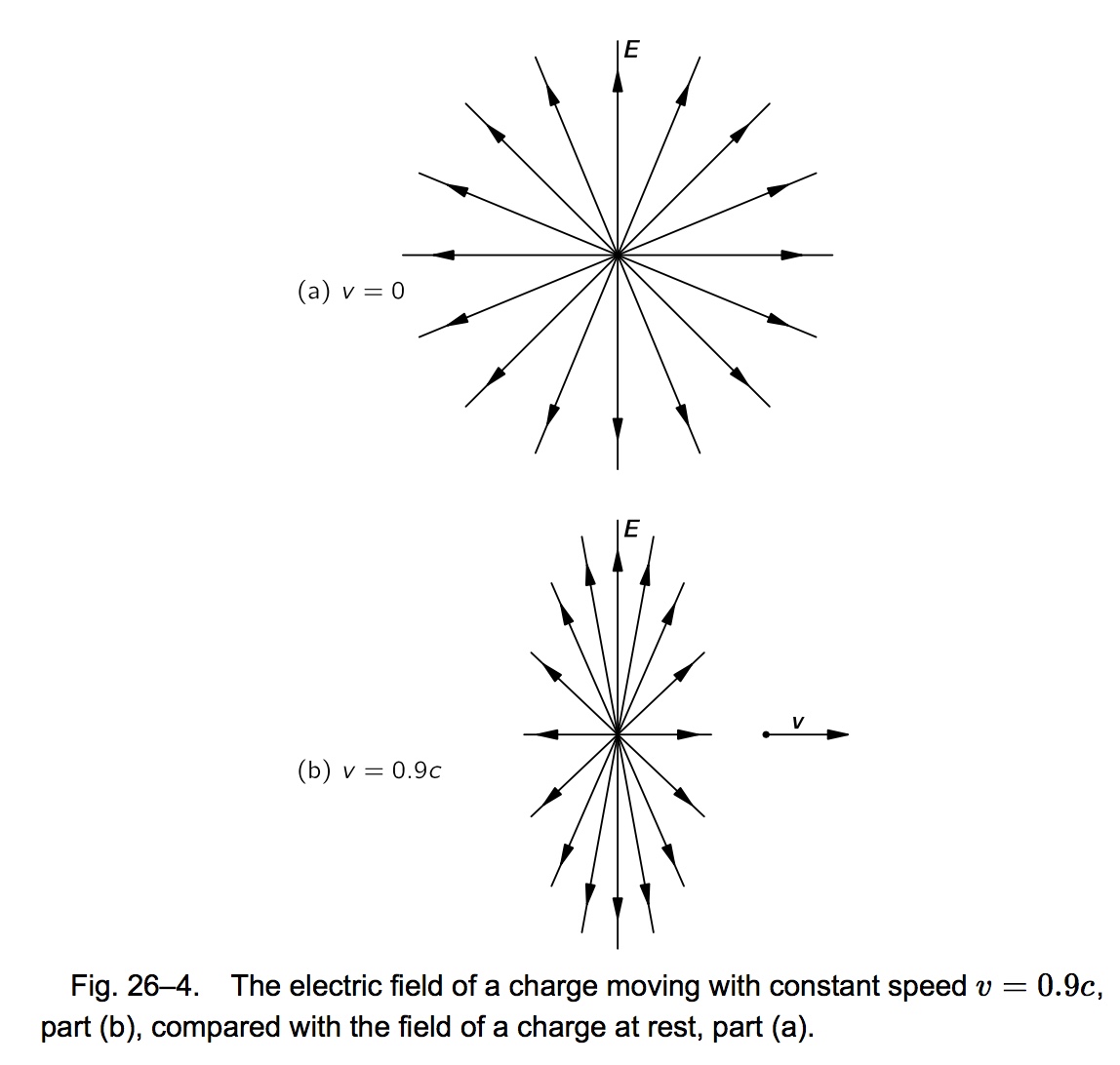
Given that the proton is much more massive then the electron, one could assume this field, and try to find analytic bound solutions for an fiducial charge $-e$ with mass $m_e$, using Schrödinger's equation, with a time dependent potential (is that even a thing?).
That's not exactly fair, as the proton is a degree of freedom in its own right, so you might write down the two-particle hamiltonian:
$$ H =\frac{\hat p_p^2}{2M_p} + \frac{\hat p_e^2}{2m_e} -\frac{e^2}{4\pi\epsilon_0|\vec r_p - \vec r_e|} $$
and look for bound solutions with:
$$ \frac{\langle\psi|\hat p_p|\psi\rangle}{M_p} = \frac{\langle\psi|\hat p_e|\psi\rangle}{m_e} = \vec v$$
which sounds difficult.
You might factor out the center-of-mass motion:
$$ \vec R = \frac{M_p\vec r_p + m_e \vec r_e}{M_p+m_e}$$
setting $$\frac{d\vec R}{dt} = \vec v$$
and solve in terms of:
$$ \vec r \equiv \vec r_e-\vec r_p$$
using the reduced mass:
$$ \mu = \frac 1{\frac 1 {M_p}+\frac 1 {m_e}}$$
which is equivalent to solving it in the rest frame. (Note the Schrödinger's equation is non-relativistic, so this doesn't really work, and you'd just be Lorentz boosting normal solutions anyway).
Since none of those are both satisfactory and tractable, one must adopt a simpler approach:
The mass of a hydrogen atom is:
$$ M_H = M_p + M_e - hc\frac{R_{\infty}}{1 +\frac{m_e}{M_P}} \approx M_p + M_e + R_E $$
($R_E = \frac 1 2 m_ec^2\alpha^2 \approx 13.6\,$eV is the Bohr energy).
Thus, if a hydrogen atom is moving relativistically at velocity $v$, its total energy is:
$$ E_T = \gamma M_H = \gamma M_P + \gamma m_e + \gamma R_E $$
with $\gamma = (1-v^2/c^2)^{-\frac 1 2}$.
The energy of the unbound constituents is:
$$ E_C = \gamma M_P + \gamma m_e $$
The difference is the binding energy:
$$ B.E. = E_T-E_C = \gamma R_E$$
which is greater than the "at-rest" binding energy of $R_E$.
Note, though, that it is not the lowest energy of the system. If we consider just the electron degree of freedom, that occurs for an unbound state in which the electron has velocity $-\vec v$. The total energy is then:
$$ E_{min} = \gamma M_P + m_e $$
If we consider adding the proton DoF, then it too would be moving at $-\vec v$, perhaps bound to the electron...which would be a hydrogen atom at rest.
So: yes, the binding energy is larger, but we expected that, as energy is not a Lorentz scalar. It really doesn't mean a thing.
We can't solve the moving hydrogen atom analytically, but it looks exactly like a Lorentz boosted spherically symmetric ground state hydrogen atom, which undergoes Lorentz contraction into an oblate spheroid.
There is one caveat though, if we could solve the moving hydrogen atom analytically, it would not be Lorentz contracted per se: it would just be squished flat, as that is the solution in our untransformed frame. If we then transformed that into the rest frame of the proton, we would find that it is Lorentz dilated (a lá Bell's Spaceship Paradox) into a spherically symmetric wave function.

