4
$\begingroup$

So I have taken an introductory level quantum physics and am currently taking an introductory level probability class. Then this simple scenario came up:

Given a fair coin that has been tossed 100 times, each time landing heads, would it be more likely that that the next coin flip be tails or heads?

I can see that since the event is independent by definition, then the probability would be even for both heads and tails:

$$P(h | 100 h) = P(t | 100 h)$$

But would this differ in a quantum mechanical standpoint? I have a feeling that $P(h | 100 h) < P(t | 100 h)$ because of the push towards equilibrium in the entropy of the system. Am I wrong to think this way?

FOLLOW UP: (turning out to be more of a statistical problem possibly?)

Something the around the "equilibrium only exists in the infinite-time limit" idea is what I'm getting hitched on.

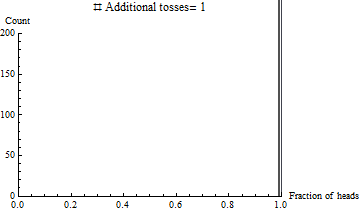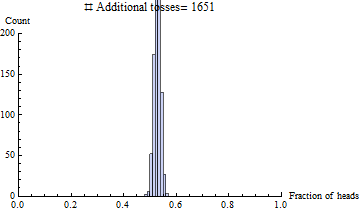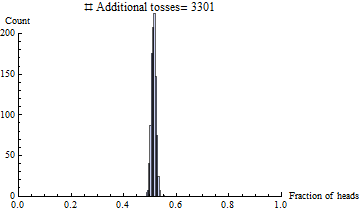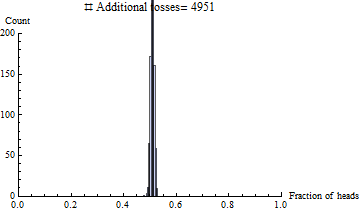
The proportion of head to tails is 1 to 1 as number of trails approach infinity (this is a fact correct?). Therefore, if this must be the case, mustn't there be an enacting "force" per say that causes this state of being to (admitted unreachable, but technically eventual) case? Or is this thought process just illegitimate simple because the state exist on at the infinity case?

Improve this question
$\endgroup$
5
  • $\begingroup$ I don't think entropy has much to do with probabilities of coin tosses. $\endgroup$
    – Michiel
    Commented Apr 18, 2013 at 20:55
  • $\begingroup$ @michielm I think he's just giving that as an example of a time invariant probability distribution. Seems appropriate to me. $\endgroup$
    – rurouniwallace
    Commented Apr 18, 2013 at 21:48
  • 1
    $\begingroup$ Nice question though -- many people don't understand the classical version of the problem; I'd never thought about the quantum version until reading your question. $\endgroup$
    – Douglas B. Staple
    Commented Apr 18, 2013 at 22:19
  • 1
    $\begingroup$ In response to your follow-up: no, there is no force of any generalized kind that ensures a 50/50 heads/tails split. The way it works is that the magnitude of the number of heads minus the number of tails increases with $\sqrt{N}$ with the number of coin-flips $N$. Thus the fraction of coin flips that are e.g. heads, $n_h$, is asymptotic to $n_h \sim 1/( 2\sqrt{N} )+1/2$. So in the limit $N\rightarrow \infty$, we obtain $n_h=1/2$. The proof of this is identical to that of the root-mean-square distance traveled during an $N$-step one-dimensional random walk. $\endgroup$
    – Douglas B. Staple
    Commented Apr 19, 2013 at 0:42
  • 1
    $\begingroup$ BTW, for those still interested, my interpretation is known as the Gambler's Fallacy $\endgroup$
    – SGM1
    Commented Apr 19, 2013 at 1:23

4 Answers 4

Reset to default
5
$\begingroup$

The physics aspect of your question is answered by saying that quantum mechanics doesn't change anything in this situation. The conclusions you get from basic statistics continue to be valid.

Now, as for why that is:

I have a feeling that: $P(h | 100 h) < P(t | 100 h)$ because of the push towards equilibrium in the entropy of the system. Am I wrong to think this way?

Yes, you are wrong to think that way. Here's how that "push towards equilibrium" comes from: let's say you've flipped the coin 100 times and you have gotten 100 heads. That's a very drastic difference between heads and tails. Then you go on to flip the coin a thousand more times. You're most likely to get around 500 more heads and 500 more tails, for a total outcome of 600 heads and 500 tails. That's a much less drastic difference. Even with a 50-50 split between heads and tails, you will be closer to the equilibrium result of 550 heads and 550 tails because the 100-head excess is a smaller fraction of the 1100 total throws.

You can go on to flip a million more coins, and you're most likely to get 500000 of each heads and tails, for a total of 500600 heads and 500500 tails. Here the 100-head excess is barely noticeable relative to the total of 1001100 throws. As you continue to make more and more throws, that 100-head excess gets more and more negligible, even though all additional throws are equally likely to have either outcome.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ +1 But... you'll most likely get $500000 \pm 707$ heads or tails. ;) $\endgroup$
    – Douglas B. Staple
    Commented Apr 19, 2013 at 0:48
  • 1
    $\begingroup$ Well, 500000 is the most probable single outcome. I figured I wouldn't go beyond that, just to keep the argument simple. $\endgroup$
    – David Z
    Commented Apr 19, 2013 at 0:51
  • 1
    $\begingroup$ Yeah, I know, it was mostly a joke. However IMHO the stochastic nature of such problems is one of the most useful things to be learned in undergrad physics. $\endgroup$
    – Douglas B. Staple
    Commented Apr 19, 2013 at 0:53
  • $\begingroup$ Gotcha ;-) and good point. $\endgroup$
    – David Z
    Commented Apr 19, 2013 at 0:54
  • 1
    $\begingroup$ Also, you're a lot more likely to get an answer in the neighbourhood of 500k than you are to get exactly 500k, 'a lot' being an understatement. (Not to clutter up your answer with my comments.) $\endgroup$
    – Douglas B. Staple
    Commented Apr 19, 2013 at 1:10
5
$\begingroup$

As you mention in your question, this is the definition of an independent event. If the coin is indeed fair, then it is irrelevant what has happened in the past in order to determine the probability of future events.

This does not change in quantum mechanics. In fact, QM gives us the first concept of truly random events. As an explicit example of a quantum mechanical coin flip, consider the probability of a spin 1/2 particle being measured to have spin $|{+z}\rangle$, immediately after it was measured to have spin $|{+x}\rangle$: as you know, the probability is exactly 50%.

Regarding any "push towards equilibrium" due to entropy: thermodynamically, the free energy of the system is a property of the state of the system in equilibrium, which by definition has no concept of 'memory', whereas equilibrium only exists in the infinite-time limit. Thus, there can be no thermodynamic forces in response to a series of events that occurred in the past, if those events cannot be inferred from viewing a snapshot of the system in its current state.

The probability of a fair coin toss is always 50%, regardless of how many times 'heads' or 'tails' has been flipped in a row. Otherwise, one would be able to 'charge up' dice, or coins, or decks of cards: Let us roll dice all day, and wait for one of them to roll '1' many times in a row. Then, we'll go play dice for money.

Improve this answer
$\endgroup$
3
$\begingroup$

The other answers are good, I just thought this would be a cute opportunity to learn some animation techniques in Mathematica. I start with a hundred heads and make a number of additional, fair, independent tosses. Then I compute the total fraction of heads and repeat this a thousand times and make a histogram of the results. This makes a single frame of the animation. As the number of additional tosses is increased you'll see the probability distribution for the number of heads shift towards $1/2$ and become more sharply peaked.

Hopefully this embeds okay (its only a 289 kb file): enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ +1 for the awesome animation. (just nitpicking: your Y-axis doesn't rescale the number of counts, if you would make it a fractional count w.r.t the number of filled bins it wouldn't have to) $\endgroup$
    – Michiel
    Commented Apr 19, 2013 at 5:22
0
$\begingroup$

This is a matter of QUALITY and aging of the "fair" coins (long-aged one can be researched for instance in museums), the entropy will grow because of friction, the process of aging, bluring and deleting of signs i.e. tails/heads will continue during very long tossing, the coin has limited life which is usually not mentioned in ideal models. The question is if it is possible to recover the coin from pieces and if yes how count the entropy? About assembling small parts search-for: ribosome, or Maxwell's demon.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.