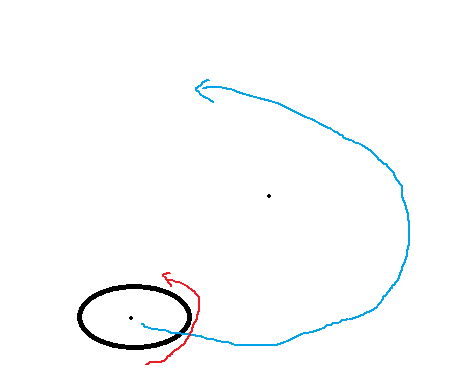
How to calculate moment of ineria of disk if it has double rotation, shown on picture below.
$\begingroup$
$\endgroup$
2
asked Jan 11, 2021 at 8:47
-
$\begingroup$ I guess it depends upon the axis about which u want to calculate $\endgroup$– imposterCommented Jan 11, 2021 at 9:31
-
$\begingroup$ for rotation around blue axis $\endgroup$– Edward Henry BrennerCommented Jan 11, 2021 at 9:34
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
5
This is done using the parallel axis theorem
$$I = I_{cm} + mr^2$$
where $I_{cm}$ is the moment of inertia of the disc and $I$ is the moment of inertia with respect to the athlete, $m$ is a mass of the disc and $r$ is the perpendicular distance between the athlete’s axis and the axis of the disk.
So if you can calculate the moment of inertia of the disc and have the other values above, the rest should be straightforward.
-
$\begingroup$ your answer is not complete what about the rotation of the disc around the centre (red arrow) $\endgroup$ Commented Jan 11, 2021 at 9:42
-
1$\begingroup$ That is what $I$ represents. Did you read my answer? $\endgroup$– joseph hCommented Jan 11, 2021 at 9:56
-
$\begingroup$ yes I did, but it is wrong $\endgroup$ Commented Jan 11, 2021 at 10:10
-
$\begingroup$ You only accounted for the rotatin marked with blue arrow $\endgroup$ Commented Jan 11, 2021 at 10:11
-
1$\begingroup$ There are TWO rotation axes. One for the discus and for the thrower. So please explain how it is wrong. $\endgroup$– joseph hCommented Jan 11, 2021 at 10:22