How do I use first-order perturbation theory to compute the first four energy levels related to a potential well?

Question

I came across a problem whose statement is as follows:

An electron moves in the potential well $P (x) = -\delta$ for $- a <x <0$ and $P (x) = \delta$ for $0 <x <a$ (Fig. 13.7). Use first-order perturbation theory to compute the first four energy levels. Set up the expression for the first-order expansion coefficients for the lowest energy state.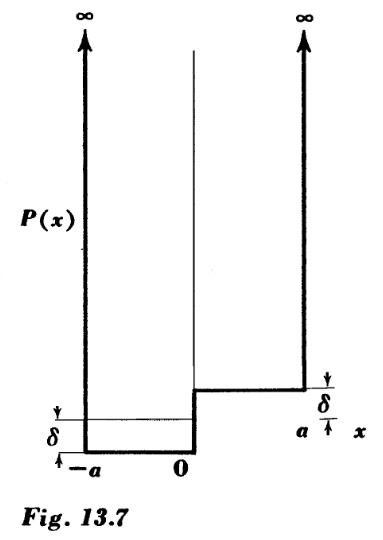

I was trying to use the following equation that I saw throughout the chapter (number 13):

$$ E'_n - E_n = \int_{-\infty}^{\infty} \psi_n^{*} f(x) \psi_n dx $$

Treating $f(x) = P(x)$ and the $\psi_n$ function equal to

$$ \psi_n = \sqrt{\frac{2}{a}} \sin{\left( \frac{n \pi}{a} x\right)} $$

However, this gives me zero as a result of $E'_n - E_n$.

How can I proceed?

The textbook I'm using is Richtmyer F.K.,Kennard E.H.,Cooper J.N. - Introduction to modern physics-McGraw-Hill (1969).

Answer 1

If you have a perturbation $P(x)$, then to 1st order, the energy shifts are:

$$ E^{(1)}_n - E^{(0)}_n = \langle n|P(x)|n\rangle $$

but you have to use the $|n\rangle$ that solve your unperturbed hamiltonian, $H^{(0)}(x)$, not the ones that solve $H^{(0)}(x'=\frac 1 2 (x+a))$.

You can either transform your $\psi_n(x')$ into $\psi(x)$ to match $H(x)$, or you can transform $H(x)$ to $H(x')$ to match you $\psi_n(x')$.

Note that as stated, you have an odd perturbation, so if an unperturbed solution spends 1/2 its time in the left (right) side of the box, the half of the time, the energy is lowered (raised), so you would expect the 1st order perturbations to be zero, esp. for even solutions, since that should be zero by symmetry.

You would expect the 1st order perturbation to the wave function to shift some weight to $x<0$, since the potential is deeper there.