Electric waveguide basic reminders.
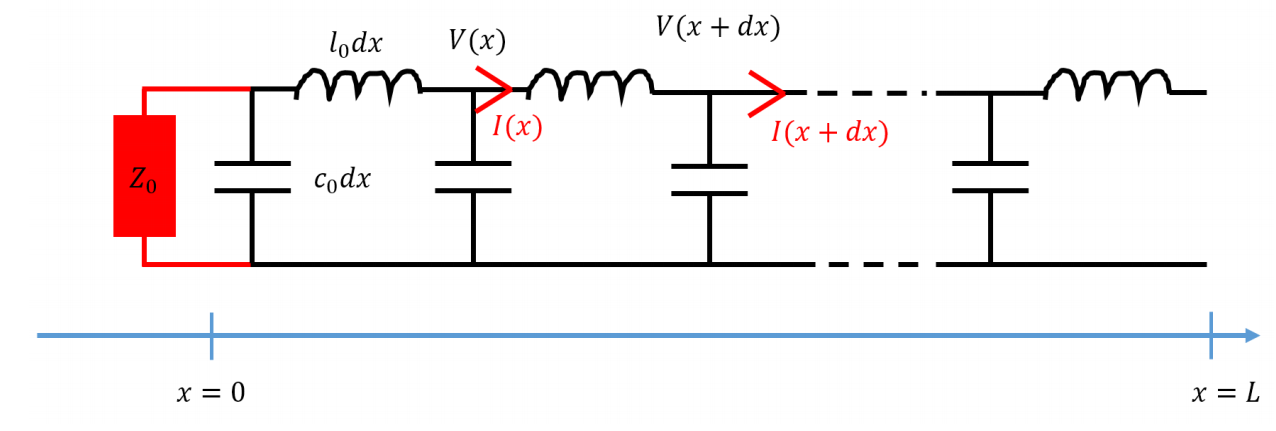
A lossless electric waveguide can be modelled as a succession of capacitance and inductance. Calling $c_0$ and $l_0$ the capacitance and inductance per unit length, we can see the waveguide as what is shown on the Figure above. The mass line is the bottom line (all voltage are defined with respect to it).
I added an impedance $Z_0$ at the end ($x=0$) of the waveguide. The impedance of the waveguide $Z_c$ is:
$$Z_c = \sqrt{l_0/c_0}$$
From this description, we can derive Telegraph equations. They tell us that we will have electric current and potential waves moving on the line. Basically, they imply:
$$ V_{\rightarrow}(x-ct)=Z_c I_{\rightarrow}(x-ct)$$ $$ V_{\leftarrow}(x+ct)=-Z_c I_{\leftarrow}(x+ct)$$
Up to my understanding, the voltage and current are always defined on the top line. Basically $I_{\rightarrow}(x-ct)$ or $I_{\leftarrow}(x+ct)$ represent the electrical current in $x$ at $t$ on the positive (upper) line of my drawing. The voltage are the voltage difference between the positive (upper) line and the mass (bottom) line.
Where $\rightarrow$ are the wave propagating toward $x>0$ ($x<0$ for $\leftarrow)$. The velocity of the waves is $c=\frac{1}{\sqrt{l_0 c_0}}$
The total voltage and current at a given point $(x,t)$ are thus the sum of forward and backward waves:
$$V(x,t)=V_{\rightarrow}(x-ct)+V_{\leftarrow}(x+ct)$$ $$I(x,t)=I_{\rightarrow}(x-ct)+I_{\leftarrow}(x+ct)$$
Now, what I do is to inject a voltage in $x=L$, it will propagate inside the waveguide until reaching $x=0$. Here the impedance $Z_0$ will describe what happens: what is the reflected waves.
We just apply Ohm law in $x=0$, which tell us:
$$V(0,t)=-Z_0 I(0,t)$$
After few calculations, it implies:
$$ \frac{V_{\leftarrow}(0,t)}{V_{\rightarrow}(0,t)}=r=\frac{Z_0-Z_c}{Z_0+Z_c}$$
My question
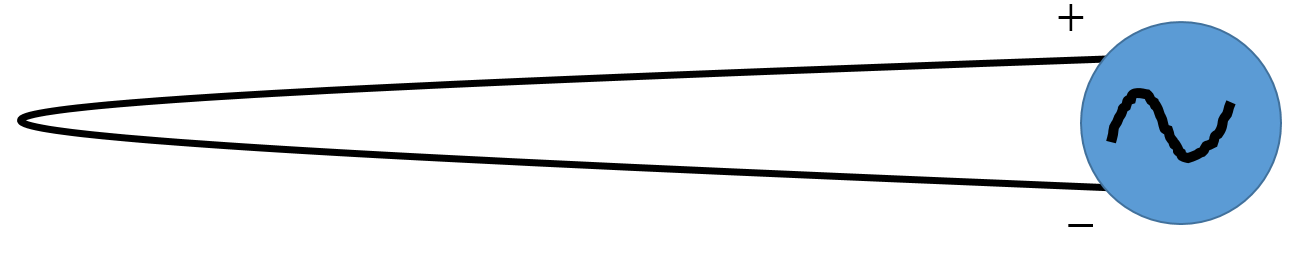
Let's assume I plug to a voltage generator a closed waveguide, like on the following figure (the wire goes out from the $+$ and goes back on the $-$. I assume the length of the wire to be long enough so that we can see propagation phenomenon.
Here the voltage and current I will inject will just follow the waveguide and there will have no reflection. It will go out from the $+$ pole of the generator, propagate, and go back on the $-$ of the generator.
My question is: how can I understand this physics from the general formalism above. Indeed in a way we should say that it corresponds to $Z_0=0$ (because no charge is plugged on the circuit), but this case implies a reflected wave, indeed I will have:
$$V_{\rightarrow}(0,t)=-V_{\leftarrow}(0,t)$$ $$I_{\rightarrow}(0,t)=I_{\leftarrow}(0,t)$$
How to describe the physics of my generator connected to a closed wire in term of the general description given above ?