But is it still true in the context of wave propagation?
Yes, a coaxial cable still has its shielding effect during wave propagation.
If I assume I send a current pulse on the outer shield, will it be exactly instantaneously compensated by an opposite current on the outer shield ? For me the current wave must propagate until the end of the line, then bounces back, and goes back on the inner line. And, after I waited long enough I will indeed have inner current = - outer current. Thus in a regime of varying signals I won't have in principle a zero magnetic field outside.
Am I correct? Am I making a mistake?
Your mistake is assuming that the current must "flow" via the inner conductor to the load first, before it "flows" back via the outer conductor. This is incorrect.
According to transmission line theory, when a coaxial cable (with negligible resistance) is suddenly connected to a voltage source like a battery or a signal generator, a transverse electromagnetic (TEM) wave is launched. The wavefront moves along the cable at the speed of light within the dielectric, typically 0.5c to 0.66c. As the wavefront travels, the electric field is established between inner and outer conductor, and the magnetic field is also established around both conductors at the same time. In other words. As a current flows through the inner conductor, an equal and opposite current also immediately flows through the outer shield. The return current is sometimes also known as the image current, similar to the problems in electrostatics and in monopole antenna that can be analyzed using the method of images.
This conclusion is true for all two-conductor TEM transmission lines, including the simplest case of two parallel wires. It may sound counterintuitive, but it's how nature really works, and it can be readily experimentally demonstrated with a long wire, a fast (5 ns resolution) oscilloscope. One can see that the return current flows immediately without a time delay.
Buildings have walls and halls. People travel in the halls, not the walls. Circuits have traces and spaces. Energy and signals travel in the spaces, not the traces.
- Ralph Morrison, Grounding and Shielding: Circuits and Interference
For two parallel wires in a vacuum, the fields around the wires may look similar to this:
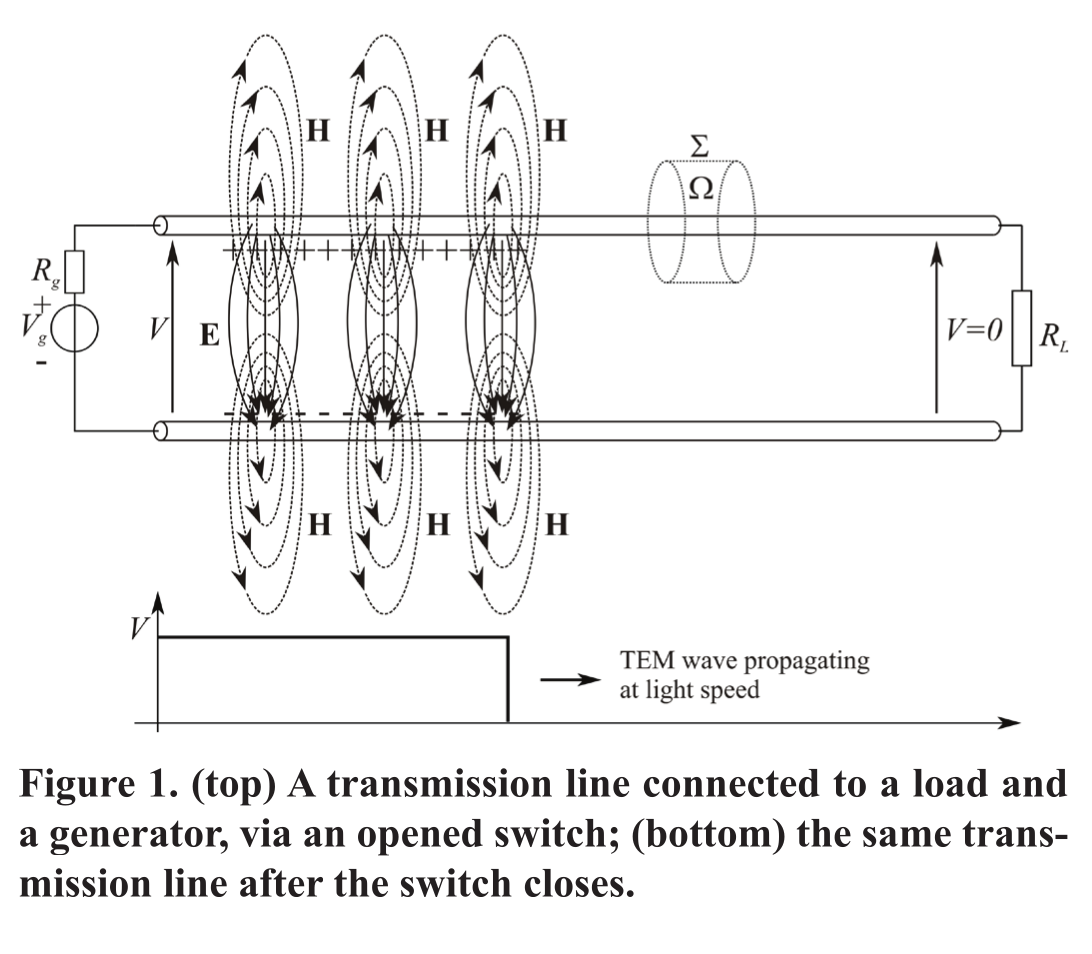
Conveniently, although transmission lines are really electromagnetic waveguides, but the behaviors of TEM waves are particularly well-defined, allowing engineers to ignore the hard physics of electrodynamics, instead modeling the line as a circuit. For an infinitely short section of a lossless line, one can represent the electric field as a voltage across a capacitor, and represent the magnetic field as a current across an inductor.
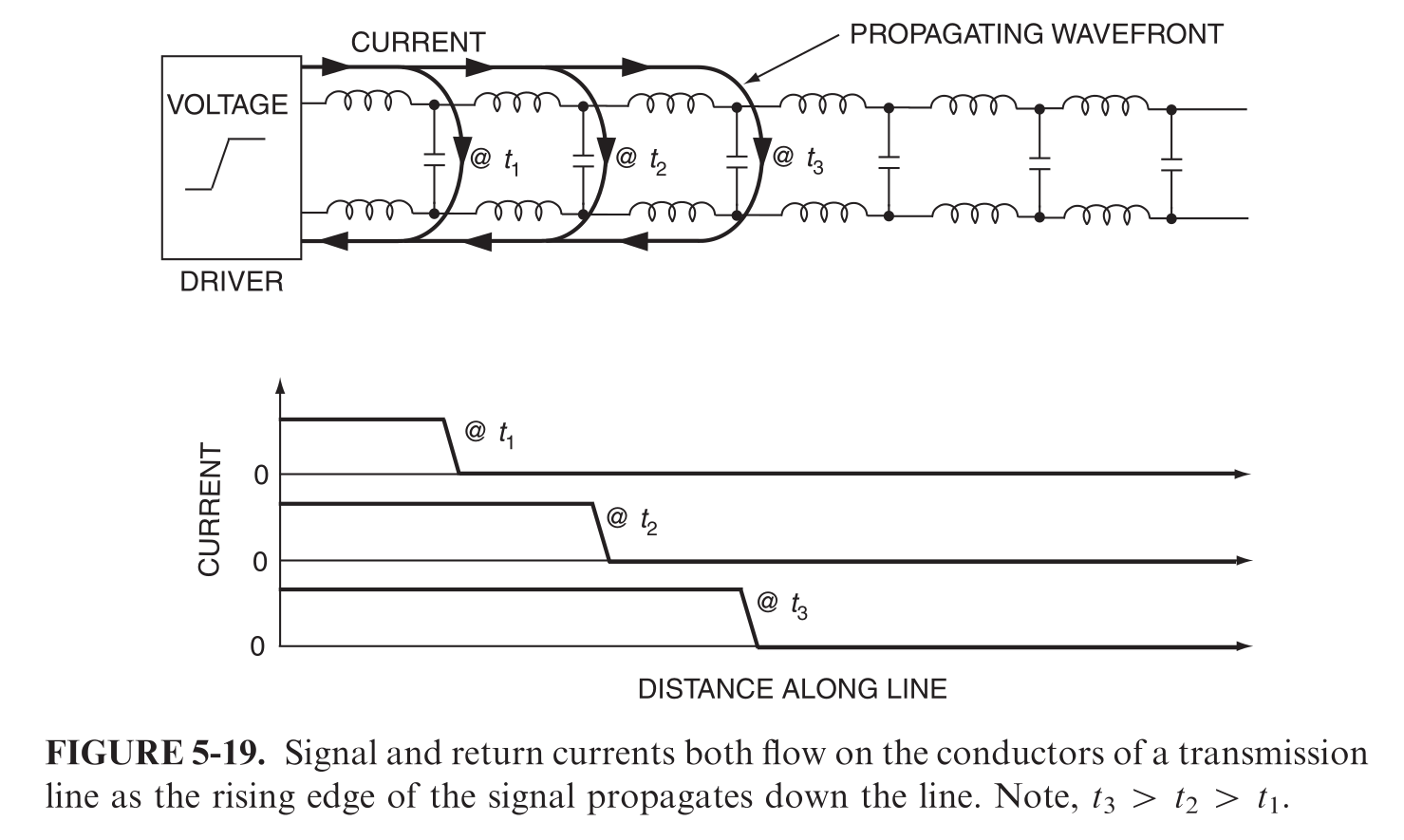
Source: Henry W. Ott - Electromagnetic Compatibility Engineering, Chapter 5.6
The second question I have is: why an outside E.M field cannot enter inside? Is the answer based on the fact the outer surface is metallic and totally reflects any outside E.M wave?
Yes. In a sense, the shielding effect of a coaxial cable requires wave propagation. When a coax cable carries a steady-state DC current or static electric charges, the shielding effect is arguably not entirely true.
What disturbs me is that this fact is true for any metallic wire (thus not only coaxial cable): electric field will never enter in it.
No, metallic conductors are not automatically shielded from electric fields. It's true that electric field lines terminate on the surface of the conductor, but by applying an external electric field to an open wire, it still creates charge movement and redistribution, and can potentially disrupt circuit operation (especially if the external field is time-varying),
The thing we should protect against is outside magnetic field that would induce current. And this is a property of the geometry of the full circuit not of the wire in itself (if my circuit has a square shape, magnetic flux inside of this square can induce currents).
You're right that magnetic fields are often a larger problem in practical applications. Minimizing the loop area is an important circuit design rule to minimize interference, this is usually done by placing signal and return conductors in close proximity to reduce the magnetic flux in the enclosed area.
In fact, even a coax cable can have this problem in practical applications when three conductors (not two) are involved - the coax's inner conductor and coax shield, with the addition of a common ground plane. The effect of a ground plane is often unavoidable and unpredictable, it may be an actual Earth ground or the floor of the room, the mains AC safety ground, or near-field coupling via random conductive objects near the cables.
At the source and load, the shield of the coax is connected to the metal enclosures of both sides, but the metal enclosure themselves is also connected to the common ground plane. As a result, a closed loop, often known as ground loop is formed by the shield of the coax shield and the ground plane.
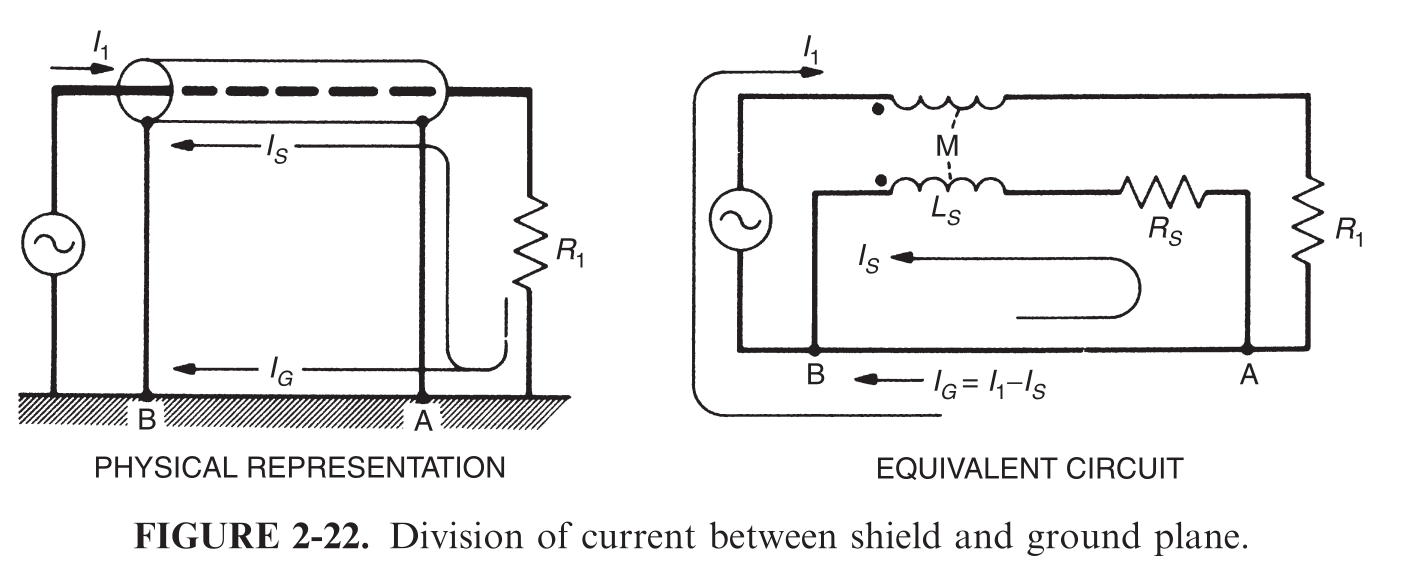Source: Henry W. Ott - Electromagnetic Compatibility Engineering, Chapter 2.5
Now there exists two problems that need elaboration. First, who says the return current must flow on the shield of the coax cable? If the return current flows on the ground plane instead, field cancellation no longer occurs, and the entire circuit radiates via the loop. Next, a changing magnetic field across the area between the coax shield and the ground plane induces a noise current on the shield. The magnetic flux enclosed in the loop can be high, since the area of this "transformer" or "loop antenna" is uncontrolled, and can be extremely large. Thus, one can argue that a coax cable can't practically shield from external magnetic field.
Both sounds like serious problems, but they're rarely discussed. Why? The answer is, coax cables are usually used for radio signals in practice, and at radio frequencies, both effects become negligible. Due to proximity effect, the impedance of the nearby shield is much lower than the impedance of the ground plane, the vast majority of return current flows on the shield (even if the ground plane has a much lower DC resistance). Due to skin effect, coax shield acts as two shields, the return current flows along the interior of the shield, and the noise current flows along the exterior of the shield. Thus, at radio frequencies, a coax cable behaves as if it's a triaxial cable with an additional Faraday cage that encloses its outer surface. When combined with the metal enclosures at the source and load, the entire system is well protected from external interference.
A similar problem exists for the electric field. From Faraday's ice pail experiment, we know that a charged object inside a metal shield induces charges on the exterior of the shield. Thus, one can argue that a coax cable can't stop its internal electric field from leaking out either, unless it's connected to the Earth ground. The solution to this problem is similar: coax cables are usually tied to the Earth ground, and even when they're not, they're meant to shield radio waves, rather than the negligible electric field from electrostatic charges.
