Resistance between metal balls in an infinite, homogeneous, conducting medium

Question

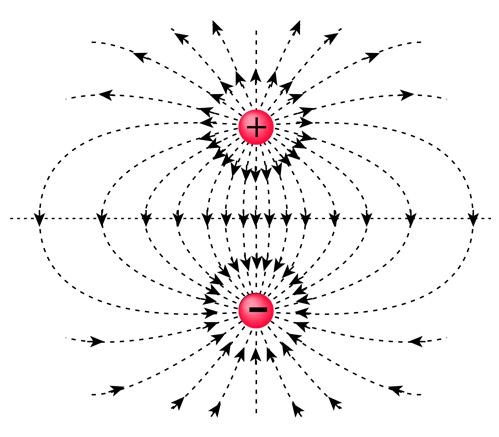
"3.157. Two metal balls of the same radius $a$ are located in a homogeneous poorly conducting medium with resistivity $\rho$. Find the resistance of the medium between the balls provided that the separation between them is much greater than the radius of the ball."

My solution:

I instill charges $+q$ and $-q$ on both the spheres so that I can measure a potential difference,

after that I write

$ J= \frac{E}{\rho}$

and,

$ I = \int J * dA$

Later,

V= IR

and, $ V= (\int J * dA) * R$ and solve for 'R'

Now my question is when integrating over area why is it wrong to take a disc between the spheres? I am taking a circular disc in middle of both spheres to integrate current density over.

However,in the actual solution, they have taken to integrate over the surface of the whole sphere.. why is that? Because like all the current which passes thru one sphere shouldn't always hit the other sphere (I'm thinking of current as beams flowing from the sphere)

Please comment if the question needs more clarification.

---

Related posts :(1) 2

Answer 1

The current flows through other regions as well, so you have to integrate over all regions, instead of just the cylindrical region between the spheres. This image shows the field lines of a large dipole, which is proportional to the local current density vector ($\vec{j} = \sigma \vec{E}$).

As an analogue, suppose the spheres are connected through a straight wire (the cylinder), and additionally, an infinite mesh of long wires (the rest of the space). You'll have to add the inverse resistances of all the wires (not just the straight wire) to get the total resistance. Also, in your derivation, $J$ is not a constant, so you cannot easily integrate this way either.

One way to solve the problem is as follows. Consider a single sphere of given radius $a$ , charged to potential $V$. Now consider a spherical surface at infinite distance, which has potential zero. Now you can calculate the electric field at any point, and from that you can calculate the current density with $\vec{j} = \sigma \vec{E}$. Now you can integrate over any spherical shell to get the net current $I$ (which turns out to be $4 \pi V a / \rho$).

Now suppose the previous arrangement is not there, and consider another sphere, charged to potential $-V$, and follow the above argument. The current will be exactly equal in magnitude but negative.

Now consider a superposition of these two. Since Ohm's law and the laws of electrostatics are linear, the principle of superposition will hold. Now the net result is, a current $I$ is emerging from the sphere at potential $V$ and entering into the negatively charged sphere. The sphere at infinity, kept at zero potential does not matter. You previously calculated the relation between $I$ and $V$. Now you can divide the net potential difference $2 V$ by $I$ to get the resistance, which is $\frac{\rho}{2πa}$.

Image source: http://hyperphysics.phy-astr.gsu.edu/hbase/electric/dipole.html

Answer 2

Now my question is when integrating over area why is it wrong to take a disc between the spheres? I am taking a circular disc in middle of both spheres to integrate current density over.

The simple answer of this mistake is that there is nothing to gaurentee that field would be uniform over such a region.. As seen in Archisman Panigrahi's answer, the sphere's emit current in all direction similar to how field lines go for dipole field.