The following statement explains the main source of error in a constant volume gas thermometer:
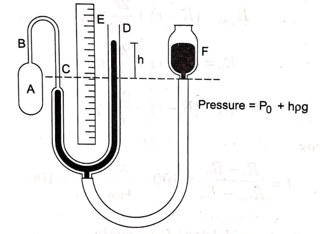
Figure 1 : Constant volume gas thermometer
In constant volume gas thermometer, the gas in the capillary tube [$BC$] connecting the bulb [$A$] and the manometer [$CD$] remains outside the heat bath. The temperature of this part is different from the main bulk of the gas and this introduces some error.
Later, the author says that "Callendar's compensated constant pressure thermometer" avoids this problem by a special design as given in the diagram below:
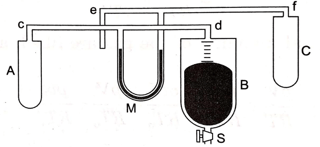
Figure 2 : Callendar's compensated constant pressure thermometer
Here, bulbs $A$ and $C$, and connecting tubes $cd$ and $ef$ are of same volumes. $M$ is the manometer whose arms are connected to $cd$ and $ef$. Bulb $B$ is filled with mercury. And mercury can be taken out through the valve $S$. Both bulbs $A$ and $C$ are filled with the same ideal gas of equal amounts. When all the parts of the thermometer are at the same temperature the level of mercury in the arms on the manometer $M$ remains equal.
The following statement explains the procedure to determine temperature using this type of thermometer:
To measure the temperature of a heat bath (at a temperature larger than the ice point), the bulb $A$ is placed in the heat bath and the bulbs $B$ and $C$ are placed in melting-ice baths.
I also understood the mathematical derivation for the following formula:
$$T=\frac{V}{V-v'}T_0$$
where, $T$ is the temperature of the heat bath, $V$ is the volume of bulbs $A$ and $C$, $v'$ is the volume of mercury taken out of the bulb $B$ and $T_0$ is the temperature of the ice bath.
I don't understand why we must keep the bulb $B$ in the ice bath along with bulb $C$? While doing so, it seems we're again inducing errors due to non constant temperature of the gas sample as it happened in the constant volume gas thermometer. Or in other words, I feel the temperature of the gas in bulb $A$, tube $cd$ and bulb $B$ to vary drastically which will again lead to errors.
So, what is the reason behind keeping the mercury bulb $B$ in ice bath? Why not keep it in some other temperature, say, either room temperature, or the heat bath in which bulb $A$ is placed?
All quotes and images are from the book "Concepts of Physics" by Dr. H.C.Verma, chapter 23, "Heat and Temperature".