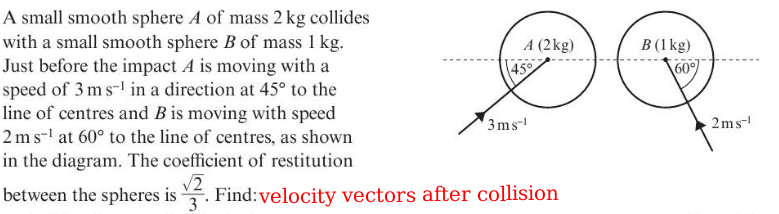
How could I solve this problem using the ZMF concept? I understand how this would be done in a 1D problem, so could I apply the same logic, finding a vector that makes momentum in each direction zero, however, impulse is only applied in the i direction, so it would not be as simple as to multiply the velocity of A in the ZMF by e?. Here is what I have so far $$2\begin{bmatrix} 3\cos(45) \\ 3\sin(45) \end{bmatrix} + \begin{bmatrix} -2\cos(60) \\ 2\sin(60) \end{bmatrix} = \begin{bmatrix} 3x \\ 3y \end{bmatrix} $$ Hence vector of frame, $(x,y)$ $$ \begin{bmatrix} x \\ y \end{bmatrix} = \frac{1}{3} \begin{bmatrix} 3\sqrt2-1 \\ 3\sqrt2+\frac{\sqrt3}{2} \end{bmatrix} $$ The next step I think would be to find the velocities of A and B in the frame, but I cannot think what would be done afterwards.
-
$\begingroup$ What is the ZMF Concept? $\endgroup$– John AlexiouCommented Mar 9, 2020 at 20:22
-
$\begingroup$ @ja72 Zero momentum frame sometimes called COM frame. I worded it like that because I want to know how to use the ZMF in this basic example rather than solving it by an easier alternative method. $\endgroup$– jamieCommented Mar 9, 2020 at 20:26
-
$\begingroup$ So you define a co-moving reference frame that tracks the center of mass of the system. The velocities in this frame are found by subtracting the original velocity with the frame velocity. The result should be two equal and opposite momentum vectors before collision. $\endgroup$– John AlexiouCommented Mar 9, 2020 at 20:37
-
$\begingroup$ @ja72 Right, so I can multiply the x component of the velocities by the cor(since line of centers parallel to i, collision will only affect i), then add back the frame velocity to get final velocities of a,b? How would this work if line of centres was not parallel to i and instead impulse was at an angle? Its sounding like it gets arithmetically complicated, whereas the nice part of 1D ZMF frame was that the calculations were really easy. $\endgroup$– jamieCommented Mar 9, 2020 at 20:42
-
1$\begingroup$ This is the reason we have invented vectors, in order to take care of the complex math needed when done by component. $\endgroup$– John AlexiouCommented Mar 9, 2020 at 20:47
1 Answer
The velocity of the zero momentum frame is
$$ v_C = \frac{ m_A v_A + m_B v_B}{m_A+m_B} $$
Then the momenta in the ZMF are
$$ \begin{aligned} p_A &= m_A\, (v_A-v_C) =\pmatrix{ \tfrac{2}{3}+\sqrt{2} \\ \sqrt{2} - \tfrac{2 \sqrt{3}}{3}} \\ p_B &= m_B\, (v_B-v_C) = \pmatrix{ -\tfrac{2}{3}-\sqrt{2} \\ -\sqrt{2} + \tfrac{2 \sqrt{3}}{3}} \end{aligned} $$
which you can show that $p_A + p_B = 0$
Now add and subtract some impulse $J$ along the contact normal (x-axis) and use the restitution law to find its magnitude.