4
$\begingroup$

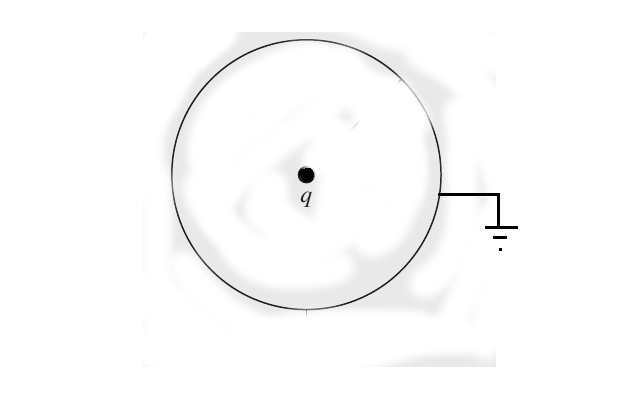
enter image description here

We have a thin, grounded, metallic spherical shell. While solving for its total energy using the formula

$$U_E = \frac{\epsilon_0}{2}\int|\mathbf{E}|^2\,\mathrm d^3r$$

the book I am following says that the electric field outside the shell is zero. But I think Gauss's law doesn't allow that as any spherical Gaussian surface larger than the shell will enclose a net charge of $q$.

If the field is indeed zero outside the shell, then the charge at the center must somehow be cancelled. I don't understand how can the grounding of the shell cancel the charge. Can anyone explain why this happens?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
3
$\begingroup$

As the shell is grounded, charge will move either to or from the ground in order to bring the potential of the shell to zero. Let the shell have net charge $x$ on it. Then the potential of shell is given by-

$$V_{total}=V_{shell}+V_q$$ $$V=\frac {kx}r+\frac {kq}r$$ But since $V=0,\; x=-q$. This means that $-q$ charge will flow from the ground to the shell. Hence if we draw a spherical Gaussian surface at any point outside the shell, the net charge contained inside will be $q+(-q)$ which is zero. Hence net electric field will be zero at all points outside the shell.

Improve this answer
$\endgroup$
0
$\begingroup$

I am suspicious of the answer given above by Sam.

Suppose the radius of the shell is $a$. Then, the expression of the contribution of the shell, $V_{shell}=\frac{kx}{r}$, to the total potential is only true for $r>a$, i.e., outside the shell. Now, the problem says that the shell is grounded, and that means that $V=0$ inside the shell. So we cannot simply set $V=\frac{kx}{r}+\frac{kq}{r}$ to zero.

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ When you say inside the shell, are you referring to the bulk material of the metal, where V = 0, or are you referring to the empty space enclosed by the shell? There is still electric field within the empty space enclosed by the metal shell, per Gauss's law. Thus, V does not equal to 0 uniformly within the space, as doing so would imply E = 0, contradicting Gauss's Law. $\endgroup$
    – lamplamp
    Commented Feb 3, 2020 at 23:09
  • 1
    $\begingroup$ @Eduardo Munguia No. "Grounded" only means that $V=0$ on the conducting shell. $\endgroup$
    – mike stone
    Commented Feb 3, 2020 at 23:09
  • $\begingroup$ The charge $q$ at the center of the hollow spherical conductor induces a total surface charge on the inside surface of the conductor which is equal and opposite in sign to $q$. And the induced surface charge on the inside surface of conducting sphere induces a surface charge which is equal and opposite on the outside surface of the conductor. When the outside of the conducting shell is grounded, the ground drains off the induced charge from the outside surface. $\endgroup$
    – Cinaed Simson
    Commented Feb 3, 2020 at 23:41
  • $\begingroup$ @Cinaed Simson Your explanation was very useful. $\endgroup$
    – eemg
    Commented Feb 4, 2020 at 0:04
  • $\begingroup$ Another way to look at it is that before connecting to wire, the charge q contained within the sphere creates an electric field outside the sphere, via induced charge that separates on the inner and outer surfaces of the sphere. This electric field, upon connecting the wire, pulls charge from the ground (earth) until the field disappears, which is when the added charge from the earth cancels out the original charge q. $\endgroup$
    – lamplamp
    Commented Feb 4, 2020 at 0:36

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.