Gyroscope - Transform data to correct coordinate system

Question

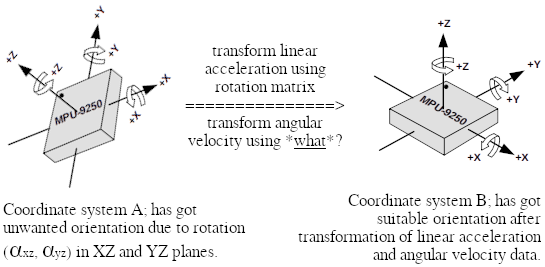
I have some data on linear acceleration ($m/s^2$) and angular velocity ($rad/s$) recorded from an electronic sensor over time.

I realized that the sensor had been displaced in its position during recording and I therefore need to perform a data correction mapping. So, assuming that the current coordinate system needs slight rotations in $XZ$ and $YZ$ planes to be corrected, correcting the linear acceleration data will be easy using a rotation matrix.

What about angular velocity of the gyroscope? AFAIK using usual vector calculus (i.e rotation matrix), transformation of angular velocity is not possible (true?). I've got the angular velocity of the gyroscope along 3 axes ($\omega_x$, $\omega_y$, $\omega_z$), and I need some transformation map to calculate correct angular velocities ($\omega'_x$, $\omega'_y$, $\omega'_z$).

---

TL;DR Is there an approach similar to using rotation matrix in case of angular velocity / spin, to transform data from rotated coordinate system $A$ to correct coordinate system $B$, when rotations applied in both $XZ$ and $YZ$ planes?

Answer 1

Regardless of what the nature of the recorded data is, all vectors transform the same. In other words, it doesn't matter whether its $\vec{a}$ or $\vec{\omega}$ as long as its a vector quantity. Whatever you used to correct the linear accelerations, $\vec{a}$, can exactly be used for $\vec{\omega}$ as long as the orientation of the sensor was fixed during recording.

Be careful though as to what needs to be rotated: the sensor or the vectors.
If $B=R A$, then $\vec{a}'=R^{-1}\vec{a}$.