I was studying circular motion and came across a formula for the relative angular velocity between two bodies. I didn't find any kind of demonstration. Is it simply a determined concept, just like the vector product or the angular velocity vector?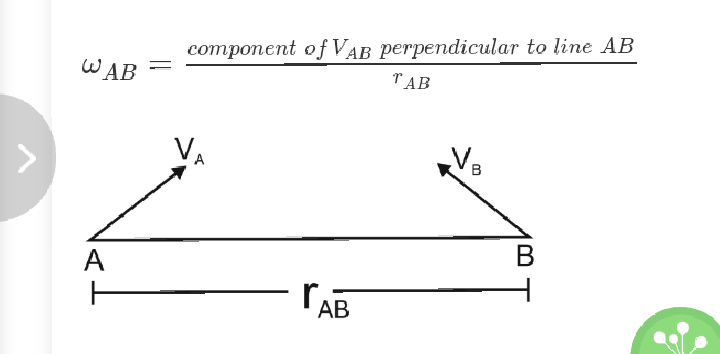
-
1$\begingroup$ The instantaneous relative angular velocity of two objects is just the angular velocity of one of them in the instantaneous inertial reference frame of the other. Think about a Galilean transformation into the frame of $A$ or $B$, and compute the angular velocity there. $\endgroup$– G. SmithCommented Nov 30, 2019 at 5:01
1 Answer
take this equation:
$$\vec{v}=\vec{\omega}\times \vec{r}$$
or
$$\vec{v}\times \vec{r}=\left(\vec{\omega}\times \vec{r}\right)\times \vec{r}\tag 1$$
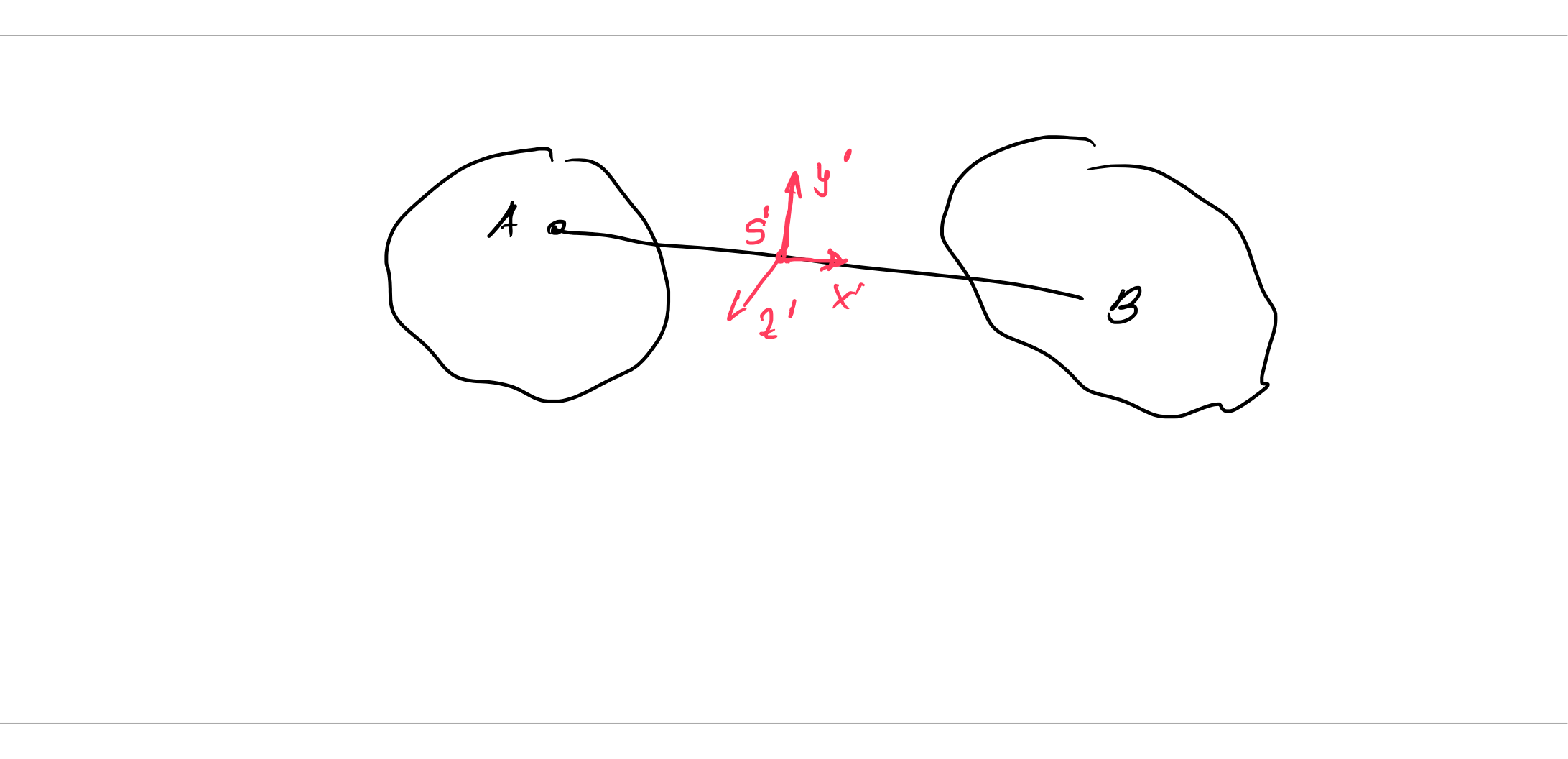
if you write all vectors in components of the S' coordinate system you get:
2D case
$$\vec{r}=r_x\,\hat{\vec{e}}_{x'}$$ $$\vec{v}=v_x\,\hat{\vec{e}}_{x'}+v_y\,\hat{\vec{e}}_{y'}$$ $$\vec{\omega}=\omega_z\,\hat{\vec{e}}_{z'}$$
in (1)
$$(v_x\,\hat{\vec{e}}_{x'}+v_y\,\hat{\vec{e}}_{y'})\times r_x\,\hat{\vec{e}}_{x'}=\left(\omega_z\,\hat{\vec{e}}_{z'}\times r_x\,\hat{\vec{e}}_{x'}\right)\times r_x\,\hat{\vec{e}}_{x'}\tag 2$$
thus:
$$-v_y\,r_x\,\hat{\vec{e}}_{z'}=-\omega_z\,r^2_x\,\hat{\vec{e}}_{z'}$$
or:
$$\omega_z=\frac{v_y}{r_x}\quad, \omega_{AB}= \frac{(V_{AB})_y}{r_{AB}}$$
this is your equation
