1
$\begingroup$

Let us say we have this configuration

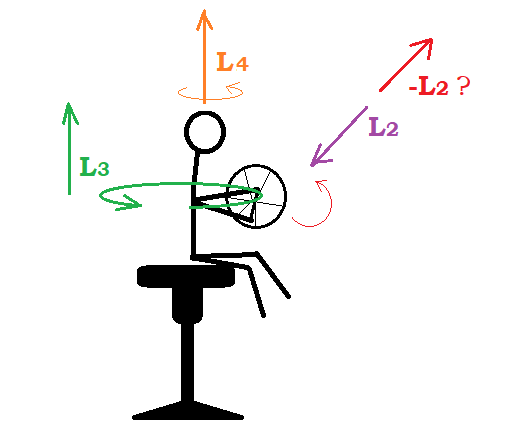
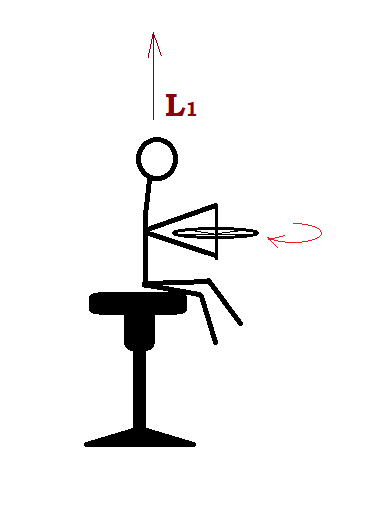
enter image description here

A person sitting on a rotating stool, which is initially at rest, holding a wheel that is rotating as indicated in the figure. The person then changes the axis of rotation of the wheel so that the new axis of rotation, initially, pierces the screen.

Initially, the system wheel$+$person had an angular momentum $\textbf{L}_{1}$ equal to the angular momentum of the wheel. In the new configuration, the person and the wheel (center of mass(CM) of the wheel) both start to revolve around the longitudinal axis of the stool; the person now has an angular momentum $\textbf{L}_{4}$ and the CM of the wheel an angular momentum $\textbf{L}_{3}$. The wheel has also another angular momentum $\textbf{L}_{2}$ due to its rotation around its axis.

According to conservation of angular momentum, the total angular momentum of the initial configuration must be equal to the total angular of the new configuration. Thus, direction-wise, there must be an angular momentum $-\textbf{L}_{2}$ that cancels $\textbf{L}_{2}$ at each instant.

Where does $-\textbf{L}_{2}$ exactly come from?

N.B. $\textbf{L}_{2}$ below is perpendicular to the screen (page). enter image description here

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ "According to conservation of angular momentum, the total angular momentum of the initial configuration must be equal to the total angular of the new configuration." That's not correct. The final angular momentum must be equal to the initial PLUS the angular impulse added by outside torques. The stool/earth system interacts with the person/wheel system and transfers angular momentum. Conservation laws ALWAYS include a continuity condition: New = Old + Flow. $\endgroup$
    – Bill N
    Commented Nov 27, 2019 at 16:34

1 Answer 1

Reset to default
1
$\begingroup$

The stool can rotate freely only about the vertical axis.

It can and does provide counteracting torque to any component of L perpendicular to that.

That allows it to provide the horizontal components of torque necessary to cancel the L2 in the diagram.

Physically, the stool is what’s preventing the person from rotating in pitch (head forward, feet back) and roll (head left, feet right). It does that by providing whatever torque is needed to resist motion. In that way it’s similar to the familiar restoring force in the block and plane example.

Improve this answer
$\endgroup$
3
  • $\begingroup$ But the stool rotates around the same axis as the person does. Its angular momentum, therefore, points toward the same direction that $\textbf{L}_{4}$ and $\textbf{L}_{3}$ point to; how can it cancel $\textbf{L}_{2}$? $\endgroup$
    – Hilbert
    Commented Nov 26, 2019 at 17:50
  • 2
    $\begingroup$ @Hilbert If, instead of a stool, the person doing the demo were mounted in a three-axis gimbal, you would expect to see conservation of $\mathbf L$ rather than $L_z$. But the horizontal components of $\mathbf L$ in a stool-mounted demo are compensated by combined motion of the stool, the floor it's on, the building it's in, and the rest of the Earth. Earth can absorb a lot of angular momentum by moving a very small amount because Earth is much heavier than most people. $\endgroup$
    – rob ♦
    Commented Nov 26, 2019 at 20:17
  • $\begingroup$ Added a bit on how the stool acts as a constraint. Let me know if that helps. $\endgroup$
    – Bob Jacobsen
    Commented Nov 26, 2019 at 20:33

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.