It's always great to see a contributor actually carrying out some experimentation, so welcome!
However, I feel your experimental protocol leaves somewhat to be desired to.
I took the aluminium and dunk it in water (25ºC and about 0.125 kg) while recording the temperature of the water through a temperature sensor.
150ºC is higher than the BP of water. It will cause temporary, localised boiling and you don't want that. Don't exceed 100ºC as initial temperature of the $\text{Al}$.
Your pieces of $\text{Al}$ seem very close together, this may reduce the effective surface area available for convection. Spread them out more.
Your set-up isn't insulated at all and the shallow water promotes heat loss to the environment. Use something like a styrofoam coffee cup (with lid) to avoid losses.
It may also be simpler and more accurate to measure the temperature increase of the water after some fixed period of time, $\Delta t$.
(Theres a tiny bump shown in the circle but I assumed its uncertainty
due to sensor and environmental factors so I considered it as an
exponential decay, is it actually uncertainty or related to what
explained below?)
You're assuming exponential evolution of the water temperature because you're thinking of Newton's Cooling Law (I assume). Because here both the water and $\text{Al}$ temperatures change, it's cautious to verify that, as I'll do below.
All parameters of $\text{Al}$ are indexed as $1$, all parameters of water are indexed as $2$.
Newton's Cooling Law teaches us that:
$$\frac{\text{d}Q}{\text{d}t}=-hA(T_1-T_2)\tag{1}$$
Assuming no heat losses (adiabatic system), then:
$$\text{d}Q=m_1c_1\text{d}T_1=-m_2c_2\text{d}T_2$$
$$m_1c_1\frac{\text{d}T_1}{\text{d}t}=-hA(T_1-T_2)\tag{2}$$
$$-m_2c_2\frac{\text{d}T_2}{\text{d}t}=-hA(T_1-T_2)\tag{3}$$
Divide $(2)$ by $(3)$:
$$-\frac{m_1c_1\text{d}T_1}{m_2c_2\text{d}T_2}=1$$
$$\text{d}T_1=-\alpha \text{d}T_2\tag{4}$$
Where:
$$\alpha=\frac{m_2c_2}{m_1c_1}$$
Integrate $(4)$:
$$\int\text{d}T_1=\int-\alpha \text{d}T_2\to T_1=-\alpha T_2+C_1$$
Determine integration constant $C_1$ from initial conditions:
$$T_{1,0}=-\alpha T_{2,0}+C_1$$
$$C_1=T_{1,0}+\alpha T_{2,0}$$
Insert all this into $(3)$:
$$-m_2c_2\text{d}T_2=-hA(-\alpha T_2+C_1-T_2)\text{d}t$$
$$m_2c_2\text{d}T_2=hA[C_1-(\alpha +1)T_2]\text{d}t\tag{5}$$
Make a simple substitution:
$$u=C_1-(\alpha +1)T_2$$
So that:
$$\text{d}u=-(\alpha +1)\text{d}T_2\to \text{d}T_2=-\frac{1}{\alpha +1}\text{d}u$$
Insert into $(5)$:
$$-\frac{1}{\alpha +1}\frac{{d}u}{u}=hA\text{d}t$$
$$-\frac{1}{\alpha +1}\int_{u_0}^{u(t)}\frac{{d}u}{u}=\int_0^t hA\text{d}t$$
$$\ln\frac{u(t)}{u_0}=-(\alpha +1)hAt$$
$$\frac{u(t)}{u_0}=\exp[-(\alpha +1)hAt]$$
$$\frac{C_1-(\alpha +1)T_2(t)}{C_1-(\alpha +1)T_{2,0}}=\exp[-(\alpha +1)hAt]$$
$$\boxed{T_2(t)=\frac{1}{\alpha +1}\Big[C_1-(\alpha+1)T_{2,0}\exp[-(\alpha +1)hAt]\Big]}$$
So that clearly the water temperature $T_2$ evolves at an exponential rate. At $t=+\infty$ both temperatures of $\text{Al}$ and water will have become equal and invariant. These final temperatures will be independent of both $h$ and $A$.
$$t=+\infty \to C_1-(\alpha +1)T_{2,\infty}=0$$
$$T_{1,0}+\alpha T_{2,0}-(\alpha +1)T_{2,\infty}=0$$
$$T_{2,\infty}=\frac{T_{1,0}+\alpha T_{2,0}}{\alpha +1}$$
We can also determine the cooling rate:
$$\boxed{\frac{\text{d}T_2(t)}{\text{d}t}=-(\alpha+1)T_{2,0}hA\exp[-(\alpha +1)hAt]}$$
Clearly, the cooling rate is proportional to $A$.
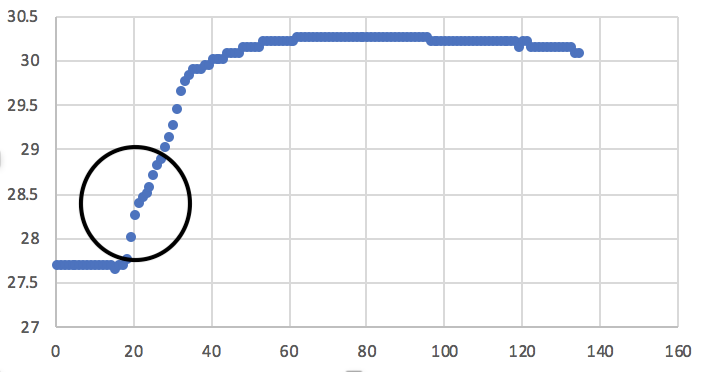(Theres a tiny bump shown in the circle but I assumed its uncertainty due to sensor and environmental factors so I considered it as an exponential decay, is it actually uncertainty or related to what explained below?)
