0
$\begingroup$

From what I understand, there can be no $E$-field inside a conductor in an electrostatic situation, because had there been any field within it, the charges on the surface would start to move, thus exiting the equilibrium state. In other words, the $E$-field must have no component along the equipotential surface.

But then, just under the inside surface of the conductor, what argument would prevent the $E$-field from existing under this form (the conductor is a hollow negatively charge sphere):

enter image description here

If ones takes a gaussian sphere with a slightly smaller radius, they could argue that the field cannot exist since there is no charge enclosed inside the surface.

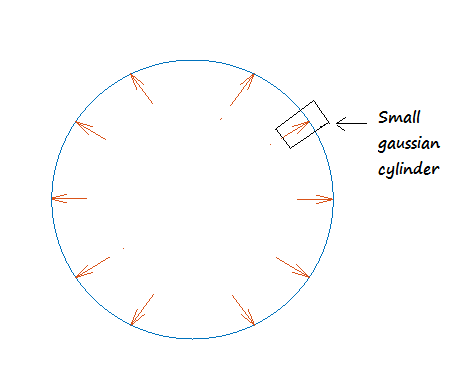
In this case I might as well decide to choose another gaussian surface that contain charges inside of it to justify the existence of the field:

enter image description here

Improve this question
$\endgroup$
7
  • $\begingroup$ "because had there been any field within it, the charges on the surface would start to move" Why do you limit this analysis to surface charges? Even though any excess charge will migrate to the surface, there are free charges in the bulk, as well. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Aug 12, 2019 at 18:20
  • 1
    $\begingroup$ Concerning your arguments about Gaussian surfaces, I'm reminded of the old joke: "At a conference, a mathematician proves a theorem. Someone in the audience interrupts him. 'But, sir, that proof must be wrong. I’ve found a counterexample.' The speaker replies, 'I don’t care — I have another proof for it.'" $\endgroup$
    – Michael Seifert
    Commented Aug 12, 2019 at 18:22
  • 1
    $\begingroup$ Which is to say that if you believe the argument about the "slightly smaller Gaussian sphere", then your second Gaussian surface is irrelevant; you've already proven that there is no field inside the shell. (The argument about the second surface fails because all it tells you is the difference between the interior & exterior field, not the value of either one.) $\endgroup$
    – Michael Seifert
    Commented Aug 12, 2019 at 18:23
  • $\begingroup$ @dmckee "Why do you limit this analysis to surface charges?" The conductor is a hollow sphere with negative charges on the surface. $\endgroup$
    – Hilbert
    Commented Aug 12, 2019 at 18:36
  • 1
    $\begingroup$ @Hilbert: That's correct; in general, you need to make an additional assumption of symmetry in order to find $\vec{E}$ using Gauss's Law. For example, it seems reasonable to assume that the electric field produced by a spherical shell should itself be spherically symmetric. If you make that assumption, then the electric field must be purely radial and have a magnitude only depending on $r$. For a spherical surface, this implies that $\int \vec{E}\cdot d\vec{a} = 4 \pi r^2 |\vec{E}|$. Then you apply Gauss's Law and find that $|\vec{E}| = 0$. $\endgroup$
    – Michael Seifert
    Commented Aug 14, 2019 at 18:39

1 Answer 1

Reset to default
1
$\begingroup$

You can have a spherically symmetric, outward, radial field in the hollow. If there is a positive charge at the center of the hollow (or some similar arrangement).

You can see why you need the interior charge by drawing a spherical Gaussian surface just inside the inner conductive surface. There is a net outward flux, implying positive net charge in the hollow. (Actually I do it by saying "Hey, those field line are diverging." and use that observation to guide my choice of Gaussian surface.)

The surface you've draw shows you, in detail, how the field from the interior positive charge can stop at the conductor, but it doesn't shed light on what is going on in the whole volume of the interior because Gauss' law tells you about things inside the surface you choice not outside of it.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.