Coefficient of friction in pulley system

Question

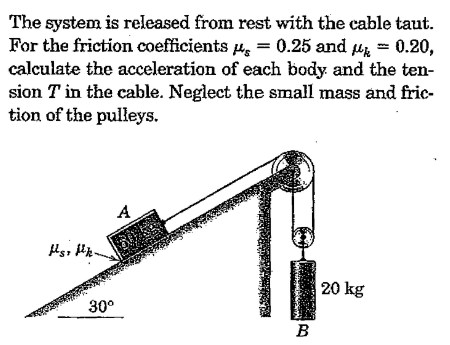
EDIT: Picture doesn't show the mass of $\mathrm A$. It is $60\ \mathrm{kg}$.

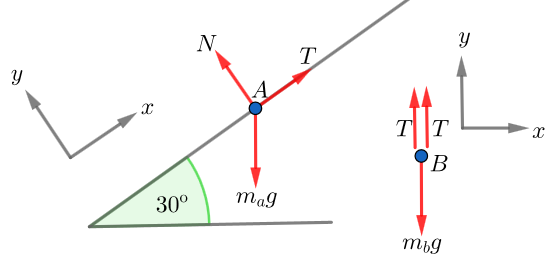
My attempt: I placed my coordinate system with $x$-axis parallel to the ramp surface and $y$-axis parallel to the normal force of block $\mathrm A$. Here are my free body diagrams of each block. Note how the coordinate orientations change depending on the block. Also note that I've omitted the friction force $F_\mathrm f$ since I don't know which way it should point at this time.

Looking at the system if it is at rest, we get that for block $\mathrm B$ the sum of forces in $y$ direction is

$$\sum F_{\mathrm b,y}=m_\mathrm ba_{\mathrm b,y}\implies2T=m_\mathrm bg\implies T=\frac{m_\mathrm bg}{2}\approx 98.1\ \mathrm N.$$

For block $\mathrm A$ we have forces in both $y$ and $x$, thus

$$\sum F_{\mathrm a,y} = m_\mathrm aa_{\mathrm a,y} \implies N=m_\mathrm ag\cos{30}=\frac{\sqrt{3}m_\mathrm ag}{2}\approx509.74\ \mathrm N.$$

$$\sum F_{\mathrm a,x} = m_\mathrm aa_{\mathrm a, x} \implies T+F_\mathrm f-m_\mathrm ag\sin{30}=0\implies F_\mathrm f=\frac{m_\mathrm ag}{2}-T\approx 196.2\ \mathrm N.$$

Using $\mu_\mathrm s=0.25$ we find the maximum friction force such that the system is starts moving: $F_{\mathrm f,\mathrm{max}}=N\mu_\mathrm s=509.74\ \mathrm N\cdot 0.25=127.4\ \mathrm N.$

Questions:

1. How does $F_{\mathrm f,\mathrm{max}} \lt F_\mathrm f$ imply that block $\mathrm A$ slides downwards?
2. When does the kinetic coefficient $\mu_\mathrm k$ come into play?

Answer 1

How does $F_{f,max}<F_f$ imply that block 𝐴 slides downwards?

It is when $m_{a}g$ sin 30 is > $F_{f,max}+T$ where $F_{f,max}=μ_{s}N$ and acts upward that block A slides downwards.

When does the kinetic coefficient 𝜇𝑘 come into play?

It comes into play when you calculate the actual tension in the string when the blocks are accelerating, because the kinetic coefficient is applicable when the block is sliding, whereas the coefficient of static friction comes into play in resisting any motion, up to when the maximum static friction is reached.

The challenge of this problem, as you surmised, is determining which direction the friction force acts (which will be in the opposite direction that block A moves). I approached it in the following way, which is not necessarily the only way or the most efficient way.

I assumed impending motion of block A is downward along the plane (i.e., upward static friction is at a maximum) and computed the tension that must be in the string in order for impending (but not actual) motion to occur. Then I used that value of tension to see what would be happening to block B. When I did I found block B would be accelerating upward. This told me that block A had to be sliding downwards. To put it another way, without tension in the string the maximum static friction force would be insufficient to prevent downward motion of block A. It’s just a matter of determining how much tension is needed.

With the above in mind, I could next determine the actual acceleration and tension in the string. But to do so, $F_{f}$ acting up the plane will now be the kinetic friction force and not the static friction force, so I used the coefficient of kinetic friction instead of static friction since the block is moving. Since the magnitude of the acceleration of block A is the same as block B I now have two equations and two unknowns ($T$ and $a$) that can be determined.

Hope this helps.

Answer 2

$F_{f,max}<F_f$ implies that the frictional force required to keep the system at rest is too less and, hence, the blocks have to accelerate. Since $F_f$, which was got to be upwards along the wedge, is insufficient in that direction, block A will accelerate downwards along the wedge.

Now you would have to work out the solution again considering the accelerations of the blocks and in doing so you would have to account for the kinetic friction acting on block A as we have shown that it is moving (thus, the coefficient of kinetic friction comes into play here).