Tension in a string attached to a spring with deformation at a point

Question

I am trying to calculate the tension in a string in the configuration shown in the diagram below. Here is a verbal description of the setup: The string is fixed at one end with the other end connected to a spring. The spring, with constant $K$, is limited to only vertical movement. The string begins with a tension $T$, which causes the free end of the spring to extend from its resting position $S_0$ to position $S_0+S$ such that the following relationship is upheld:$$F_s=K\cdot S=T\cdot\frac{h}{\sqrt{D^2+h^2}}$$ A distance $x$ along the horizontal from the fixed end of the string, a force $F$ is applied vertically downward. This force is such that the string is forced into the configuration shown with a tension $T_1$. The spring will naturally extend to accommodate this deformation until the free end is $h_1$ above the horizontal line defined by the segment labeled $x$. It seems to me that if the string has cross-sectional area $A$ and Young's modulus $Y$ then it should be possible to determine the tension $T_1$ as a function of $A$, $Y$, $x$, $T$, $D$, and $h$. 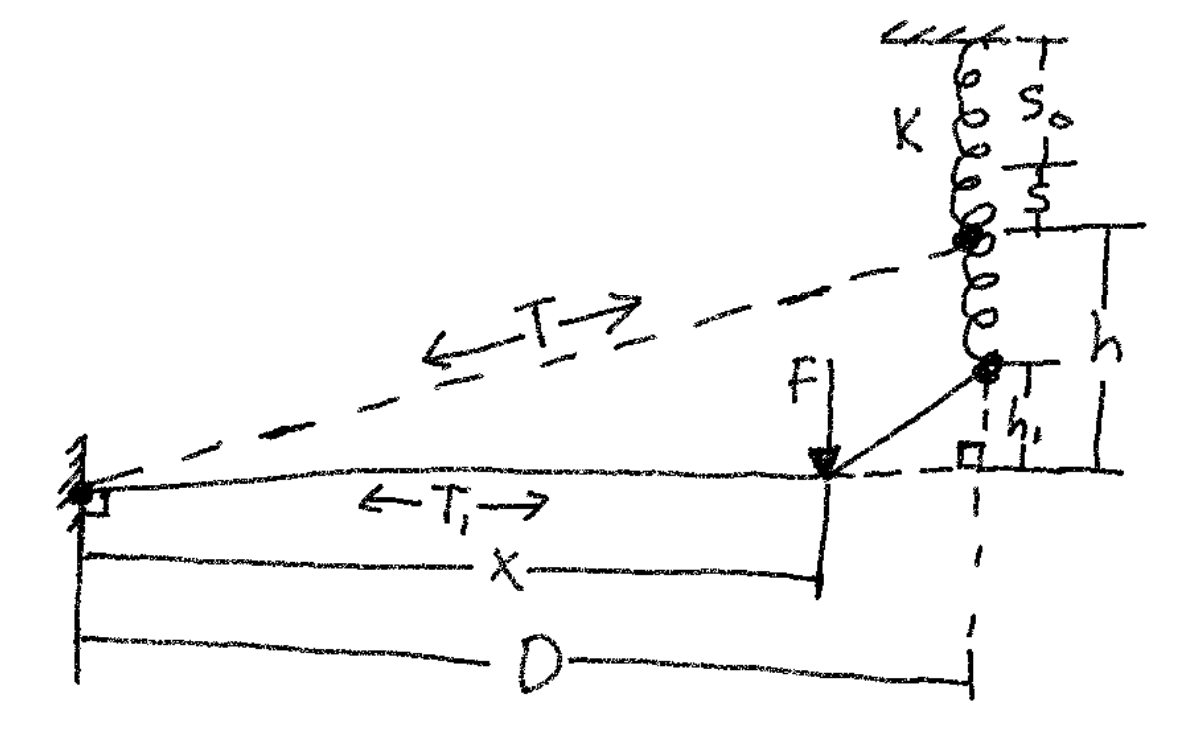$S_0$ is not relevant to the calculation at all. I can calculate $S$ using $$S=\frac{T}{K}\cdot\frac{h}{\sqrt{D^2+h^2}}$$ I should be able to calculate $$T_1=K(S+h-h_1)\cdot\frac{\sqrt{(D-x)^2+h_1^2}}{h_1}$$ and from previous problems I know that I can calculate $$T_1=(T+AY)\frac{x+\sqrt{(D-x)^2+h_1^2}}{\sqrt{D^2+h^2}}-AY$$ However, I can't for the life of me find a way to write $h_1$ in terms of the other variables so that I can remove it from either equation for $T_1$. I think it should be possible since $h_1$ is simply an equilibrium point, but I've been unable to work it out myself. I'm probably missing something obvious, but some other eyes on this problem would be greatly appreciated!

Answer 1

I assume the length of the rope remains constant. At first the length is given by:

$$L=\sqrt{D^2+h^2}$$

After applying the force, the length is given by:

$$L=x+\sqrt{(D-x)^2+h_1^2}$$

By assuming $L$ is constant, one can derive an expression for $h_1$:

$$\sqrt{D^2+h^2}=x+\sqrt{(D-x)^2+h_1^2}$$

$$\left(\sqrt{D^2+h^2}-x\right)^2=(D-x)^2+h_1^2$$

$$h_1 = \pm \sqrt{\left(\sqrt{D^2+h^2}-x\right)^2-(D-x)^2}$$