5
$\begingroup$

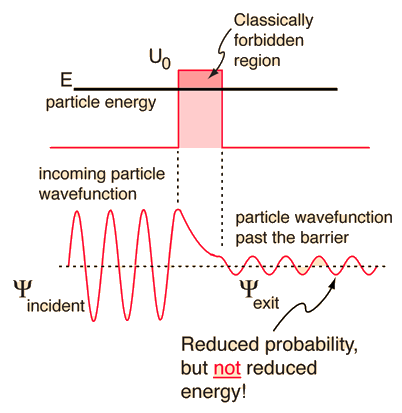
According to my understanding quantum mechanics, the probability of any particular particle in the universe being at any specific location in the universe is very small but never actually becomes zero. Thus, a little bit of all of us is everywhere.

Let's assume I suddenly quantum tunneled from sea level to the top of Mount Everest. That jump represents a net increase in energy/mass for Earth, which would violate the first law of thermodynamics. My calculations indicate it would have increased the mass of Earth by around 0.0858657µg, unless it is somehow offset some other phenomenon.

It seems implausible to me that energy conservation for a closed system could actually be violated, so how would that net increase in energy/mass be accounted for?

Or does the fact that such a scenario by definition represents such a significant decrease in the entropy of the system mean that an increase in mass should be the expected result?

Improve this question
$\endgroup$
3
  • $\begingroup$ Can you please elaborate why "That jump represents a net increase in energy/mass for Earth"? $\endgroup$
    – Árpád Szendrei
    Commented May 22, 2019 at 4:10
  • 1
    $\begingroup$ @Árpád Szendre it would have more potential energy. The subject could then fall again to sea level and acquire kinetic energy it did not previously have. $\endgroup$
    – rghome
    Commented May 22, 2019 at 9:07
  • $\begingroup$ In my example, I have "Earth" represent the combined system of the planet and everything on it's surface or in its atmosphere. Raising myself manually from sea level to 8000 meters requires energy—energy that would be released if I fell. If I were to climb that height, there would be no net effect on the total mass of the system "earth". However, in my example, I didn't climb: I tunneled. Where did that energy come from? If it came from nowhere, that would amount to an effective increase in the mass of the combined "Earth" system. $\endgroup$
    – Robert Quattlebaum
    Commented May 22, 2019 at 22:29

1 Answer 1

Reset to default
4
$\begingroup$

Here are the basics of quantum tunneling:

barrier penetration

Note, the energy level of the particle in the above simple example does not change.

This holds true for all tunneling scenaria:

There should exist wave functions $Ψ$ , i.e. solutions for the quantum mechanical equations applicable to the problem where the specific boundary conditions have been applied. Then the $Ψ^*Ψ$ computation will give the probability for the particle to be found outside the barrier.

Energy conservation is a universal rule that is incorporated in all wavefunction calculations. Thus the probability of finding the particle in a different energy level than the one in the solution is zero, no matter how complex the solutions and the boundary conditions.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Note that in my example, my own energy level did not change: the energy level of the closed system of the earth (or the solar system, or the galaxy) changed. Alternately, are you saying that my wave function has a probability of exactly zero at the top of Mount Everest? $\endgroup$
    – Robert Quattlebaum
    Commented May 22, 2019 at 22:06
  • $\begingroup$ Yes, because of energy conservation your wavefunction can have a finite probability to tunnel only at the same energy level that your are now in.. It does not have a nonzero solution from - infinity to + infinity of space time, there are boundary conditions defining your energy level, and that level constrains your probability by conservation of energy which is an absolute law at the measurement level. $\endgroup$
    – anna v
    Commented May 23, 2019 at 3:50

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.