Musical instruments generally produce sound waves at a collection of frequencies, even when playing a single note. For instruments which make distinct pitches, these frequencies are roughly multiples of a fundamental frequency, which determines the perceived pitch. The perceived brightness is determined by the strength of higher frequencies, in the kHz range. A percussive instrument like the gong obeys the same principles, except that generally there are a whole lot more frequencies present.
If the behavior of a percussive instrument is well-described by linear differential equations, you would expect all of the frequencies to act independently. Their strength would be determined by the initial strike, and then they would each exponentially decay, perhaps at different rates. This is a good description for, e.g. pianos, but not for the gong. According to Fletcher and Rossing's exhaustive work The Physics of Musical Instruments:
When we seek a physical origin for the extreme nonlinearity of the tam-tam [the Chinese gong], we find this first in the fact that its metal is thin and not under significant tension. This means that the stiffness and tension restoring forces are very small, so that the quadratic tension generated by mode displacements ·has a large effect. Another source of mode coupling and nonlinearity arises from the hammered bumps in the surface, since abrupt changes in slope are known to generate mode coupling and nonlinear frequency multiplication.
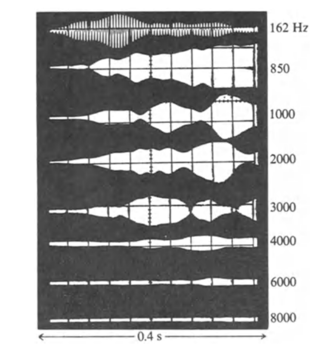
When the gong is struck, most of the energy goes into low frequencies, and nonlinear effects feed it into higher frequencies only gradually. For example, Fletcher and Rossing (1982) collect the following data for the buildup and decay of vibrations in different frequency bands:
Quoting The Physics of Musical Instruments again:
The initial large excitation of low-frequency modes is apparent, as is the subsequent transfer of vibrational energy to modes in the range 1- 5 kHz, which contribute the late-developing "sheen" to the sound.
Fletcher and Rossing don't mention "warming up" the gong at all, but presumably the point of this is to get some energy into the higher-frequency modes from the beginning, so that they can build up quicker, giving a brighter and more immediate sound. (Of course, this "warming up" has nothing to do with the temperature of the gong.)
That's presumably the canonical explanation, but I still find it a little puzzling why light taps can have such a large effect. In the simplest possible nonlinear equations, the amplitude $a_l$ for the low, excited modes feed into higher modes $a_h$, making them grow linearly in time. For example, one might have the leading behavior
$$\dot{a}_h \sim a_l^n, \quad a_l \sim e^{-t/\tau}$$
if the high frequency is $n$ times the low frequency. So starting with a small amplitude for the high-frequency modes wouldn't make that much of a difference.
I'm going to make a wild guess about what else is going on here. From Fletcher and Rossing again:
The low-frequency domain has several prominent axisymmetric modes, which absorb much of the energy of the initial blow. [...] Other families of vibrational modes of considerable interest arethose that have a number of radial modes equal to the number (or an even multiple) of hammered bumps in one of the circles. These modes would be favored in the delayed sound...
If the high-frequency modes break a symmetry that the low-frequency modes don't, then the rate of change of $a_h$ has to be proportional to $a_h$ itself. This changes the growth from linear to exponential, so starting with a nonzero value of $a_h$ really can make a significant difference. (However, in the absence of any warmup, energy can still be transferred to the asymmetric modes by asymmetric bumps on the gong's surface; it just takes longer to get started.)

