The ladder paradox - with equal lengths

Question

Take the usual ladder paradox in special relativity, but this time the ladder and the barn have the same length, at rest. Since each one of them contracts when viewed in the rest frame of the other, I would guess that:

• an observer on the ladder would see that the barn is too small and the ladder doesn't fit inside,
• an observer at the barn would see that the ladder is contracted so now it can fit in the barn with room to spare.

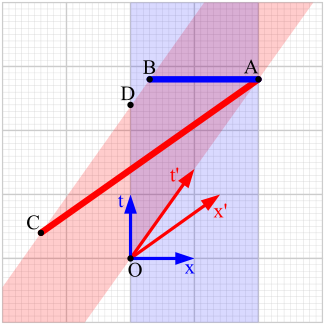
My problem is, I tried solving it with the diagrams in Minkowski spacetime (drawing the barn as two parallel lines and intersecting with the two lines of the ladder, etc.). Basically the diagram turns out to be like this one (from https://en.wikipedia.org/wiki/Ladder_paradox)

except that the red segment has a length equal to the horizontal width of the blue band, that is two "big squares" of the grid. Wikipedia says (referring to the diagram in the image):

We see that such line segments never lie fully inside the blue band; that is, the ladder never lies fully inside the garage.

But in my case, the red segment can very well fit inside the blue band, and I think this tells me that the ladder would fit in the barn even in the ladder rest frame.

I think I did something wrong with this reasoning, but I can't find what it is...

Answer 1

Here is what the spacetime diagram looks like for two inertial observers with the same-sized ladder (5 units long), one at rest, and the other moving with (6/10)c. [If you wish, you can say that the observer at rest in this frame has a barn that is just as long as the ladder (also 5 units).]

I've drawn the spacetime diagram on rotated graph paper so we can more easily see and count the ticks along the various segments, as well as see the orthogonal directions showing an observer's "space" is perpendicular to her "time".

Note that, in Minkowski-spacetime, when the back-ends of the ladders meet at event O,
the front-ends are on a common hyperbola with radius 5,
which is unlike the situation for Euclidean geometry and its circle.

For v=(6/10)c=(3/5)c, the gamma factor $\frac{1}{\sqrt{1-v^2}}=(5/4)$.

Note that the observer-at-rest measures the other ladder to be only $\frac{5\ \rm units}{(5/4)}=4$ units long.
And by the principle of relativity for these inertial observers,
the observer-in-motion measures the other ladder to be only $\frac{5\ \rm units}{(5/4)}=4$ units long.

I included an additional set of worldlines that are 4 units away. This would be useful to study the alternative problem if the measured lengths in the same reference frame were equal. In that case, each observer would measure the other ladder to be $\frac{4\ \rm units}{(5/4)}=(16/5)=3.2$ units long.