Looking at this image, it is said that the two triangles are similar. How are they similar? I can't seem to figure it out.
I understand that they're both isosceles triangles, but how can I prove that $\angle CAB$ is the same $\angle RPQ$ in the derivation of $$ a=\frac{v^2}{r}$$
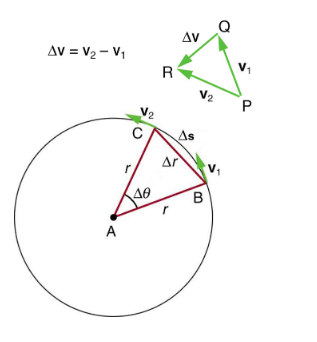
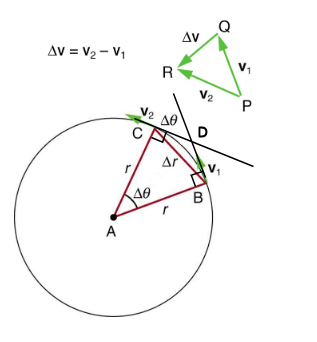
In the main diagram AB is perpendicular to v1, and AC is perpendicular to v2.
If you rotate the line AB anticlockwise by 90 degrees it will be in the direction of v1.
If you rotate the line AC anticlockwise by 90 degrees it will be in the direction of v2.
Make a V shape with your fingers, now rotate your hand 90 degrees.
Both fingers rotate by the same angle so the angle between your fingers doesn't change.
So the angle CAB in the magenta triangle is the same size as the angle RPQ in the green triangle.
Both triangles are isosceles, therefore the other two angles are the same for both triangles.
Of course the length of the sides may not be the same so the triangles may not be congruent.
But the angles are the same so the triangles are similar.
Two sides in the same ratio and the included angle the same make triangles similar.
Find angle CDB using quadrilateral CDBA and note that the magnitudes of the two velocities $\vec v_1$ and $\vec v_2$ are the same.
Okay, it is not so evident. Recall that velocities are tangent to the trajectory, thus perpendicular to the radii.
If you call $\theta_1$ and $\theta_2$ the angles of the radii, with respect to the $x$ axis, you'll see that velocities form an angle $\pi/2 + \theta_1$ and $\pi/2 + \theta_2$, but their difference is the same $\Delta\theta$, so the triangles are similar.
