Why does the moon sometimes appear giant and a orange red color near the horizon?

Question

I've read various ideas about why the moon looks larger on the horizon. The most reasonable one in my opinion is that it is due to how our brain calculates (perceives) distance, with objects high above the horizon being generally further away than objects closer to the horizon.

But every once in a while, the moon looks absolutely huge and has a orange red color to it. Both the size and color diminish as it moves further above the horizon. This does not seem to fit in with the regular perceived size changes that I already mentioned.

So what is the name of this giant orange red effect and what causes it?

Answer 1

(Source, Wikipedia Commons)

The moon is generally called a "Harvest Moon" when it appears that way (i.e. large and red) in autumn, amongst a few other names. There are other names that are associated with specific timeframes as well. The colour is due to atmospheric scattering (Also known as Rayleigh scattering):

may have noticed that they always occur when the Sun or Moon is close to the horizon. If you think about it, sunlight or moonlight must travel through the maximum amount of atmosphere to get to your eyes when the Sun or Moon is on the horizon (remember that that atmosphere is a sphere around the Earth). So, you expect more blue light to be scattered from Sunlight or Moonlight when the Sun or Moon is on the horizon than when it is, say, overhead; this makes the object look redder.

As to the size, that is commonly referred to as the "Moon Illusion", which may be a combination of many factors. The most common explanation is that the frame of reference just tricks our brains. Also, if you look straight up, the perceived distance is much smaller to our brains than the distance to the horizon. We don't perceive the sky to be a hemispherical bowl over us, but rather a much more shallow bowl. Just ask anyone to point to the halfway point between the horizon and zenith, and you will see that the angle tends to be closer to 30 degrees as opposed to the 45 it should be.

University of Wisconsin discussion on the Moon Illusion.

NASA discussion on moon illusion.

A graphical representation of this:

Dr. Phil Plait discusses the illusion in detail.

Answer 2

The two effects are not related.

The size appearing larger is a matter of some speculation to this day, but it is purely a psychological effect. If you want to prove this, take a look a the moon while standing up and looking between your legs. It won't look nearly as large.

The red/orange color is related to the sunset being red. In fact, it's the same thing exactly. The blue and green light has already been scattered, leaving only the red/orange light. This can be exacerbated by any of the things which cause sunsets to be more vivid, including pollution, clouds, dust, volcanic activity, etc.

Answer 3

It is an optical illusion. It only looks bigger near the horizon because it can more easily be compared to familiar objects on the ground. If you hold up a coin in front of your line of sight while looking at the moon and then compare your arm extension for a low moon and a high moon you see that they are the same. IOW, the diameters are the same.

Don't have a link because I learned this from the late night PBS astronomy show Jack Horkheimer: Star Gazer years ago and I don't think Jack would lie to us ;o)

Edit to answer the color question. At a low angle your line of sight is cutting through more atmosphere, so the "color saturation" goes up depending on what gases are in the atmosphere at that particular time and area.

Answer 4

1. Analysis

As shown in the figure, $HG$ is the lens, $w$ is the height of the imaged object $AC$, $x$ is the height like $JM$, $v$ is the image distance, $u$ is the object distance, and $f$ is the focal length. The red line is the light path. $DE$ is the screen.

We all know that the relationship between $u$, $v$, and $f$ is

$\cfrac{ 1}{ u }+ \cfrac{ 1}{ v}= \cfrac{ 1 }{ f } (1)$

$f = \cfrac{uv }{ u + v } $

From similar triangles we can see:

$\cfrac{ x}{ w }= \cfrac{ v-f}{ f }= \cfrac{ v }{ f }-1$

and so

$x = w(\cfrac{ v}{ f }-1)(2)$

From equation (2), we can see that if $w$ and $v$ are unchanged and $f$ decreases, then $x$ will increase.

It is also the reason why the human eye sees the moon on the horizon so it grows bigger. When looking at the horizon and the moon, due to the influence of the ground scenery (the distance is much smaller than the moon), the human eye uses a relatively short focal length, so the image of the moon is relatively large, although the image of the moon is not so clear; When the moon is at the top, the human eye uses a longer focal length, so the image of the moon is smaller, but the image of the moon is clearer.

The image distance $v$ does not change, and the object distance $u$ also does not change. Will changing the focal length blur the moon's image? This will not be blurred within a certain limit, because the moon is very far away, the object distance $u$ is large, and the aperture of the human eye is relatively small, so the moon imaging has a large "depth of field."

2. Magnification

Let $K$ be the magnification, look at the zenith moon's focal length as $f$, and image height as $x$, and look at the horizon moon's focal length as not $F$, and image height as $X$. Then according to formula (2)

$K = \cfrac{ X}{ x } =\cfrac{ \frac{ v}{ F }-1}{ \frac{ v}{ f }-1 } = \cfrac{ \frac{ 1}{ F }-\frac{ 1}{ v }}{ \frac{ 1}{ f }-\frac{ 1}{ v }} (3)$

$F ＜ f$

If the object distance of the zenith moon is exactly the distance $u$ from the earth to the moon, set

$\cfrac{ 1}{ U }= \cfrac{ 1 }{ F }-\cfrac{ 1}{ v }$, then (3)

$K = \cfrac{ \frac{ 1}{ U } }{ \frac{ 1 }{u } }= \cfrac{ u }{ U }$ (4)

It can be seen that the smaller $F$ is, the smaller $U$ is, and then the larger $K$ is, the larger the magnification is.

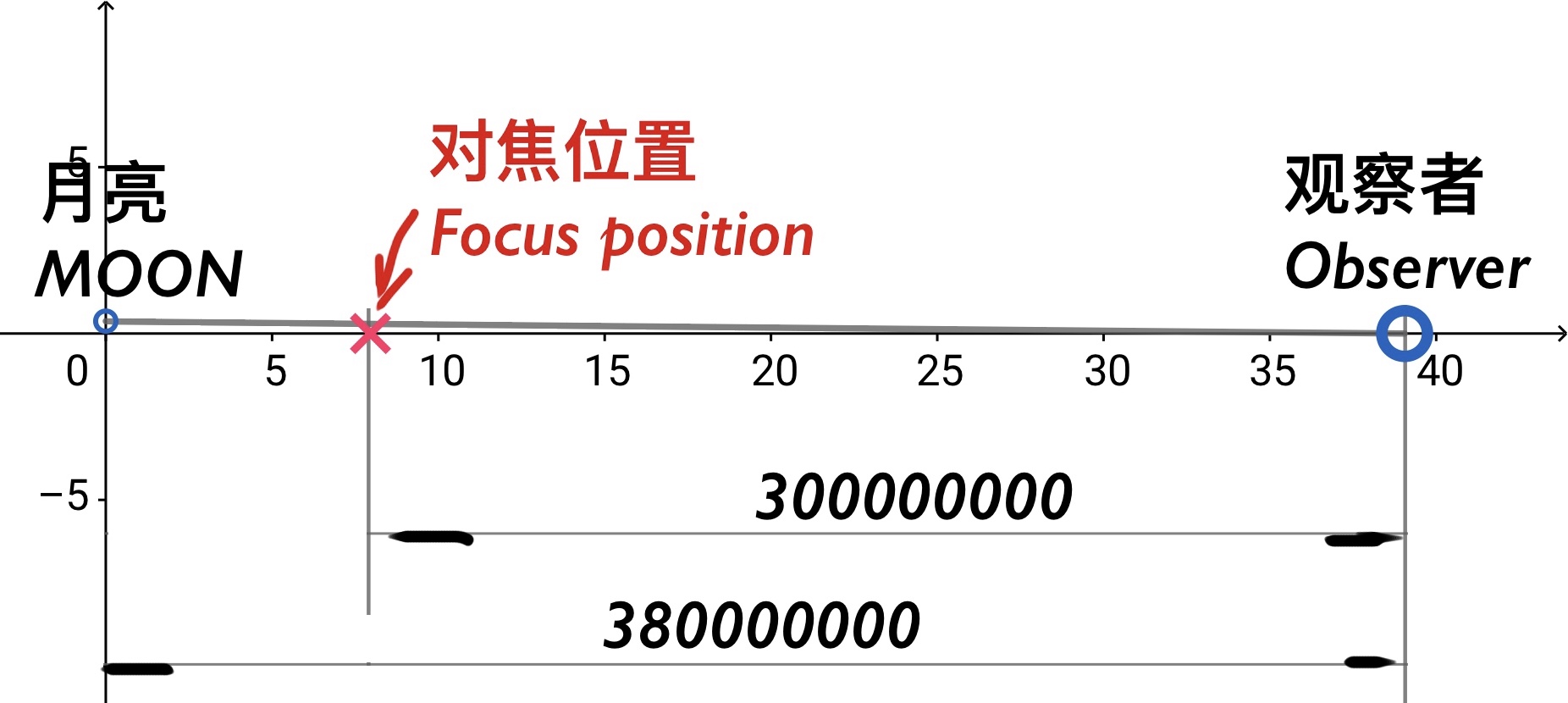
If you focus at $300,000$ kilometers, the magnification is

$K = \cfrac{ 38}{ 30 } = 1.266$ times

3 Discussion

When shooting with a camera, the moon above the head is the same size as the moon on the horizon. This is because the camera shoots with the same focal length. This is different from human eyes. Due to the influence of ground scenery and viewing habits, the focal length of the human eye when observing the moon close to the ground will be shorter than that of the zenith moon.

4 Conclusion

I think the moon illusion is the result of observing the moon with a relatively short focal length. I think this is the reason for the moon illusion.