I was just wondering if there would be a difference in the magnetic field inside a wire carrying AC current as compared to a wire carrying current through its cross sectional area. I want to know if the skin effect of AC current would make any difference.
I am aware that the magnetic field inside is given by $\left[\frac{uI}{R^{3}}r\right]$ for a wire with current through its cross sectional area.
Quoting Hyperphysics website, the Ampere's law, defining magnetic field surrounding a current, states that "for any closed loop path, the sum of the length elements times the magnetic field in the direction of the length element is equal to the permeability times the electric current enclosed in the loop.
The law talks about currents - not about wires. So, if we want to calculate magnetic field inside a wire, along a circle of radius r, we just have to calculate the magnitude of the current flowing through that circle and apply the Ampere's law.
In case of a DC current, which has a uniform density, we would arrive to the formula you've mentioned in your question, B = μIr/2πR^2, where I is a total current in the wire or radius R.
In case of an AC current, which tends to have uneven density, with most of the current concentrated near the walls (skin effect), this formula would not be applicable. So, to find the magnetic field along a circle of a given radius, the current flowing through that circle would have to be re-calculated based on the knowledge of the current density distribution as a function of the radius.
But to answer your question, we don't need these calculations, since it is clear that with most of the current pushed to the walls, less current would be flowing through the circle of radius r and, therefore, the strength of the magnetic field along that circle would be lower than in the DC case.
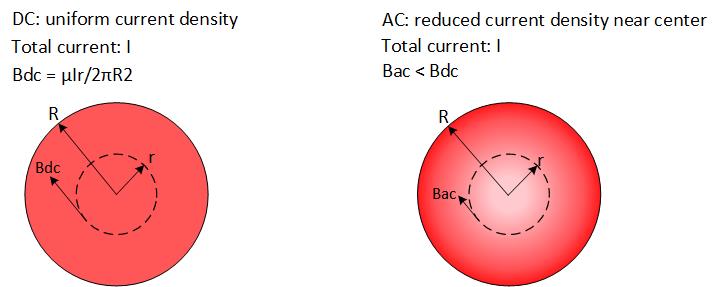
Moreover, given the same wire and the same total current, the magnetic field inside the wire carrying an AC current of sufficiently high frequency would be lower than the magnetic field inside the wire carrying a DC current, everywhere in the wire except on its surface, where the magnetic fields of AC and DC currents would equalize, since at that radius all the current in the wire will be encircled in both cases.
