Here we have Hooke's Law (not in vector form),
$$F=kx,$$
with $k$ being the spring constant and $x$ being the amount displaced from equilibrium (wiki).
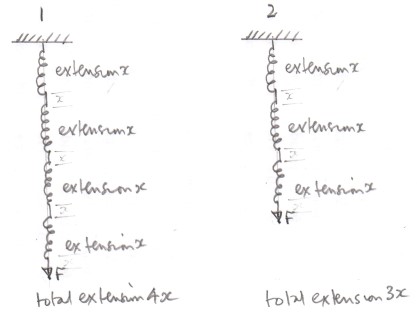
My question deals with the fact that it seems like we are missing something here; let me explain. Say you buy two identical springs and they both have the same $k$ and have 10 windings per meter. Now you cut one meter off of one, hence the only difference is one is originally 3 meters and the other is 4 meters (hence their equilibrium locations).
They both have 10 windings per meter when un-stretched/compressed.
Now, logically, the force is also proportional to the amount of (windings per meter - original windings per meter) also even as a young boy I knew that the longer I made the yarn, the further I could stretch it (same force yet different $x$). That being said, both springs are now stretched one meter. According to Hooke's Law the same amount of force was used to do this. But this doesn't resound well with the fact that now one has 7.5 windings per meter and the other has 8 windings per meter.
So can someone please explain where the logic is no longer sound.