0
$\begingroup$

Let there be a wheel which rotates around an axis , just like that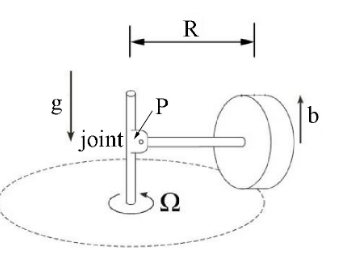

I am trying to calculate the angular momentum about point P. To make matters simpler, let's say that the wheel is slipping, that is, it isn't rolling and that it has zero thickness, it is two-dimensional.

My question is, is it correct to state that the velocity of the whole wheel is the same as the velocity of its center of mass?

I'm not sure about it because, since the axle is precessing with angular velocity $\Omega$ and the wheel is attached to the axle, it must be moving as well with angular velocity $\Omega$. Consequently the velocity of every part of the wheel must depend on its position.

Improve this question
$\endgroup$
5
  • $\begingroup$ Different points in the wheel will have different velocities at different times. $\endgroup$
    – Mauricio
    Commented Mar 26, 2018 at 13:18
  • $\begingroup$ @Mauricio Yes, I know. But what about at the same time? Is the wheel moving as a whole or isn't it? $\endgroup$
    – Gamabunto
    Commented Mar 26, 2018 at 13:26
  • $\begingroup$ If the wheel doesn't rotate every point in the wheel would have the same velocity. $\endgroup$
    – Mauricio
    Commented Mar 26, 2018 at 13:54
  • $\begingroup$ If there’s no friction, treat the whole wheel as a point(center of mass). If there is friction consider the velocity of the wheel. $\endgroup$
    – physics2000
    Commented Mar 26, 2018 at 14:03
  • $\begingroup$ @physics2000 But if I do so, then the wheel isn't precessing at the same speed, there are some points thereof which are slower. $\endgroup$
    – Gamabunto
    Commented Mar 26, 2018 at 14:06

1 Answer 1

Reset to default
1
$\begingroup$

No, it's not correct to state that velocity of the whole wheel is the same as velocity of it's center of mass. (I assume that "velocity of the whole wheel" means "velocity of every point of the wheel is the same and we call it 'velocity of wheel'")

Even if the wheel is not rolling, the speed (magnitude of velocity) of different points of the wheel is different. Because the speed is proportional to the distance from the point to the rotation axis. Obviously it's different for different points of the wheel even if the wheel has zero thickness.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.