X component changes, because measuring rod of moving observer Lorentz -contracts and observer measures x – component with contracted ruler.
That means, from the "point of view" of moving observer x – component stretches as velocity increases.
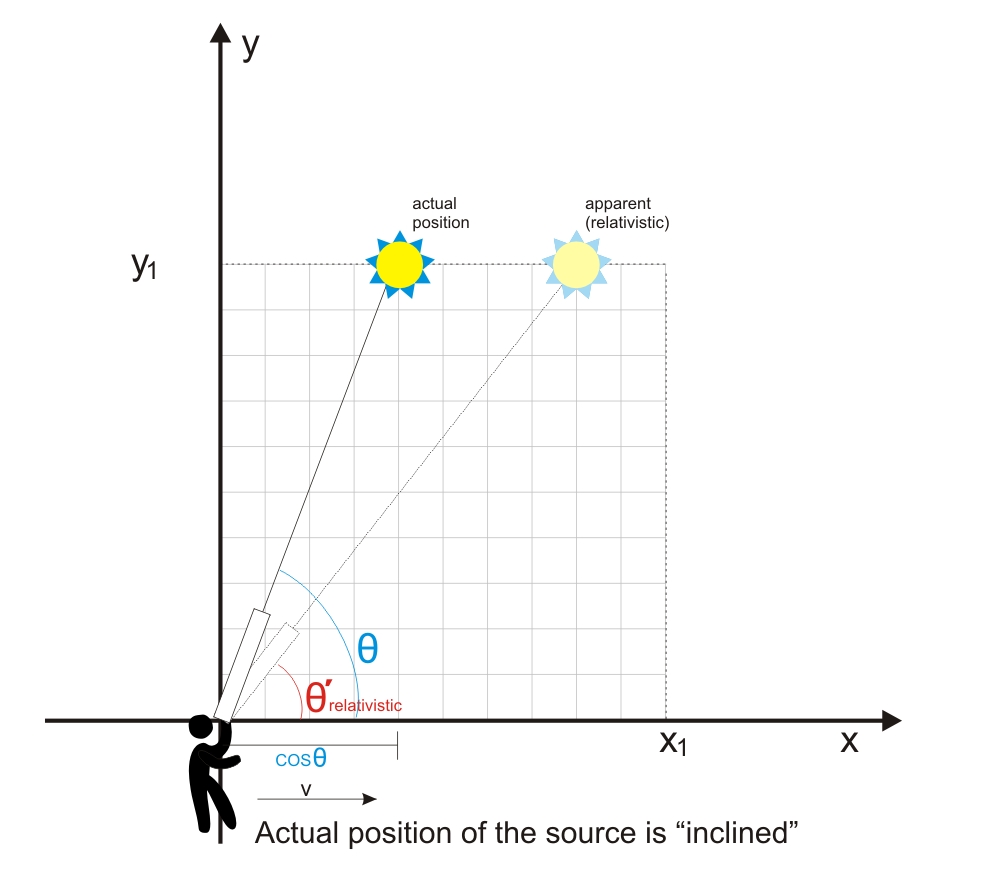
If observer moves with velocity v, and receives pulse of light at points of closest approach with the source (at this moment the source actually is over his head, in zenith, at angle $\theta = 0$) he will see this source at angle $\tan \theta’= \frac {v/c} {\sqrt {1-v^2/c^2}}$. While vertical distance travelled by light will be $ct$, horizontal travelled by observer will be $vt$. That means, that in classical case if observer moves with velocity $v=c$, maximum angle would be $\pi/4$ but in relativistic case (if $v$ is very close to $c$) due to length contraction of measuring rod of the observer the source appears to be much further, almost in the front of him on the horizon at angle $\sin \theta'=v/c$.
34.8. Aberration: http://www.feynmanlectures.caltech.edu/I_34.html
Further we plot the angle from the abscissa axis:
$$\tan \theta’ = \frac {\sin \theta} {\gamma (\cos \theta + v/c)} = \frac {\sin \theta \sqrt {1-v^2/c^2} } {\cos \theta + v/c}$$
Derivation from Lorentz Transformations:
https://en.wikipedia.org/wiki/Stellar_aberration_(derivation_from_Lorentz_transformation)
Received frequency also increases, i.e. due to dilation of observer’s clock he will see that the clock of the source is ticking faster than his own.
$$ f_o = \frac {f_s} {\gamma (1+ \frac v c \cos \theta_0) }(1) $$
here $\theta_0$ is the angle of reception. Angle of emission and angle of reception are tied by relativistic aberration formula.
$$ \cos {\theta_o} = \frac {\cos {\theta_s} + \frac v c} {1+\frac v c \cos \theta_s} $$
We can rewrite:
$$ f_0 = \gamma (1 – \frac {v \cos \theta_s} {c}) f_s (2)$$
If source was straight overhead when light pulse was received, that means that the source emitted pulse of light at right angle to direction of motion of the observer $\cos \theta_s = 0$ and we get
$$f_0= \gamma f_s$$
i.e. light will be blueshifted at reception.
Transverse Doppler Effect: https://en.wikipedia.org/wiki/Relativistic_Doppler_effect
The source (for example - illuminated sphere) will also appear rotated at relativistic aberration angle (Terrell-Penrose rotation)
This way, if the source is straight overhead (in zenith) but observer moves with velocity close to c, the source will appear to be "in the front of him on the horizon" and source's radiation will be blueshifted. The cause of these effects are aberration of light, contraction of observer's measuring ruler and dilation of observer's clock.

