This shows the motion of the particle and turntable at an instant in time. We assume that the system has settled down i.e. at the instant shown the particle is moving at the same velocity as the bit of the turntable immediately underneath it.
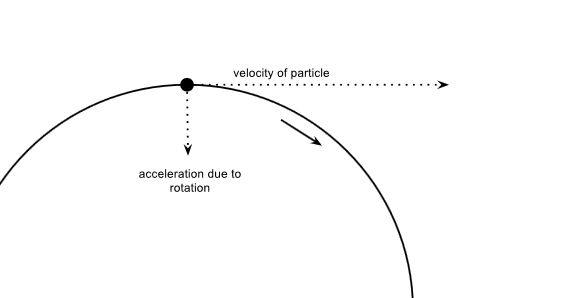
The particle's velocity is tangential to the turntable - I've indicated this by the dotted line. Because the turntable is rotating, the point on the turntable immediately under the particle is accelerating towards the centre at $a = r\omega^2$ where $r$ is the radius of the turntable and $\omega$ is the angular velocity.
If there were no friction the particle would travel in a straight line (along the dotted line) at constant velocity and we'd see the particle fly off the turntable.
If instead we see the particle stay in place on the turntable, there must be a force accelerating it, and that force must act in the direction of the acceleration i.e. towards the centre of the turntable. That force is of course the friction between the particle and the turntable, so the friction acts towards the centre of the turntable.
Update: Tanya asks what happens when a stationary particle is placed on the turntable.
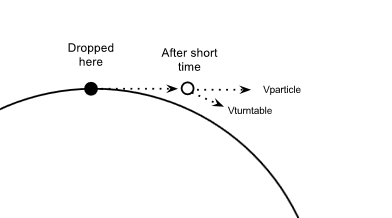
The particle is placed on the turntable where the black dot is. At this instant the particle is stationary with the turntable skidding underneath it. The frictional force acts in the direction of the relative velocity between the particle and turntable, so at this moment the frictional force is tangential.
The particle accelerates in response to the frictional force, so some very short time later it has moved to the position shown by the open circle. I'm assuming the time is short enough that we can assume the particle has moved in a straight line: I've exaggerated the particle motion on the diagram to make the argument clearer.
Anyhow, because the particle has moved in a direction tangential to the turntable the direction of the relative velocity between the particle and turntable has changed. That means there is now a component of the frictional force downwards as well as in the original direction. The result is that the particle is now accelerated downwards as well as to the right. If you watched the particle it would follow a spiral centred on the centre of the turntable until it reached the edge and flew off or came to rest relative to the turntable. In either case the net result is that there has been an acceleration towards the centre of the turntable i.e. there is a component of the fictional force acting towards the centre.


