Why do I not see evidence of discrete energy in the spectrum?

Question

The electromagnet spectrum looks to be continuous, yet energy is discrete. Shouldn't one see evidence of the electron's "quantum leap"? What's going on?

Moderator, please size my photo. Thank you.

Answer 1

Even before quantum mechanics spectral lines distinctive of elements were seen:

Gustav Kirchhoff, who proposed the study of blackbody radiation, made use of Bunsen's burner. In 1859, he announed that all the chemical elements he had studied each produced a distinct spectrum, which he could identify by passing the flame's light through a prism. The prism spread out the light, but instead of seeing a continuous rainbow, Kirchhoff observed a pattern of distinct lines. The pattern of lines was different for each element, and what's more, Kirchhoff saw that when light passed through a vapor of some element, the element absorbed the same colors as it emitted when it was heated. (This led to his interest in the blackbody spectrum.)

Theser are the disntictive electrons quantum leaps.

Kirchhoff realized that if a new rock sample were heated and its spectrum found to contain lines not characteristic of any known element, the sample must contain a new element, hitherto unknown. Using this technique, he discovered cesium a year later, in 1860. He named it after a Latin word meaning "sky blue", because a spectral line of that color gave away the new element's existence. A year after that, he discovered the element rubidium, which he named for the Latin word meaning "red".

Then came Maxwell's equations with their strict mathematics and black body radiation fitted with discrete electromagnetic energy levels.

You are confusing the discreteness of the energy packages which are the photons, with the possibility of creating any frequency photon , given enough sources. As there are 10^23 atoms in a mole of matter, and tens of common elements which can be excited and in deexcitation release a photon in a 4pi sphere all frequencies can appear and overlap . In addition accelerating charges can also emit all frequencies, which happens in the sun's plasma Light is an emergent effect from a confluence of zillions of photons. It is only with careful experiments that the spectra will appear, as with specific flames.

Absorption spectra also indicate discrete energy levels.

spectra

The bottom spectrum is the absorption spectrum of the sun, and those above it for galaxies progressively further away. The pattern of absorption lines shifts further and further to the right, toward the red end of the spectrum.

Fraunhofer lines are a result of gas in the photosphere, the outer region of the sun. The photosphere gas is colder than the inner regions and absorbs light emitted from those regions.

Answer 2

Particles in bound states exhibit quantized energy levels. This is a result of boundary conditions on the wave function. For instance, an electron in an infinite well must have 0 probability of being found outside the well or at the boundaries (to keep the wave function continuous). Solutions to the Shrodinger equation will look like this (as you can see, only a discrete set of energy values are allowed):

As another example, an electron orbiting a hydrogen nucleus should have 0 probability of being found infinitely far from the nucleus, so that the wave function is normalizable. The math for this one is more complicated, but you still get a discrete set of solutions to the Shrodinger equation, with discrete energies. Thus the electron can only be observed in these discrete states, and if you excite hydrogen gas, you will observe a discrete emission spectrum: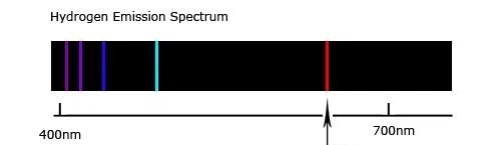

Free particles however, have no boundary restrictions on their wave functions, and may be observed to have any energy whatsoever. This is why you see a continuous spectrum from natural light.

(Note that it is technically still discrete, because each photon has a definite energy and there is a finite number of photons, so you cannot actually obtain every possible real number energy from them)