Ising model consists of up spin and down spin or empty/filled space. Can we model random walk for different densities of packing through the Ising model?
$\begingroup$
$\endgroup$
2
-
$\begingroup$ What do you mean by "Can we model random walk for different densities of packing through ising model"? $\endgroup$– Yvan VelenikCommented Mar 9, 2017 at 16:17
-
$\begingroup$ Hello Yvan , i am working on tipping points (phase transition) in ecological systems such as lake system using ising model. Here i am focusing on flow dynamics through vegetation .I want to simulate particle velocity using ising model for different packing of spins (vegetation ) .Hope , i made it clear $\endgroup$– CuriosityCommented Mar 9, 2017 at 23:14
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
There are ways to map the magnetization distribution problem of the Ising model to persistent random walks. The relevant references are:
R. Garcia-Pelayo, "Distribution of magnetization in the finite Ising chain," Journal of Mathematical Physics 50, 013301 (2009)
and
T. Antal, M. Droz, and Z. Racz, "Probability distribution of magnetization in the one-dimensional Ising model: effects of boundary conditions," Journal of Physics A: Mathematics and General 37, 1465 (2004)
$\begingroup$
$\endgroup$
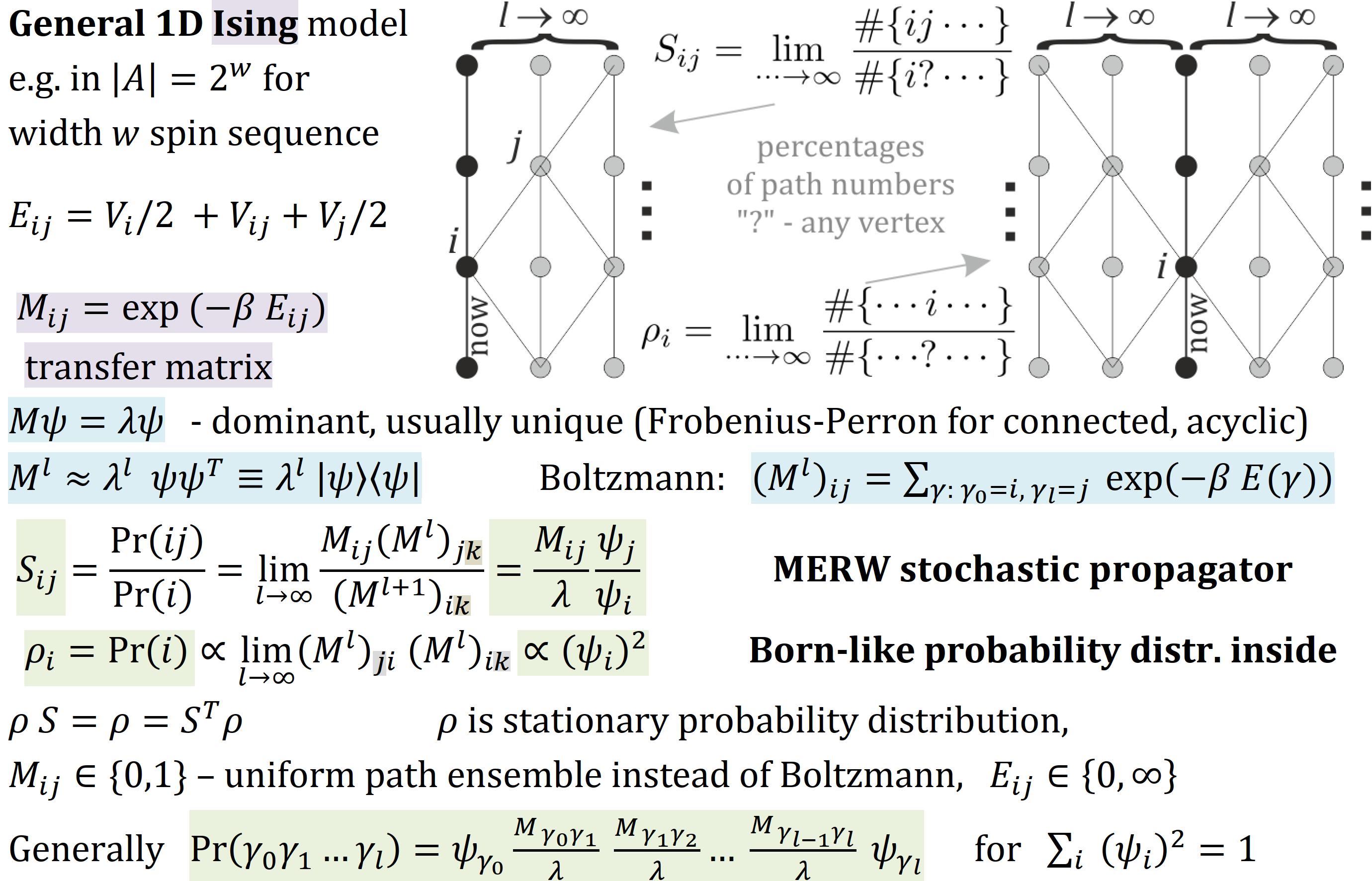
Yes, we can imagine going through 1D Ising model as random walk, analogous to Maximal Entropy Random Walk, e.g. to model 2D Ising $w\times \infty$ as 1D for width $w$ slices ( https://arxiv.org/pdf/1912.13300 ).
For $M_{ij}=\exp(-\beta E_{ij})$ transfer matrix, where $E_{ij}$ is energy of $i-j$ edge (e.g. negative for the same spins in ferromagnet), you need to find dominant eigenvector $M\psi = \lambda \psi$, then
stationary probability distribution is $Pr(i)\propto (\psi_i)^2$,
Stochastic matrix: $S_{ij}=Pr(x_t=j|x_{t-1}=i)= \frac{M_{ij}}{\lambda} \frac{\psi_j}{\psi_i}$.
Sketch of derivation:
