Why can we use the 2D projection of a 3D gaussian surface to calculate electric flux?

Question

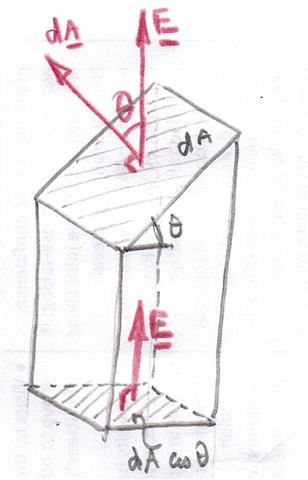
In order to calculate the electric flux passing through one side of a cone with no net charge enclosed, I originally thought you needed to take infinitesimal areas and dot the normal vector with the field vector and integrate. However, I found that you can take the 2D projection of the cone, find the area, and multiply it by the field to get flux. However, I'm a bit confused on why this works. The area of the 2D projection is different from the surface area of one half the cone, and Gauss's law has an integral of areas.

I have a feeling that the discrepancy of area is made up by the fact that the dot product between the vectors makes up for this difference. Does this have something to do with how vector projection ties into the dot product? I'm not super familiar with the deep intuition of it, but I'm curious why this discrepancy in area gives the same flux.

Answer 1

It is about how "many" field lines that pass through. These determine the Flux passing through, and they don't change when the object is drawn in 3d.

• Its like putting down a plane wired mesh into a water stream and counting water particles passing through.
• If you put down a half-sphere shaped wired mesh, you still count the same amount of particles passing over the same time. They just have more area per particle.

In the same way, a 3d object gives bigger total area but with "fewer" field lines per area. The corresponding cross section has smaller area and "more" lines per area. It turns out that the "amount" of lines is the same. Since cross section area is easier to calculate, that is what people do.

Answer 2

and Gauss's law has an integral of areas

Gauss has an integration of the dot product of the electric field and areas which is different.

From what you have written I assume that the electric field $\vec E$ is constant.

For a small element of area $d\vec A$ you need to evaluate the electric flux through that area $\vec E \cdot d\vec A$.

Evaluating the dot product gives $E\,dA\, \cos \theta$.
Now you will see from the diagram that $dA\, \cos \theta$ is just the projection of the area which is at right angles to the electric field.
Provided the electric field is constant you can perform the same projection over a surface and evaluate the total electric flux by multiplying the magnitude of the electric field by the total projected area.

If the electric field is not uniform then all the projected areas at right angles to the electric field will not be in the same plane.