$SU(2)_L$ Gauge theory and particle-antiparticle annihilation

Question

I have a problem understanding how to reconcile the particle antiparticle annihilation vertex with the $SU(2)$ gauge theory, in the context of the weak interaction. Let me explain better :

Invoking $SU(2)$ gauge invariance we deduce there must be three gauge bosons, associated to the three Pauli matrices. We take, as usual, the linear combination yielding $\sigma_+, \sigma_-$ and $\sigma_z$ that are respectively associated to $W^+, W^-$ and $Z$. I am aware that I should be considering $U(1)_Y\times SU(2)_L$, but in the context of this question I believe it is irrelevant.

Now consider the SU(2) doublets,$\begin{pmatrix}l^+\\ l^- \end{pmatrix}$, where $l^+$ has weak isospin $1/2$ and $l^-$ has isospin $-1/2$. Let's take $\begin{pmatrix}v_e\\ e^- \end{pmatrix}$, we find that the weak current by coupling to the $Z$ boson is: $$j^{\mu}_Z \propto \begin{pmatrix}\overline{v}_e & \overline{e}^- \end{pmatrix}\gamma^{\mu}\sigma_z \begin{pmatrix}v_e\\ e^- \end{pmatrix}$$ Where $\overline{u} = u^{\dagger}\gamma^0$. Expanding this, we find that : $$j^\mu_Z=\frac{1}{2}\overline{v}_e\gamma^{\mu}v_e-\frac{1}{2}\overline{e}^-\gamma^{\mu}e^-$$

Where, $v_e$ and $\overline{v_e}$ stands for the spinors of the neutrino, and likewise for the electron. As we can see from this, it seems that the Z-boson couples particles of same weak isospin. However, we can have an annihilation vertex where $e^-$ and $e^+$ annihilate into a Z boson, despite the fact that $e^-$ has $I_w^{(3)} = -1/2$ while $e^+$ has $I_w^{(3)} = 1/2$. How can this reconciled with the representation of Z as $\sigma_z$ ?

I know that there is some problem with my current, since obviously an $e^-$ cannot annihilate with an $e^-$, in a vertex such as : , but only in a vertex such as : .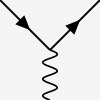

However, in my derivation, there does not seem to be a distinction in which one of these vertex I'm considering, so I'm confident that there lies my mistake, but I am unable to figure it out. I think somehow, in an annihilation vertex, particles of opposite weak isospin should interact while in a scattering vertex particle of same weak isospin should interact. This is also consistent with conservation of weak isospin, but I am unable to understand how to make this distinction in the currents using $\sigma_Z$ as the Z boson coupling.

Answer 1

How can this reconciled with the representation of $Z$ as $\sigma_{z}$?

Don't confuse: the isospin $T$ is not the isospin projection $T_{3}$. The $Z$ and $W^{\pm}$-bosons, as well as fermions, correspond to the definite isospin projections. The difference is that the fermions are in the fundamental 2-dimensional representation of the $SU(2)$ group (the so-called $2$ representation), with isospin projections $\frac{1}{2}, -\frac{1}{2}$, while $W^{\pm}, Z$-bosons are in adjoint 3-dimensional representation (the so-called $3$ representation), with isospin projections $1,0,-1$. The isospin projection $T_{3}$ of the fundamental representation (say, just for electron) is defined as $$ \frac{1}{2}\sigma_{3}\begin{pmatrix} 0 \\ e\end{pmatrix} = -\frac{1}{2}\begin{pmatrix} 0 \\ e\end{pmatrix} \equiv T_{3}\begin{pmatrix} 0 \\ e\end{pmatrix} $$ The isospin projection of weak bosons is determined in a way $$ [\sigma_{3}, \sigma_{i}] \equiv T_{3}\sigma_{i}, $$ where $\sigma_{i}$ is the generator associated with the given boson; see also the question. For $Z-$boson, $i = 3$, and $T_{3} = 0$; for linear combinations of $W_{1,2}$ bosons, namely, $W_{+} = W_{1} - iW_{2}$, $W_{-} = W_{1} + iW_{2}$, the projections are $T_{3} = + 1, T_{3} = - 1$ correspondingly.

Therefore the operator $\bar{e}\gamma^{\mu}(c_{V} - c_{A}\gamma_{5})eZ_{\mu}$ has zero total isospin projection.

Answer 2

The main point is that the barred fermions have "opposite charges", or, for non-Abelian groups, they transform in the conjugate representation.

Charges (e.g. electric) or other gauge quantum numbers such as weak isospin determine how a field transforms under the gauge group. If the group generators are $T_i$ (in some representation), an unbarred fermion (or any field in that representation) transforms as $$ \psi \longrightarrow e^{\text{i}\alpha_i T_i} \psi\,,$$ where $\alpha_i$ are some (real) parameters. This expression includes the convention that the generators are Hermitean ($T^\dagger=T$), which is common in physics, and requires a factor of $\text{i}$ in the exponent to make the group element unitary. (In some mathematical contexts, the convention is to use anti-Hermitean generators, and no $\text{i}$ consequently.)

This implies that the barred fermion, which involves a complex conjugation, transforms as $$ \bar\psi \longrightarrow \bar\psi \, e^{-\text{i}\alpha_i T_i} \,.$$ Hence, in particular they have opposite "charges" under the (diagonal) Cartan generators, in your case $\sigma_3$. For $U(1)$ groups, the charges are indeed opposite, without scare quotes -- otherwise you should also wonder why the $Z$ couples to particles with electric charge $-1$!

Note that the same thing shappens for the kinetic term, which in your notation looks like $$\frac{1}{2}\overline{e}^-\gamma^{\mu}\partial_\mu e^-$$ and of course still is a singlet, ie.e has zero isospin.