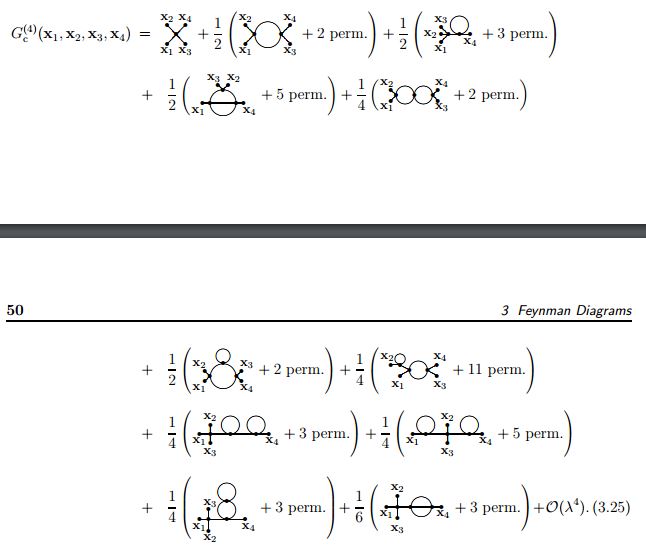
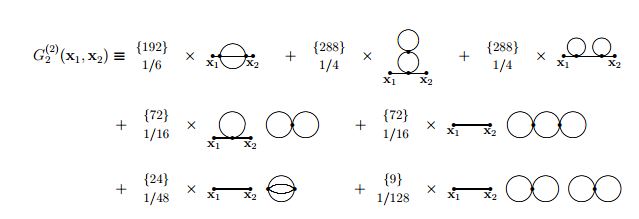I'm considering $\phi^4$ theory with the action $S[\phi] = S_{\mathrm{FREE}}[\phi] + \frac{g}{4!} \int d^{4}x \ \phi(x)^4$.
I'm supposed to come up with the Feynman diagrams up to order $g^2$ for the 2-point and 4-point correlation functions.
It's not necessarily hard, it's just extremely tedious and I'm worried I'm missing some diagrams (I think there are 21 diagrams at order $g^2$ for the four-point function, for example. This is headache to make sure I've got everything down correctly)
My question is, is there a resource that lists off the Feynman diagrams up to order $g^2$? It's easy for me to find up to order $g^1$ (but not higher than that).