To start addressing this question I will first say that while I think that string theory has something to do with fundamental physics I do not particularly see it as all (strings uber alles), but as something which may reflect a pattern in the structure of reality. The M-theory aspects of string theory in AdS/CFT correspondence has been observed in condensed matter physics. It think string theory may be wrapped in with physics of quantum phase transitions, quantum critical points, and symmetry protected topological phases.
The string in a classical sense may be seen as a rod moving through space. It is then a body moving through space with position $X^\mu$ with a momentum $p^\mu$ with
$$
X^\mu(\sigma,~\tau)~=~X_0^\mu(\sigma)~+~p^\mu\tau
$$
where $\sigma$ parameterizes the string extension and $\tau$ parameterizes the time. This is the “classical” string or rod, which is the quantum ground state as well. To make this into a quantized system we need to add quantum oscillation terms so that
$$
X^\mu(\sigma,~\tau)~=~X_0^\mu(\sigma)~+~p^\mu\tau~+~\sum_{n=0}^\infty (a_nf(\sigma,\tau)~+~\bar a_nf^*(\sigma,\tau)).
$$
Here $a_n$ and $\bar a_n~=~a_{-n}$ are lowering and raising operators of harmonic oscillator states and $f(\sigma,\tau)$ are oscillatory functions. The nature of these depend upon whether the string is open or closed in a loop.
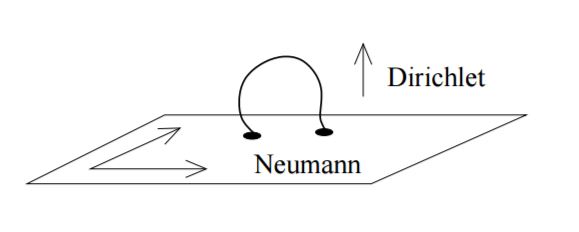
The closed string has two oscillation modes in opposite directions along the string. These independent modes for spin $1$ states can form a spin $2$ state corresponding to a graviton. The open string has ends that when unattached to anything anchoring an end point have Newman boundary conditions for reflected waves. When they are attached the boundary conditions are Dirichlet. An open string can have one end anchored, usually two what is called a $D-brane$ and the other unanchored with mixed boundary conditions like an organ pipe or a wave in a quarter wave stack. These waves correspond to spin $1$ states. Some of these may have supersymmetry correspondence with fermion states for leptons and quarks. The supersymmetric pairs of gauge bosons are the gluinos, photinos etc.
There are two ways we might consider the particle correspondence to strings. I will write first about ordinary particles and get to gravitons at the end here. The standard idea is that an elementary particle such as a quark or lepton, spin $1/2$, or a gauge boson with spin $1$ is that the open string is tied to a D-brane, often thought of as a $D-3$-brane corresponding to space or a $D4$-brane corresponding to spacetime. A diagram illustrates this idea
Which illustrates string connected to a single D-brane, connected to two and closed strings that can move between the through D-branes. I lifted this diagram from David Tong's website. Often we think of the closed string attached to a single D-brane as an elementary particle.
There are some very important experiments to keep track of. These experiments involve the measurement of the electron magnetic moment. The $g$-factor meansred is $g~=~2.0023\dots$ has been measured to an accuracy of $10^{-13}$. This permits us to observe whether the electron has a field configuration that deviates from a point charge. So far the electron appears to be point-like down to $10^{25}cm$, which is $7$ orders of magnitude within the string length. Keep tabs on these developments, for it might illustrate the electron is point-like to scales smaller than a string!
What happens if these experiments find there is no deviation from a point-like particle configuration of the electron? Does this kill string theory? Not necessarily, but it does change how we model things with strings. To see this we consider S and T duality. S duality comes from the very early observation Dirac made about monopoles. A solenoid that is semi-finite in length will have at its opening a magnetic field that appears monopole like. We however want to make the solenoid disappear. How do we do that? We think of the solenoid with a vector potential $\vec A$ so that the Aharanov-Bohn effect induces a phase
$$
\phi~=~\frac{e}{\hbar}\oint\vec A\cdot dr,
$$
on the quantum wave $\psi~\rightarrow~e^{i\phi}\psi$. If the phase is $0,~2\pi,~\dots,~2n\pi$ the the solenoid is made to disappear. From Stokes' rule we then have
$$
\phi~=~\frac{e}{\hbar}\oint\vec A\cdot d\vec r~=~\frac{e}{\hbar}\int\int\nabla\times\vec A\cdot d\vec a
$$
$$
\frac{e}{\hbar}\int\int\vec B\cdot d\vec a~=~\frac{e}{\hbar}g~=~ 2n\pi,
$$
where the last step is basic Gauss' law and $g$ is the magnetic monopole charge. We then see the charge quantization condition $eg~=~\hbar 2n\pi$. So we may then think of an elementary particle as not an open string on a single brane but as connected to two D-branes. Further, the charge associated with this string open on a brane is similar to that from the Dirac solenoid and is then by duality what is called a $D1$-brane. This is like a string, but is more massive and presents its quantum number on a D-brane. An elementary particle may then turn out to be of this nature, where what we observe is just of open end of the $D1$-brane.
Briefly there is also T-duality, which exchanges the momentum of a string with modes $n$, say $p~=~n/R$ for $R$ the radius of a closed string, with its winding number on a brane with some radius $R$ so that $n/R~\leftrightarrow~wR$ is a dual symmetry. With S-duality there is a general set of transformations called STU, which are important for entanglement symmetries on black holes.
The graviton is a bit mercurial. As a closed string it is not tied to a D-brane by open ends. It may however be wrapped on a D-brane with T-duality. How a closed string remains tied to a D-brane is a bit mysterious, and if closed strings can fly across branes then in principle gravitons can carry mass-energy away from our observable universe.
So keep looking at results and be of flexible mind.

