Using Ampère's on balloon shape

Question

In an assignment I'm looking at for exam preparation I am asked to use Maxwell's corrected version of Ampère's law (the integral form) $$\oint_{loop}\vec{B}\cdot d\vec{l} = \mu_0 \left(I_{encl} + \epsilon_0 \frac{d}{dt} \int_{surface} \vec{E} \cdot d\vec{a}\right)$$
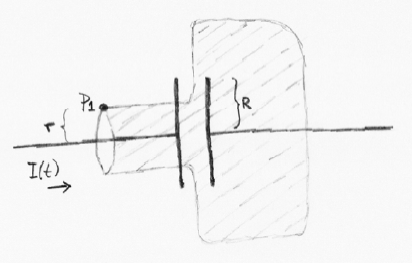
to find the magnetic field in the point $p_1$ in the distance $r<R$ above the wire. There is a current, $I(t)$, running through the wire, the capacitor is circular and has a radius $R$, and the loop is a circle around the wire.

I have to calculate it using this complicated balloon shape given (see the figure below), which I have some troubles with.

What I'm thinking is that the wire is piercing the balloon three different places - to the far right and at the capacitor twice. The piercing on the far right would contribute with $\mu_0I$ from the first part of the equation, but I'm not sure what to the with the other two.

Then comes the surface integral of the $\vec{E}$ field, which I'm not sure how to incorporate either.

Can you come with some tips or explanation that could help me better understand how to use the law on the surface?

Answer 1

The left hand side is straight forward?

The $I_{\rm encl}$ term you have to be careful with because although a current $I$ enters on the left hand side of amperian surface there are two places where it is leaving.
One is the right hand area of the amperian surface but there is also some leaving at the left hand plate as the outside the amperian surface part of the plate has to be charged up.
So in effect the current term is found by relating it to the ratio if the areas $\frac{\pi r^2}{\pi R^2}$.
If you are unsure about this just reverse the current direction with a current going into the right hand side of the amperian surface how much current actually gets out through the orifice of the amperian surface with the rest passing through the amperian surface to charge the capacitor plate outside the amperian surface?

For the second term on the right hand side you are only looking to integrate between $r$ and $R$ as that is the only part of your amperian surface where the electric file is perpendicular to the amperian surface.
Assuming an ideal capacitor you can relate the electric field between the plates to the surface charge density $(=\frac{\rm charge}{area})$ on the plates.
Differentiating the charge with respect to the time will give you the current.