Is it always true, for any cycle followed by a gas, that, if the cycle is "travelled" clockwise in the $P-V$ plane then the work exchanged by the gas is positive, and viceversa for the clockwise direction?
I came up with this situation, which I represented in the picture. The irreversible cycle is made of an isothermal compression, and two adiabatics. One of the adiabatics must be irreversible for the cycle to exists.
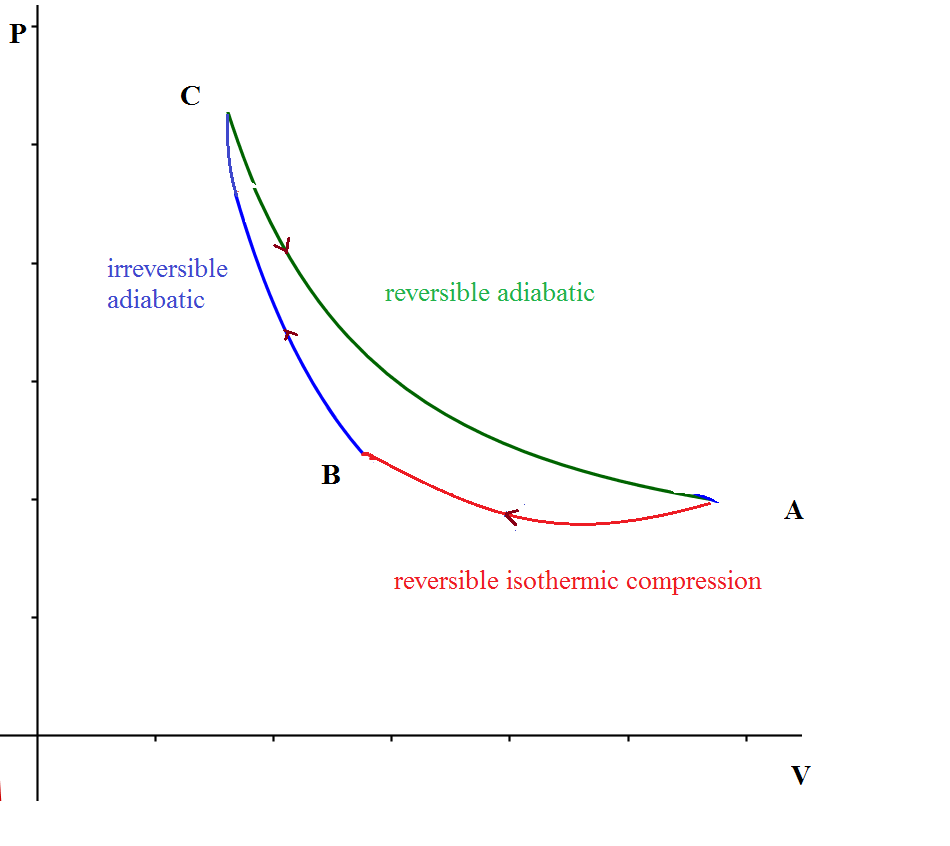 The cycle seems possible to me because since $$\Delta S_{universe}=\Delta S_{environment}=\Delta S_{environment, A->B}>0$$
The cycle seems possible to me because since $$\Delta S_{universe}=\Delta S_{environment}=\Delta S_{environment, A->B}>0$$
This cycle is clockwise, but $$Q_{cycle}=W_{cycle}=Q_{A->B}<0$$
So this appears in contrast with the rule $$\mathrm{clockwise} \implies W>0$$ $$\mathrm{anticlockwise} \implies W<0$$
Is that possible or am I missing something?
Sign convention: