Dipole Moment and separation

Question

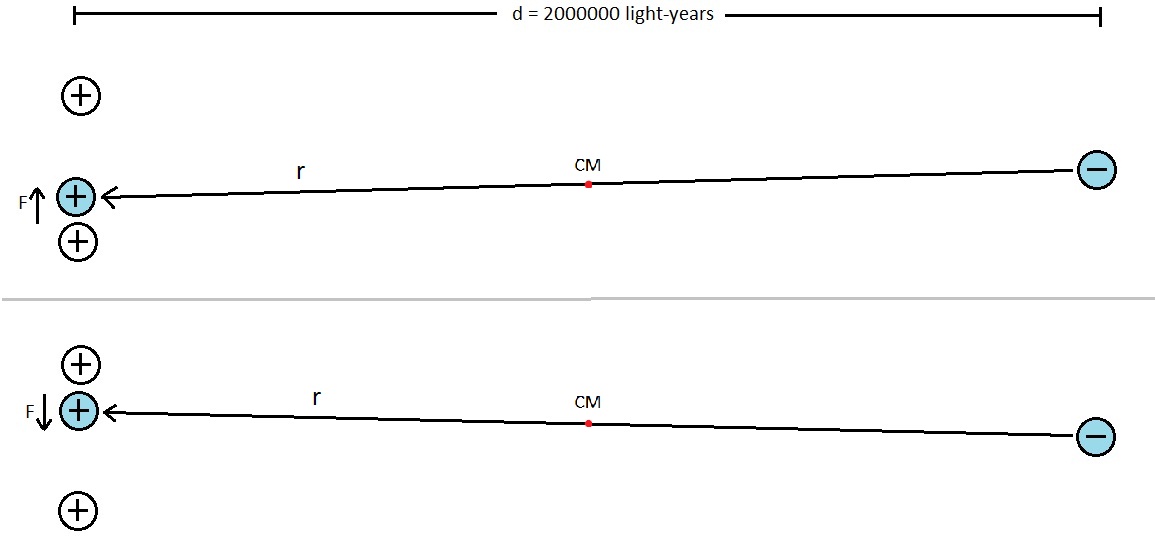
My understanding of a dipole moment is it defines the strength of various dipole interactions, eg the common example of torque in an electric field. My confusion stems from the fact that the magnitude of the dipole moment is proportional to the charge separation. In many areas of physics, we deal with quantities that are inversely proportional to distance. I can reason very intuitively that as I move further from something, our mutual interaction is reduced. The idea that in a dipole, the further you remove something, the stronger the interaction becomes is equally counter-intuitive to me.

For example, if I removed two charges to infinity from each other, their dipole moment is infinity, and they are the "strongest" dipole possible. In fact, why don't massive dipole moments exist between charged particles on earth and charge particles on some star light-years away. With such a large dipole moment, even the smallest electric field would result in an unimaginable torque on our charged particles. I know particle charges are distributed on a macroscopic scale very finely so as to be overall neutral, but there must be even to a minuscule order some small imbalance that would evidence these incredible torques.

I suppose I think of the dipole moment as some kind of force, or at least proportional to a force, and I am having trouble understanding how a force's effect can increase with distance.

Please help me understand this better.

Answer 1

The idea that in a dipole, the further you remove something, the stronger the interaction becomes is equally counter-intuitive to me.

The dipole moment does not define an 'interaction'. You can just interpret it to be a useful mathematical quantity - nothing more, nothing less.

For example, if I removed two charges to infinity from each other, their dipole moment is infinity, and they are the "strongest" dipole possible. In fact, why don't massive dipole moments exist between charged particles on earth and charge particles on some star light-years away. ...

The crucial point you're missing is that in order for those two-opposite-charges-in-separate-galaxies to generate a torque (and therefore have any measurable effect), they need to be connected to each other. Indeed, electric dipole moments are typically only ever useful when considering molecular scale processes whereby opposite charges may be constrained by intra-molecular bonds.

Answer 2

Indeed, there must be dipole moments of such magnitude occurring (if you just base it on the definition)! But usually, there is no extensive electric field to support the continuous rotation of the dipoles, because the net electric field in space is zero. Though there will occur some abrupt torque (again, based on definition) of intense magnitude due to the interaction of the dipole charges to the electric field of other charges, the torque will quickly reverse direction alternately, and the average torque will become zero.
The blue-colored is the dipole charges.
At the left side of the universe, a charge from the dipole interacts with other charges, jiggling it back and forth.
In this case, $T = r \times F$.
But because they're not connected, this torque has little to no effect on the position of the dipole charge at the right side of the universe. Only the relative position with each other has changed.

Answer 3

As per my understanding of dipoles : When we talk about dipole we usually think of a charge pair of +q-- -q separated by a distance of 2d. But we are much far away from the dipole. i.e. 2d << D where D is the distance of observer from dipole. If this condition do not hold the charge dumbbell can not be treated as dipole. You have to treat them as individual charges.

Single charge 'q' will produce a much larger field when seen from distance 'D' compared to dipole because in dipole the field of positive charge is almost cancelled by the negative charge. What you see is just the residual field. As you increase the distance between the two charges this cancellation reduce which in turn results in increased dipole moment.

I hope this will resolve your doubts.