1
$\begingroup$

A) Is there a formula for the phase difference between the electric and magnetic field oscillations, in vacuum, in an electromagnetic wave emitted from an antenna, as a function of the frequency the distance from the antenna?

B) Does the formula depend on the antenna type and on the direction of the radiation?

Improve this question
$\endgroup$
1

1 Answer 1

Reset to default
1
$\begingroup$

The electric and magnetic fields are always in-phase if the wave can be treated as a plane wave (which simply means it cannot be too close to the source), and in vacuum or any medium with linear response, such as air.

Boundary conditions of wave guides change this relationship, and must be solved for each specific case. If the wave guide is large enough, you will only see effects near the surfaces.

Transmission through a conductor results in phase-lag of the magnetic field, and a rapid extinction of the propagating wave; see skin depth.

The methods used are nicely described here: Chapter 9: Electromagnetic Waves - MIT OpenCourseWare

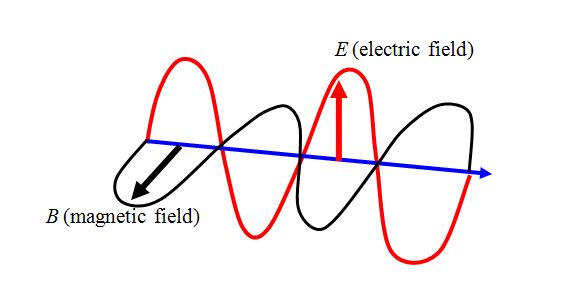Electromagnetic plane wave: electric and magnetic fields are always in phase. Electromagnetic plane wave: electric and magnetic fields are always in phase.

You can study the mathematical proof, based on Maxwell's equations for an arbitrary plane wave, here.

So the direct answers are: (A) No, except that they are always in phase in the "far field"; (B) No, see (A).

In the near field, for a dipole antenna, see Chapter 10: Antennas and Radiation - MIT OpenCourseWare, especially section 10.2.

This is why you ordinarily only need to consider the electric field with radio or light transmission, except in a wave guide, or non-linear media.

Occasionally somebody claims that the electric and magnetic fields are out of phase for circular polarization. This is not quite correct: the quarter wave plate accepts linearly polarized light at some angle wrt the fast axis of the QWP; the orthogonal components of the electric field vector under go different amounts of optical delay, resulting in two distinct electromagnetic waves, with the same phase delay in their electric and magnetic fields. See this animation of circular polarization; the input light is linearly polarized, with the red and blue representing the two components wrt the fast axis of the quarter wave plate, QWP.

Improve this answer
$\endgroup$
5
  • $\begingroup$ I am not following your argument about electric and magnetic fields being in phase for an electromagnetic plane wave. They must be out of phase according to Maxwell's equations (e.g., $\nabla \times \mathbf{E} = -\partial_{t} \mathbf{B}$), unless you mean something different when referring to phase. If both are linear, they oscillate along orthogonal directions to each other and to the wavevector. But they could reach their maximum at the same phase... Is that to what you refer? $\endgroup$
    – honeste_vivere
    Commented May 28, 2016 at 16:42
  • 1
    $\begingroup$ I'm interested exactly in the transition between close-field and far-field. Would there be such a formula if I concentrated specifically on a half-wave dipole antenna? $\endgroup$
    – Lior
    Commented May 28, 2016 at 17:28
  • 1
    $\begingroup$ @honeste_vivere: Yes, that is exactly what is meant; see illustration. The proof can be seen by following the link; the equation given, the Maxwell-Faraday form of Faraday's Law of Induction directly relates spatial and temporal aspects of the fields; when one goes through the details, for plane waves, the fields are in phase. $\endgroup$
    – Peter Diehr
    Commented May 28, 2016 at 17:35
  • $\begingroup$ @Lior: See additional link to Chapter 10: Antennas and Radiation - MIT OpenCourseWare, section 10.2. Equations are provided for a specific case, 10.2.2 Near fields of a Hertzian dipole, on p. 310. $\endgroup$
    – Peter Diehr
    Commented May 28, 2016 at 17:41
  • $\begingroup$ What about in a conductor. They are always off by 45-deg. Why different than vacuum $\endgroup$
    – Al Brown
    Commented Aug 20, 2021 at 6:36

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.