I realize this must have been considered but please explain what I am missing. My understanding is that the Equivalence Principal states essentially that someone in a closed cabin accelerating at 9.8 m/s can't tell whether he is in a gravitational field or is in fact accelerating. But wouldn't it be true that the no matter how far one was in the cabin from its "floor" the acceleration would be the same whereas in a gravitational field, if you are high enough, the acceleration would be less. So at least in this respect, the two situations would appear not to be equivalent.
$\begingroup$
$\endgroup$
6
-
2$\begingroup$ You are missing that this is a local equivalence. Nowhere does the equivalence principle state that is has to be valid anywhere but in a sufficiently small volume element. $\endgroup$– CuriousOneCommented Apr 25, 2016 at 20:35
-
2$\begingroup$ Someone will explain this in more detail in a proper answer, but the idea is this: the equivalence principle only applies in small regions of spacetime. In other words, only at a single "point" -- if you can imagine that. $\endgroup$– user113914Commented Apr 25, 2016 at 20:37
-
$\begingroup$ So is the important idea not that the astronaut can't perform an experiment that shows him he is in a gravitational field or not? $\endgroup$– JeffCommented Apr 25, 2016 at 20:40
-
$\begingroup$ Depends on how big the astronaut is. The folks on the ISS can tell easily that they are in LEO and not in completely free space because the tidal forces, which are the leading order deviations from the local prediction of the equivalence principle, are actually quite large and so is the drag of the ISS in the upper atmosphere. As a zero g laboratory, which is an often advertised selling point of the ISS, it kind of sucks in one direction and blows in the other. :-) $\endgroup$– CuriousOneCommented Apr 25, 2016 at 20:58
-
$\begingroup$ Jeff, if you are off the floor of your cabin, there would seem to be nothing to provide the normal force that it would take to accelerate you. Does your argument not "fall apart" if this condition applies? $\endgroup$– David WhiteCommented Apr 25, 2016 at 23:23
|
Show 1 more comment
1 Answer
$\begingroup$
$\endgroup$
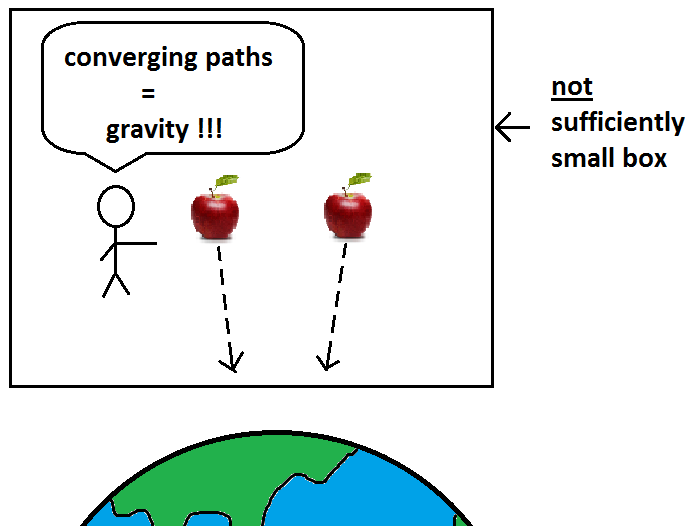
The equivalence principle is local (as the comments indicate), only applicable for sufficiently small regions. Another example that highlights the necessity of the word "local" is the following picture, in which the person is able to distinguish between linear acceleration and gravity.