How to include temperature effect in optical bloch equations (optical pumping)?

Question

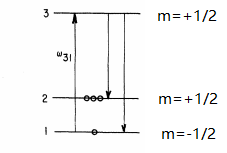
My problem is about the optical pumping of Alkali atoms by circularly polarized pump light. Consider a circular polarized light ($\Delta m=+1$) $$\vec{E}(z,t)= \vec{E}^{(+)}_0 e^{-i\nu t}+c.c. $$ incident on a $\Lambda$-type atom (shown below). The optical bloch equation (OBE) $\dot{\rho}=[H_0+V,\rho]/(i\hbar)-1/2\{\Gamma,\rho\}$ (where $V=-e \vec{r}\cdot \vec{E}$) from standard textbooks is

$$\dot{\rho}_{11}=\frac{1}{i\hbar}(V_{13}\rho_{31}-\rho_{13} V_{31})+\gamma_{31}\rho_{33},$$ $$\dot{\rho}_{22}=\gamma_{32}\rho_{33}, $$ $$\dot{\rho}_{33}=-\frac{1}{i\hbar}(V_{13}\rho_{31}-\rho_{13} V_{31})-\gamma \rho_{33},$$ $$\dot{\rho}_{31}=-(i\omega_{31}+\gamma/2)\rho_{31}+i(\rho_{33}-\rho_{11})\frac{V_{31}}{\hbar}.$$ From the last equation we get the solution for $\rho_{31}$: $$\rho_{31}=\frac{(\rho_{33}-\rho_{11})V_{31}}{\omega_{31}-\nu-\frac{i\gamma}{2}}$$ where $\gamma=\gamma_{31}+\gamma_{32}$. Inserting this into the other equations and finding static solutions $\dot{\rho}_{11}=\dot{\rho}_{22}=\dot{\rho}_{33}=0$ results in $\rho_{22}=1, \rho_{11}=\rho_{33}=0$. We immediately see the problem: the atomic ensemble is completely pumped into the $m=+1/2$ ground state even when the pump light is very weak (as long as it's nonzero!!).

That's quite ridiculous. We know from intuition that when the pump light is sufficiently weak, the atoms are distributed according to Boltzmann law $$\rho=e^{-\beta H_0}/Z$$. Seems that we need to include other terms in OBE so that its solution could reproduce Boltzmann law at weak field limit. How to do this?

Note: Yeah, at room temperature and optical frequency the Boltzmann distribution gives approximately $\rho_{11}=\rho_{22}=1/2, \rho_{33}=0$ since $\Delta E_{Zeeman}\ll kT\ll \hbar \omega_{31}$, which should have been the static-state solution of the OBE in the weak pump light limit. But my model fails, because, as I learned later, that I should have included in the first two equations of OBE terms that account for ground state relaxation due to collisions between the atoms and the wall. Pumping light tends to pump the atoms to $\rho_{22}$, while collisionoal relaxations tends to erase the population difference between $\rho_{22}$ and $\rho_{11}$. It is their competition that determines the static state population. My current trouble is that I don't know whether someone has seriously considered about the collisional relaxations of Alkali-atoms in any theoretical model of optical pumping?

Answer 1

First, I don't think the result, $\rho_{22}=1, \rho_{33}=\rho_{11}=0$, is ridiculous given the way how you modeled the $\Lambda-$type atom. Basically, the ground level "2" of $m=+\frac{1}{2}$ has no leakage of the energy. As the weak light keeps pumping the energy into the system, a portion of it keeps being accumulated to the ground level "2". This is seen from the second equation $\dot{\rho}_{22}=\gamma_{32}\rho_{33}$. Therefore it is a correct result derived from the ansatz. Otherwise (at least) an additional leakage term needs to be added in the second Bloch equation to prevent this effect:

$\dot{\rho}_{22}= -\kappa\rho_{22} + \gamma_{32}\rho_{33}$

Secondly, to involve the thermal equilibrium effect in the Bloch equations, you have to link the spontaneous decay rate $\gamma$, $\kappa$ and the pumping rate $|V_{13}|$ through the thermodynamical ansatz, namely, the Boltzmann's law.