1
$\begingroup$

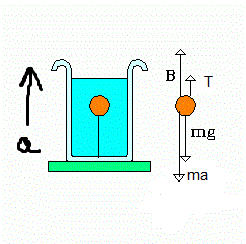
I can't understand why buoyant force exerted by the liquid on a ball attached to a rope in a box having liquid of density $p$ moving in an elevator moving with acceleration $a$ has buoyant force as,

$B= Vp(g+a)$ (V is the volume immersed ,i.e. the volume of ball itself)

Why its not $B=Vpg$?

Please try explaining mathematically/by using equations please.

enter image description here

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ If the elevator accelerates upward, the formula should have g+a in it. The force would be greater than from gravity alone. g-a is for a downward acceleration $\endgroup$
    – mmesser314
    Commented Dec 26, 2015 at 15:18
  • $\begingroup$ Related demonstration: Buoyancy Paradox - University of Maryland $\endgroup$
    – Vishnu
    Commented May 19, 2020 at 9:54

5 Answers 5

Reset to default
2
$\begingroup$

This becomes clearer if you think about the liquid, not the ball.

The buoyant force is caused by the pressure of the liquid. The pressure is caused by the weight of the liquid. The weight is cause by gravity.

When an elevator accelerates downward, it is just as if gravity had become weaker. The weight of the fluid decreases. The pressure decreases. The buoyant force decreases.

This question may also help. How does buoyancy work?

Improve this answer
$\endgroup$
1
$\begingroup$

Ask yourself why it was $pVg$ in case of non accelerated (inertial) frame. Because the pressure gradient is $pg$ or $p$ times the acceleration due to the force that is pulling it downwards.

Now in an accelerated frame, the pressure gradient is not really $pg$ but $p(g+a)$.

If you had to write pressure at a depth $d$, would you write $pVg$ or $pV (g+a)$?

Improve this answer
$\endgroup$
0
$\begingroup$

If the elevator goes up the water might concave slightly at the surface but remain in constant state because of the box it is contained by.If the elevator goes down the water can not move due to the suction pressure to the bottom of the box,and the pressure remains the same and the state is unchanged.So the medium of water did not deiferated enough to show drastic movement of the ball.

Improve this answer
$\endgroup$
0
$\begingroup$

Since the water is accelerating, the pressure distribution within the water must change to accommodate Newton's 2nd law locally. The net result of this is that the vertical pressure gradient becomes $\rho (g+a)$ rather than $\rho g$. It is as if gravity had increased.

Improve this answer
$\endgroup$
0
$\begingroup$

We find the pressure at some depth of any such accelerating column of water. The mass of some water is ρV. The net force on the water is ma+mg=ρVa+ρVg= ρV(a+g) because the force must not only "balance" the weight but it must also "push up" the water to result in a net force of ma upwards. Pressure will then be ρV(a+g)/A=ρh(a+g). Buoyant force is the difference in pressure between the top and bottom of an object. Hence, the buoyant force is ρh(a+g)*A=ρV(a+g)

Thus, the buoyant force=ρV(a+g). This is as if the gravity had been increased by a.

Improve this answer
$\endgroup$
1
  • $\begingroup$ You write "Buoyant force is the difference in pressure between the top and bottom of an object." Do you mean "Buoyant force is the difference in net force between the top and bottom of an object."? $\endgroup$
    – Tony
    Commented Jan 8, 2023 at 5:09

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.