I'm guessing you're all familiar with the classic intuitive way of explaining time dilation: with a light clock traveling at velocity v directed at a parallel direction to the mirrors that make up the light clock.
Now, what happens if we have a light clock that goes upwards at v? That is to say, what if its velocity is in a direction perpendicular to the mirrors' orientations? As far as I can tell, this situation wouldn't present any time dilation. Thoughts?
In that case, time dilation still occur, of course. In order to show this using t=d/v, you'd have to take into account the space contraction in the direction of motion. Mathematically, if d is the height of the clock, then the time taken from a photon at the bottom to reach the top of the clock isn't $\frac{d+vt}{c}$ but $ \frac{d/\gamma+vt}{ c}$. When you calculate the time taken for that photon to get back to the bottom of the clock and add it up to the time previously calculated, you get the exact same time dilation than the clock moving parallelly to the mirrors.
Time dilation would occur in this scenario.
This diagram depicts two light clocks. The left light clock is stationary. The right light clock is traveling to the right at 60% of the speed of light.
The green and red lines represent the mirrors of each light clock.
The blue lines represent photons bouncing between the mirrors of each light clock. All 45 degree lines represent the speed of light. The numbers indicate ticks of the light clocks, which are counted when the photon strikes a clock's left mirror.
The right light clock ticks slower because half of the time, its right mirror is moving away from the photon, which takes longer to catch up with it.
After 5 ticks of the left light clock, the right light clock has recorded only 4 ticks, indicating that time dilation has taken place.
yes, time dilation still occurs. The reason is that the mirrors are there to provide an intuitive view of how/why time dilation occurs, not to to create it. This time dilations still occurs regardless of the existence of the mirrors or the direction of motion. But the drawing will no longer have explanatory power.
The two parallel mirrors, A and B, are traveling together such that the axis of reflection is parallel to the direction of travel, and in such a direction that A trails behind B as both travel at the same velocity near the speed of light relative to our observatory. A laser attached to A fires a pulse at B which returns at some time $x/c$ later. Because B is moving rapidly away from the initial pulse location, it takes much longer for the pulse to reach B and reflect back in our frame than it does in the clock frame: time dilation still occurs.
What this arrangement introduces in addition to the textbook example (with the axis of reflection perpendicular to the axis of motion) is the relativity of simultaneity: in the textbook example, if a laser is mounted on each mirror and both fire at the same time, then both beams reach a sensor on the other mirror at the same time as well. In your example, the beam from B will reach A long before the beam from A reaches B, from our observational perspective; though in the frame of the ship carrying the light clock, they will still happen simultaneously.
By A and B I am referring to the two mirrors. Mirror A is the rearmost mirror; Mirror B is the foremost. As you say, the distance is increased when the light travels from A to B, but is reduced by the same amount when travelling from B to A. Thus (ignoring, for a moment, the Fitzgerald contraction) the total path length for a complete cycle remains the same as for a stationary system.
Acceleration does not come into it. The light clock thought experiment is a demonstration of Special Relativity, in which the clock and the observer are travelling at constant relative speed.
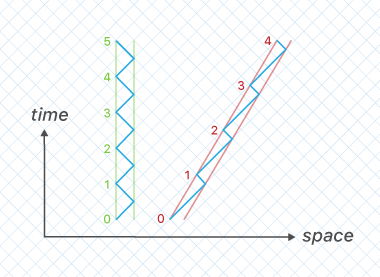
If we imagine that clock is rotated 90 degrees from the textbook example, and now the clock light travels back and forth on the same axis that object is moving. For a stationary clock, time needed for a photon to go from one mirror and back would be:
τ(stat)=2*d0/c
For a moving clock and a stationary observer, we first know that photon must go over distance d, increased by the distance that mirror 2 has traveled:
c*t1 = d + v*t1
Notice that we're assuming that distances d are not equal for both cases. Then it must go back, but this time the distance it has to go will be reduced by the amount that mirror 1 travels in it's direction:
c*t2 = d - v*t2
Total amount of time needed for photon to go from mirror 1 to mirror 2 and back would be:
τ(mov)= t1 + t2
= d/(c-v) + d/(c+v)
=2cd/(c^2 - v^2)
Now, the distance d here is not the same as distance d0 that we have in the first equation, because we have the effect of length contraction. Motion distance will be:
d=d0/γ
d=τ(stat)*c/(2*γ)
Where we know:
γ^2= c^2/(c^2 - v^2)
If we replace that in our equation, we get:
τ(mov)= [2*c*d]/(c^2 - v^2)
= [2*c*τ(stat)*c/(2*γ)]/(c^2 - v^2)
= τ(stat)*c^2/[γ*(c^2 - v^2)]
= τ(stat)*γ^2/γ
= τ(stat)*γ
This should be a time dilation formula for longitudinal light clock.
The question describes the "Longitudinal Light Clock",
versus the more common "transverse light clock" found in many textbooks.
@Drew 's diagram is correct.
Although, in the answers by @Drew and @thermomagnetic condensed boson and others,
"length-contraction" is highlighted to account for time-dilation,
its probably be better to highlight the "invariance of the square-interval"
via the invariance of the area of the triangle (more generally, causal diamond).
This area invariance follows from the determinant of the Lorentz boost transformation being equal to 1. Additionally, the eigenvectors of the Lorentz boost preserve the edges to lie along the light cone.
Some details of this idea and some applications
have been worked out in my article
"Relativity on Rotated Graph Paper"
American Journal of Physics 84, 344 (2016)
https://doi.org/10.1119/1.4943251
(see also: https://arxiv.org/abs/1111.7254 )
(see also: https://www.physicsforums.com/insights/relativity-rotated-graph-paper/ )
The rotated graph paper makes it easier to draw the "ticks" of the light-clock
(called light-clock diamonds) and, more importantly, calculate graphically with them.
To address the comment to the OP by @thermomagnetic condensed boson
about visualizing the standard "transverse light-clock" on a spacetime diagram, one can view
one of the episodes of
Caltech's Mechanical Universe , episode 42 (at 24m10s):
https://www.youtube.com/watch?v=feBT0Anpg4A&t=24m10s
For a more complete visualization,
one can look at an older article of mine
"Visualizing proper-time in Special Relativity"
Physics Teacher (Indian Physical Society), v46 (4), pp. 132-143 (October-December 2004)
https://arxiv.org/abs/physics/0505134
For an animation with "circular light clocks" (which include the transverse and longitudinal light clocks),
https://www.youtube.com/watch?v=tIZeqRn7cmI
More animations are at http://visualrelativity.com/LIGHTCONE/LightClock/ .
In the textbook version it is easy to see how the path length increases in accordance with Pythagoras' theorem. With the light travelling parallel to the direction of motion, the pulse will travel further in order to get from $A$ to $B$ than from $B$ to $A$, but the total path length over a complete cycle remains the same regardless of the relative speed. So the clock runs at the same rate, whatever the relative speed. But hang on: the Fitzgerald-Lorenz contraction will shorten the distance between the mirrors by a factor of $\gamma$. So the clock will run faster with increasing relative speed.
