How are anti-unitary operators applied?

Question

I was reading about anti-unitary operators from Wikipedia. They give an example of an anti-unitary operator:

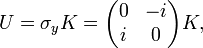
were $K$ is complex conjugate operation. $\sigma_y$ is defined with respect to two orthonormal vectors spanning a 2-D Hilbert space $H$ say $|0\rangle$ and $|1\rangle$. Now if I have an initial vector as $\alpha|0\rangle+\beta|1\rangle$ would the operator $K$ apply only on complex number $\alpha,\beta$ ( result being $\alpha^*|0\rangle+\beta^*|1\rangle$ ) or on the vector $|0\rangle$ and $|1\rangle$ aslo ? If it does what does it mean to take complex conjugate of $|0\rangle$ and $|1\rangle$ ? My doubt arises because anti-unitaries not being linear can't be expressed only in terms of matrices.

Answer 1

The antiunitary operator is an operator on the Hilbert space. Thus, it is nonsense to say that "the operator $K$ apply on complex number". Nevertheless, it can be shown that $U\alpha |\phi\rangle=\alpha^*U |\phi\rangle$, where $U$ is an antiunitary operator. $U|\phi\rangle$ should not be confused with $\langle\phi|$, the complex conjugate of $|\phi\rangle$.