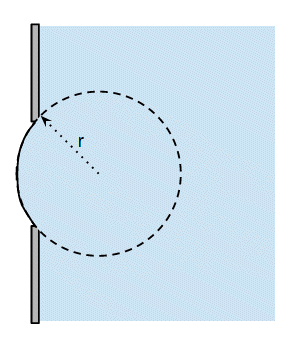
Assume I have a trough of water 5mm deep. If I cut a slot (oval) into that trough what is the maximum size/area (length x width) that slot can be without leaking?
This question is similar to Max. Radius of a hole 47021 but this is a slot.
From the equation provided in 47021, I don't believe it would be reasonable to calculate the area of the round hole and carry it over to my slot situation. As the slot could mathematically be very thin and very long.