[I have changed the title and also edited the question in other ways to make it clearer what I am asking. I hope it no more reads like a duplicate. Nor it remains any more a history question.]
I have gone through "Lectures on Physics" (Vol. 1) by R.P. Feynman and have been convinced that at first scientists were in search of a quantity which remains constant w.r.t. any other internal change,in a closed system.The quantity later turned out to be 'Force x displacement'.
But we know that momentum is also conserved in closed systems. So why don't we invent a scalar 'mass x speed' (in order to fix the problem that momentum is a vector) and use this instead of 'Energy'? While we could take the advantage that 'mass x speed' is much simpler than '1/2 (mass x square of velocity)'.
One of my teachers, on being asked this question, said that energy is more fundamental than momentum. And giving the example of a field force, he wrote-
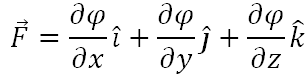
And showed that the quantity phi turns out to be the potential energy of a particle (e.g. a point mass in case of a gravitational field) in the field at the point (x,y,z). But what is the THOUGHT BEHIND the approach to find out such a quantity whose change w.r.t. position will describe the force? And at which point 'Force x displacement' becomes more fundamental than momentum?
