How is periodic potential approximation justified in semiconductor physics?

Question

In semiconductor physics the usual approximation is that of infinite crystal. This seems to allow one to write Hamiltonian for electron subsystem (in adiabatic approximation) as having periodic potential created by nuclei. But, as I've tried to plot how such a potential would look like, I was surprised to see that increasing the number of nuclei doesn't lead to convergence to a periodic potential. Instead it globally looks like a parabolic potential, with local features like Coulomb singularity. Here's a plot of 1D cross-section of 3D cubic nuclear lattice potential:

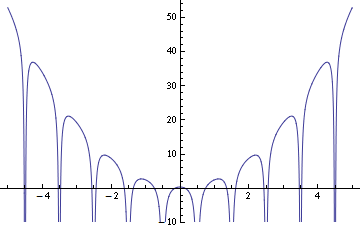
But in this case we can no longer say that the potential is actually even similar to a periodic one. This shouldn't actually surprise us, because in the limit of continuous nuclear charge the potential should become parabolic. So, how is periodicity approximation justified?

I can think of the following reasons:

• The combination of nuclear charge and lattice constant makes the parabola curvature small, so that the potential is approximately periodic across hundreds and more of lattice cells

• Or, if the above is wrong, then due to mean field approximation, we end up with an effective potential for a single effective electron, for which the whole crystal looks like a unit positive charge smeared across infinite number of nuclear sites, thus the curvature of parabolic potential appears infinitesimal, and we recover periodic potential

Which if these explanations is correct, if any? If none, then what is the correct justification?

Answer 1

Upon further consideration, I think that your second solution is probably closer to the truth. We are told that the potential is due to an array of ionic cores, not necessarily point charges. So there has to be some assumption of screening. Basically the offset of the overall potential amounts to the work function, and the periodicity of the potential is assumed, and then shown to be self consistent. This is especially important because we need to solve the electron wave function in order to find $V(x)$, a la Born-Oppenheimer.

In 3d, we should expect the unscreened potential to grow as $r^2$ around any point, as predicted by Gauss's law, and this is what you are finding. I think therefore screening is important, because the crystal is really neutral. The very crudest mean field approximation would be to subtract the potential that Gauss's law predict for a uniformly charged sphere around your origin.