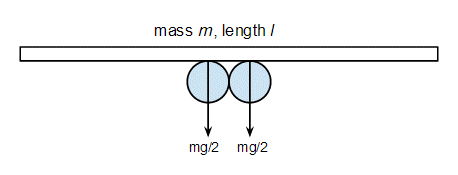Why do your fingers alternate then meet at the center of mass of the object?

Question

Hold a pen (or pencil, ruler etc.) using your two index fingers with your fingers at the ends of the object. Now move your two fingers toward each other. Assuming the frictional force provided by both fingers is identical, they should meet at the center of mass of the object, with your fingers alternating between being stationary and moving. When going backwards from the centre, one finger stays stationary the whole time. Why is this the case?

I have a general 'hand-wavey' explanation (the closer to the centre of mass a finger is, the greater the reaction force and thus the frictional force exerted, the finger with less friction moves until it is holding more weight and the frictional force becomes too large...) but I am looking for a much better detailed (mathematical if necessary) explanation including all the forces at play, torque, moments etc.

Answer 1

If we take a side view the initial state of your system looks like this:

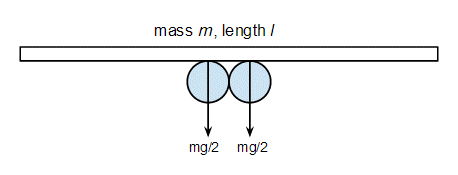
It should be obvious that the force at each finger/ruler contact is $mg/2$, and therefore the frictional force is:

$$ F = \tfrac{1}{2}mg\mu_s $$

where $\mu_s$ is the coefficient of static friction. Now you start to pull your fingers apart, and one of your fingers will slide first. Which finger will slide isn't predictable because it depends on minor details like how sweaty the two fingers are, whether you have minor muscle tremors, whether the fingers are at exactly the same angle and no doubt many other details. Let's assume the right finger slides first so the system now looks like this (I've exaggerated the amount of movement to make the diagram clearer):

I've labelled the finger that didn't slide $1$ and the finger that did slide $2$. The forces are no longer the same because the system is no longer symmetrical, though of course they must still add up to $mg$.

Now, the frictional force at finger $1$ is $F_1\mu_s$, and the frictional force at finger $2$ is $F_2\mu_d$, where $\mu_d$ is the dynamic friction. Note that dynamic friction is generally lower than static friction so $\mu_s > \mu_d$, though actually this won't make a difference to our conclusions. So we have three possibilities:

1. $F_1\mu_s > F_2\mu_d$ in which case finger $2$ carries on sliding

2. $F_1\mu_s < F_2\mu_d$ in which case finger $2$ stops sliding and finger $1$ starts sliding

3. $F_1\mu_s = F_2\mu_d$ in which case both fingers slide

It's pretty easy to show that case 1 applies. There are three forces acting, $F_1$, $F_2$ and the weight of the rod $mg$. We'll take moments about the centre of the rod, which means that the moment due to the weight of the rod is zero, and we get:

$$ F_1 d_1 = F_2 d_2 $$

or:

$$ F_1 = F_2 \frac{d_2}{d_1} $$

and because $d_2 > d_1$ the ratio $d_2/d_1 > 1$ and therefore $F_1 > F_2$. If we take our condition 1 above and rearrange it slightly to:

$$ F_1 > F_2\frac{\mu_d}{\mu_s} $$

We just proved that $F_1 > F_2$, and we know that $\mu_d/\mu_s < 1$ because dynamic friction is less than static friction, so we've proved that:

$$ F_1\mu_s > F_2\mu_d $$

and condition 1 applies.

Our conclusion is that as soon as a finger slips even a little bit the friction between that finger and the rod is reduced while the friction between the other finger and the rod is increased. So whichever finger slides first will carry on sliding.

Answer 2

We'll assume a simple cylindrical rod with the center of gravity halfway along the length. Due to the nature of the problem we're considering, all gravitational effects act as torque rather than linear acceleration, which is convenient for the analysis.

You start the exercise with one finger under each end of the rod. Examining only the left finger (or pivot, in the mechanical sense) you see an apparent clockwise torque about the finger due to the weight and length of the rod. Likewise examining only the right finger, there is a counterclockwise torque. Because the situation is symmetric, each torque is equal. This also means that each finger experiences an equal downward force, since you can consider the rod as a lever, with one finger acting as a pivot and the other acting as a stop, while gravity applies force to the lever - this situation is also symmetric, and the overall result is a net downward force centered in the middle of the rod. Because the net downward force due to gravity acts at the center of the rod, and the net upward force from your fingers also zero out in the center of the rod, there is no net torque on the rod and it continues to rest where it is. The downward force on each finger is also equal, and thus the friction force of each contact point is equal.

You now grasp the rod in your left hand while you move your right finger a quarter rod-length towards the middle. Now there is a lesser counterclockwise torque and a greater clockwise torque about the right pivot. Combined with the original maximum clockwise torque about the left pivot, the net downward force on the rod is closer to the right pivot than to the left - again acting on the center of the rod, or the rod's center of gravity.

Now, however, the net torque acting on the rod due to gravity is clockwise. Because the right finger is opposing the clockwise torque and the left finger is opposing the counterclockwise torque, there is now a higher force applied to the right finger and therefore a higher static frictional force. In the previous step you grasped the rod while you moved one pivot; here you will slide the left finger while the rod simply rests on the right. You apply force inward with both fingers, and because there is a lesser downward force on the left finger, it begins to slide while the right finger clings to the rod through fiction.

If you stop you left finger the same distance from the center as your right, you'll be back to a similar symmetric situation as where you started. However, as long as one finger is sliding, it will continue sliding past that equilibrium point, since the coefficient of dynamic friction is less than that of static friction. As you continue sliding the left dinner inwards, the net torque shifts toward counterclockwise until the dynamic friction on the left finger is greater than the static friction on the right finger. At that point the left finger stops sliding and the right finger starts: the process of sliding, torque rebalancing and frictional changes continues until both fingers eventually meet under the center of gravity.