Fundamentals of Light

Question

Is it possible to determine the number of cycles in a single photon? Do photons with higher frequencies have more cycles in each photon than those with lower frequencies? Would this mean that all photons have a uniform length, or would higher energy photons be shorter than lower frequencies to maintain the same number of cycles?

Answer 1

As I understand your question, it narrows down to "what is the length of a photon?", and thus how many "waves" are in each photon. As you comment yourself, this is not an appropriate way to think of a photon. The link you provide has an answer for a packet or pulse of several photons, which can have any length, given by the duration of the pulse. But a single photon does not really have any length, and should more appropriately be thought of as a single point propagating in space, with oscillating and mutually perpendicular electric and magnetic vectors, in turn perpendicular to the direction of propagation. The distance that this point travels between each time the vectors reach maximum (or any other point on the sine curve described by the vectors) is the wavelength. But the two wave crests do not exist simultaneously.

Googling "length of a photon" directed me to this discussion of the concept.

I made a beautiful drawing here that may help. Maybe not. If the wavelength is, say, λ = 6000 Å (orange), then it takes the photon λ/c = 2×10^-15 seconds to travel its own wavelength, but it exists at the point x₀ only at time t₀, and at the point x₁ only at a later time t₁.

Answer 2

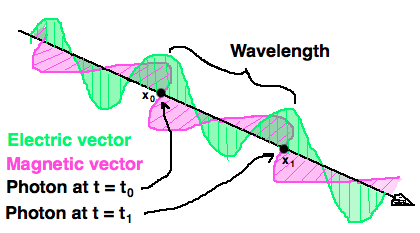
See the picture below. This is how an electromagnetic wave looks like, and a photon from the electromagnetic wave also looks like this.

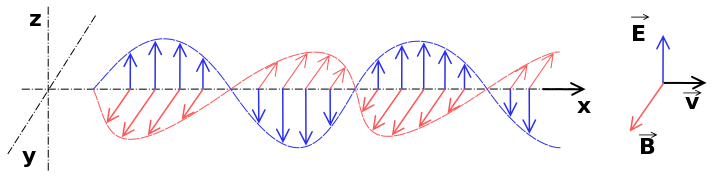
But in fact, the picture is not static, see in Wikipedia the moving electromagnetic wave.

You see there the picture moving. An observer at a point P in space will see the electric field increasing, decreasing, again increasing, and so on. This is a cycle. And the duration of a cycle is named period, $T$. Multiplying $T$ by the light velocity we get the wave-length $\lambda$.

On the other hand, we don't use to work with $T$ but with the frequency $\nu = 1/T$, which represents the number of cycles per second.

Now, gain to your photon. Its properties are like those of the beam in which it is found. If you consider, for instance, a beam of visible light, red, or green, so looks like a single photon from it.

About the number of cycles in a photon, it is given by the duration of the wave-packet to which belongs the photon. If we emit a signal of V (ultra-violet) light for 2$\mu$sec., and the UV frequency is 5x$10^16$Hz, then a photon from this signal lasts for $10^11$ cycles. The wavelength is given by the formula $\lambda = c/ \nu$ so, in our case $\lambda$ = 6nm, s.t. the length of the photon is 600m.

Answer 3

The photon is an elementary particle in the standard model of particle physics, so it is a point particle. It is described by a wavefunction which is the solution of quantized Maxwell's equation. The wavefunction controls the probability of the location of a photon, and it does have information on the electromagnetic potential in the equations. The frequency just give information about its energy from E=h*nu.

When in synergy with a large number of photons, the probabilities in space build up the electromagnetic field of the classical electromagnetic wave. The classical wave has the frequency identified in the individual photons as energy.

Here is a double slit experiment that breaks down to individual photons.

The single particle events pile up to yield the familiar smooth diffraction pattern of light waves as more and more frames are superposed (Recording by A. Weis, University of Fribourg).

Each photon is a point on the screen, no spatial spread. As they are accumulated the classical interference pattern of a beam with the frequency of the laser appears.

So, a photon does not have a cycle. An aggregate of photons does have cycles in space characterized by the wavelength. The same holds with variations in time, except it is not as simple an experiment for demonstrations.

Answer 4

As answered by @anna v, quantum nature of light tells you that you cannot "count the cycles". I would just like to present this from another angle... the photon itself does have a wavefunction that of course has some shape that you want to detect (some kind of wavepacket probably, but could be more complicated, it could even be entangled with another photon). The problem here is that you cannot measure the wavefunction. For one photon, you can only measure one sample value of a chosen operator (be it instantaneous E field amplitude, frequency, phase, polarization...). When you measure the value, the wavefunction collapses to that value, so you cannot re-measure it to get the other necessary values. So you cannot record the wavefunction shape, once you record the first point on the graph, you ruined the photon. You cannot even measure an expectation value (average) on a single photon, it only makes sense if you obtain enough samples and a single photon only gives you one sample.

A very specific consequence is the well known uncertainty principle. If you measured the frequency, you lost the ability of accurately measuring the time of arrival and vice-versa.

As a result, you can only observe a wave, if there are enough photons to sample the shape of the wave. A single photon has a wavefunction but recording it would be like quantum cloning, which we know isn't possible (recording the wavefunction would allow you to duplicate it).

what you measure depends on your apparatus. It is possible to measure nonlocal properties (for instance, you can have a circuit that only fires if the wavefunction has a peak at two spatially separated receptors). Measuring discrete location is only a very special case of measurement (a delta function operator, if you want).