I think this is what Callen has in mind: he takes one finite body isolated from everything else and assumes that it is separated by internal constraints so that the several homogeneous parts that maintained by said constraints are in equilibrium within itself and with each other. Next he removes some constraints and in his view the 2nd law is that after equilibration the new entropy is the maximum among all other possibilities conformant with these new set of constraints in effect. Formally, for a differentiable entropy function, the maximum entropy principle means that in equilibrium $\delta S=0$ and $\delta ^2 S < 0$ where $\delta S$ is a virtual variation of the entropy.
Since in a reversible process the entropy does not change and each step is an equilibrium you are rightly asking "How can a system go to another state with the same entropy? This seems to be a contradiction. When he says that the entropy must be monotonically nondecreasing, I think in view of the postulate it should be monotonically increasing."
The real answer is that there is no reversible path, it only exists as the mathematical limit of all those quasi-static paths whose entropy change at every step approaches zero and whose second derivative is negative in the limit.
In practice, the way this is achieved is by connecting a single body (a system) to reservoirs of much larger size, so large compared to the "system" that all irreversible entropy production within them is negligible and that their individual intensives do not change relative to that of the system. Ideally, they are of infinite size.
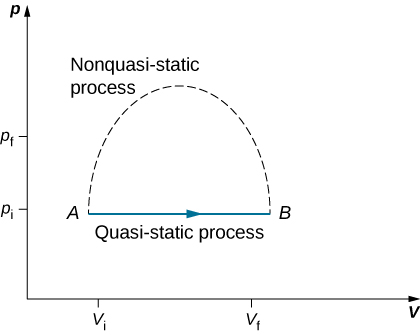
Say, we have a diathermal cylinder with fluid inside and connect it to a pressure and a thermal reservoir that are so large that whatever happens to the cylinder it will affect neither pressure or temperature of the respective reservoir. The reservoirs are thus in both mechanical and thermal equilibrium, resp., with the gas and its cylinder that is equipped with a movable piston with small marbles on it. Now to approximate a reversible process we add/remove very small masses to/off the piston, if these are small enough then nearly every step is an equilibrium with the reservoirs and total entropy change is a small positive quantity.
We cannot claim that the total entropy is ever maximum when all the entropy comprising the ideal reservoirs and the system added up because the entropy of an infinite thermal reservoir is infinite. But we can still claim that the entropy change is zero or almost zero because we can measure the entropy transported at the interface between the system and thermal reservoir, and we can also measure the entropy change within the system. If we do this quasi-statically with smaller and smaller steps we can achieve smaller deviations from equilibrium. This example is an approximation of an isothermal and reversible process. But smaller the "disequilibrating" steps, the longer it will take to go from one equilibrium state to another.
Instead equipping the system with external reservoirs, we can also approximate a quasi-static process by stepping through small enough changes within the internal constraints, and after every new setting we wait until equilibrium and start anew.
In either case, Callen assumes that in the limit such disequilibrating small steps will lead to sufficiently near a single equilibrium state.
He assumes so but it is not proven anywhere that such procedure really converges to a reversible process. At this level of abstraction it is a mathematical problem and it cannot be just dismissed because each and every tiny irreversibly step being finite, the corresponding internally generated entropy must be positive even if it is arbitrarily small. But the smaller the steps, the larger their number is and their sum may not ever converge to zero. I have never seen anywhere this issue even brought, never mind even analyzed, it is just assumed to be true.
On the other hand, we do know that all processes are irreversible, that there are no adiabatic walls, no true isolated bodies, etc., and we can only approximate those. Approximating one irreversible process $\mathcal P_1$ with another irreversible process $\mathcal P_0$ we can measure the differences between the two from the start to finish including its entropy change.
If we say the body is almost isolated, and its entropy also barely changes during, say, $\mathcal P_0$, we can say that it represents a nearly reversible process that could be approximated by processes that have successively smaller and smaller entropy changes relative to it and that in the limit approaches that of $\mathcal P_0$. For most bodies I think this assumption holds empirically. I say most, because I suspect that it will fail to hold for a ferromagnet or for a plastic body going through a hysteretic cycle.